Warning: Your questions require answers using quantum field theory. Thus, in some cases in the following I refer to the functional integral and some of its properties. In the situation of interest for your question, these concepts may be made precise, though I do not do this here.
1) You should think of the hemisphere that they are considering a two-dimensional manifold with a metric. In general in a quantum field theory on a manifold $M,$ a metric is required to formulate the action functional. For instance the "kinetic terms" for a scalar field $\phi$ typically take the form $\int_{M} \phi \Delta \phi$ with $\Delta$ given by the usual Laplacian.
In a topological field theory, like the ones they are considering, it may turn out that certain quantities do not depend on the choice of the metric, or more generally depend only on an equivalence class of the metric under some notion of equivalence (like conformal equivalence). However, regardless one still picks a metric to formulate an action and a functional integral.
2) The twisted and untwisted theories are equivalent on any space, like the cylinder, which is metrically flat.
Starting from the ordinary field theory, we obtain the theory on a Riemannian manifold $M$ by replacing partial derivatives by covariant derivatives, promoting the Lebesgue measure to the volume form, and all other natural things associated to making the action well-defined on a general manifold.
The theory has a global $R$-symmetry, meaning that all fields also transform in representations of some group Lie Group $G_{R}$, and hence may be viewed as sections of vector bundles. $G_{R}$ also has the important property that enters the supersymmetry algebra non-trivially, with the supercharges also in representations of $G_{R}$.
To obtain the topologically twisted version, we now do an additional step utilizing $G_{R}$. We activate a connection (called an $R$ gauge field) for $G_{R}$ and covariantize derivatives with respect to this connection as well.
The value of this connection is fixed to be related to the value of the spin connection on $M$. More explicitly, the twisting construction requires a choice of Lie algebra homomorphism $\chi$ from the local holonomy algebra of $M$ (ie $so(d+1)$ if $M$ has dimension $d+1$) to the Lie algebra of the $R$-symmetry group. Then we set
$\chi$(spin connection)=$R$ gauge field.
If $M$ is flat, then the spin connection on $M$ is trivial so the second step does nothing, and the twisted theory on $M$ is the same as the ordinary untwisted theory on $M$.
3) The construction that they are doing is a special case of the state operator map in conformal field theory. In general given a conformal field theory in dimension $d+1$ it assigns a Hilbert space to any manifold of dimension $d$.
An important special case is the that of the sphere $S^{d}$. Given a formulation of the CFT in question in terms of fields, we consider all values of the fields on $S^{d}$ (satisfying some $L^{2}$ conditions which I suppress). We form a vector space $V$ whose basis elements are in one-to-one correspondence with these boundary conditions. An element of the Hilbert space associated to $S^{d}$ is a linear functional on these boundary conditions, ie an element of the dual space $Hom(V,\mathbb{C})$ (again satisfying certain normalizability conditions which I suppress).
There is a bijection between the set states on $S^{d}$ and the local operators $\mathcal{O}(p)$ at any fixed point $p.$
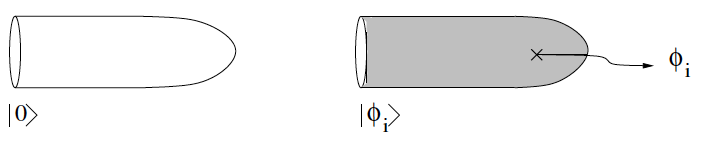
To set up this isomorphism, one considers a functional integral on the $d+1$ dimensional ball with boundary $S^{d}$. To carry out the path integral one must specify boundary conditions for fields. The result of the functional integral produces a complex number, which depends upon these boundary conditions, and hence via the definition above results in a state. We refer to this state a $\Psi_{1}$, it is the state corresponding to the unit operator in the claimed bijection.
More, generally, for each local operator $\mathcal{O}(p)$ we obtain a state $\Psi_{\mathcal{O}}$ by considering the functional integral on the ball but now with the local operator $\mathcal{O}$ inserted at the center of the ball. This defines a map from local operators to states on $S^{d}$. If the theory is conformally invariant, then an inverse may be constructed by shrinking the $S^{d}$ to a point and constructing a local operator from any state.
(If you are new to CFT, I highly recommend that you work out the state operator map in detail for the case of a free scalar. The answer can be found in Polchinski's string theory book in chapter 2.)
Finally, let us return to the construction in question. We consider the path integral on a very long cigar. We view this as a flat cylinder of fixed circumference and length $L$, glued to a round cap where the cap has a fixed metric. We are interested in what happens as the length $L$ of the cylinder tends to infinity.
We perform the path integral in two steps.
First, by integrating over fields in the cap together with a local chiral operator $\mathcal{O}$ inserted at the tip of the cap, we obtain a state $\Psi_{\mathcal{O}}$ as above.
Next, we do the path integral over fields on the long cylinder. Although we could do this explicitly, it is simpler to instead note that the path integral on $S^{1}\times I$ computes the evolution operator $e^{-H L}$ where $H$ is the Hamiltonian of time evolution for states on $S^{1}$, and $L$ is the length of the interval $I$. Thus, the complete result of the path integral on the long cigar together with the insertion of the local operator is the state in the Hilbert space
$e^{-HL} \Psi_{\mathcal{O}}$
Now we let $L$ tend to infinity. The operator $H$ is positive definite and bounded below by zero. Hence, as $L$ tends to infinity, $e^{-H L}$ is a projection operator onto the subspace of the Hilbert space with $H=0$. This is exactly the subspace of vacuum states.