Consider a big finite rescaled piece of $\mathbb{Z}^2$, i.e. consider a unit square with a thick grid. Famous Wilson's method allows to generate a colored spanning tree of such a graph in a uniform way by popping out the cycles: if the mesh-size tends to 0, then the interesting fractal structure occurs: one can read about this in R.Lyons, Y. Peres Probability on Trees and Networks. It is said there, that this fractal structure is not yet explained. Could someone point to me some works connected to this question, if there are any?
$\begingroup$
$\endgroup$
2
-
$\begingroup$ Have you tried contacting Lyons and/or Peres? $\endgroup$– Gerry MyersonCommented Jul 21, 2013 at 23:38
-
$\begingroup$ Not yet, I first though about asking in the club.) $\endgroup$– OlgaCommented Jul 22, 2013 at 6:52
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
Is this the fractal structure you have in mind?
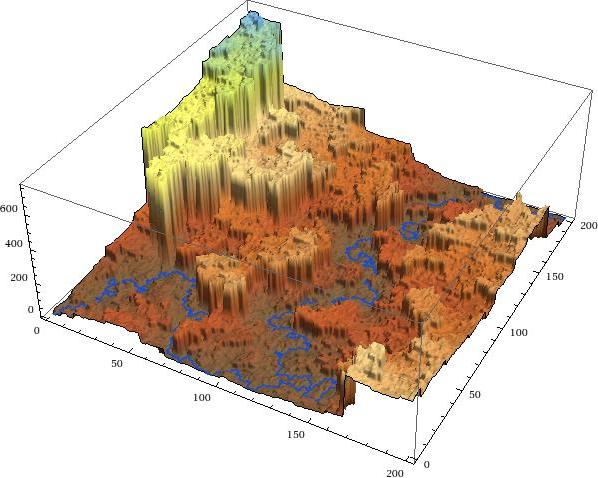
Image from Russell Lyons' book page. Figure 4.6 in the book. Caption:
"The distances in the tree to the path between opposite
corners in a uniform spanning tree in a 200x200 grid."
answered Jul 21, 2013 at 23:33
-
3$\begingroup$ This fractal can also be built in SimCity 2K. $\endgroup$ Commented Jul 21, 2013 at 23:38
-
-
1$\begingroup$ This is a 3D-picture of distances but already a 2D picture (it's projection) is interesting: the colors are the colors of the cycles that are popped in Wilson's method. I have in mind a picture from the same book, one page before. $\endgroup$– OlgaCommented Jul 22, 2013 at 6:49