9
$\begingroup$

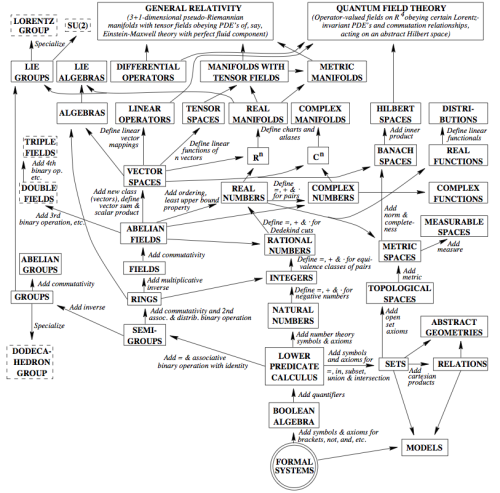
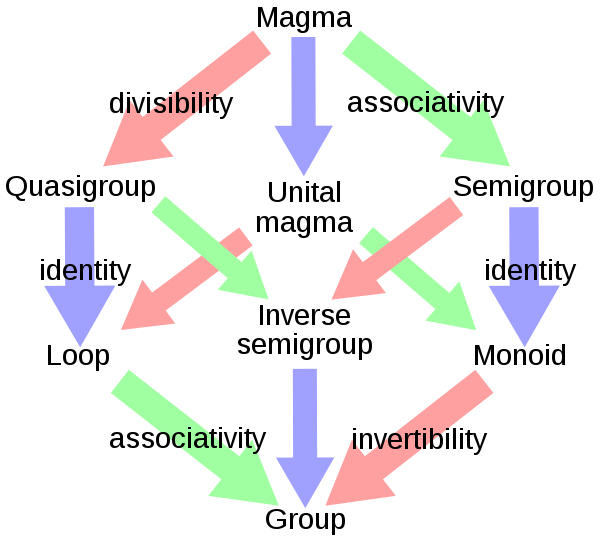
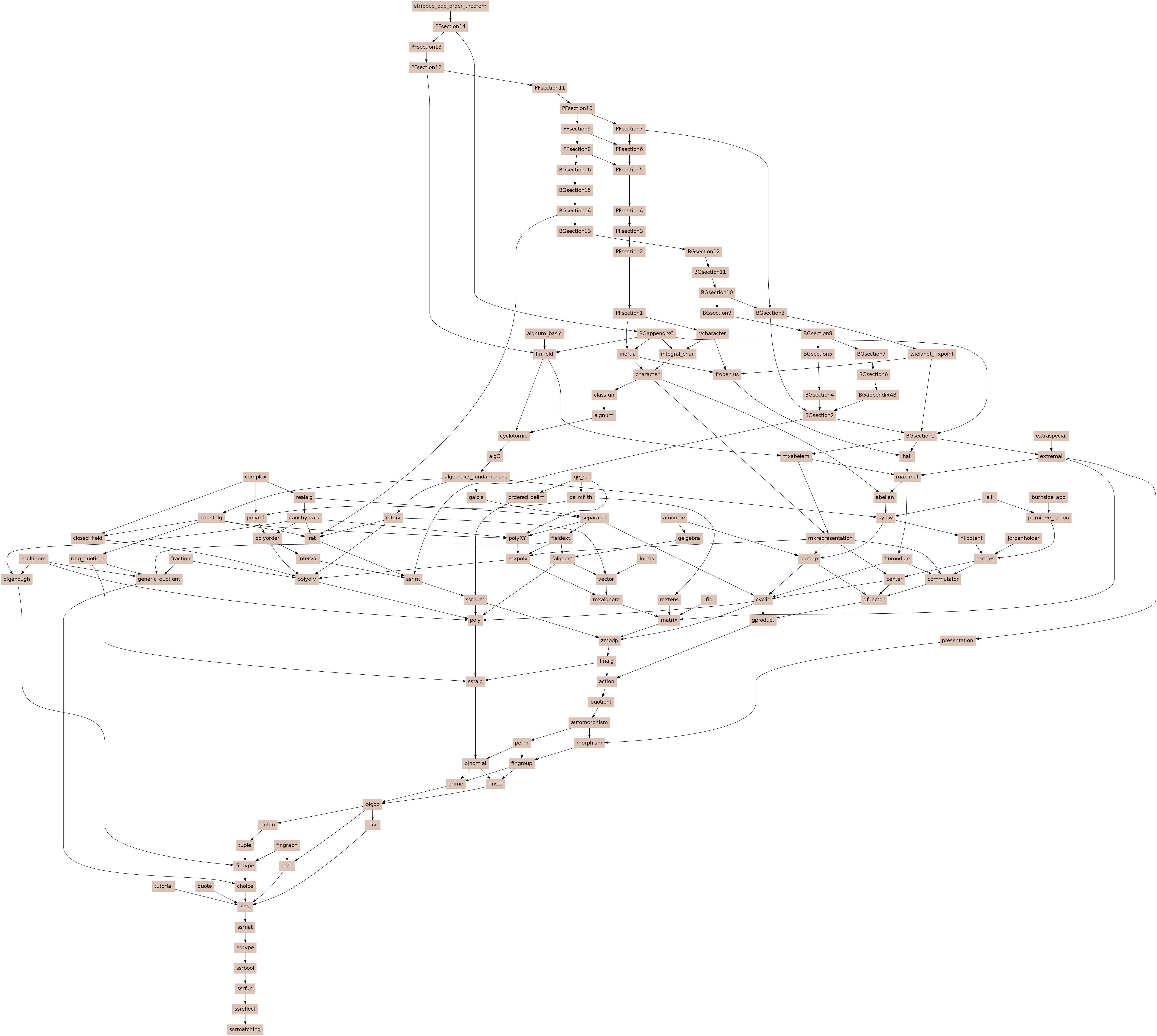Hello! Does anybody know an image of a graph featuring the hierarchy of algebraic structures? Something rather complete.

So far I've found similar images describing the hierarchies of classes/categories in various programming languages. For example

Improve this question
$\endgroup$
1
  • 4
    $\begingroup$ "Something rather complete" sounds like too tall an order. There must be thousands of algebraic structures that mathematicians are interested in. $\endgroup$
    – Todd Trimble
    Commented Aug 14, 2011 at 13:28

3 Answers 3

Reset to default
10
$\begingroup$

Page 2 of "Is 'the theory of everything' merely the ultimate ensemble theory?" by Max Tegmark contains the following image:

Relationships between various basic mathematical structures

Improve this answer
$\endgroup$
3
  • 13
    $\begingroup$ What on earth are double fields and triple fields??? Also, why does the dodecahedron group belong to that chart? ...strange choices. $\endgroup$
    – André Henriques
    Commented Aug 15, 2011 at 14:29
  • 4
    $\begingroup$ +1 to André's comment. However much I respect physics and physicists, I personally think the article in question is a truly bizarre choice of a reference when it comes to dealing with algebraic structures. $\endgroup$
    – Vladimir Dotsenko
    Commented May 14, 2012 at 8:41
  • 2
    $\begingroup$ There are some pieces one might consider useful, but a lot of it inspires a resounding "LOLWUT" $\endgroup$
    – rschwieb
    Commented Jul 14, 2015 at 2:54
7
$\begingroup$

This is sort of a delayed response, but I came upon this question while googling for the visualization of the hierarchy of structures used in the Coq proof of the Feit-Thompson Odd Order theorem. That visualization is located here, image copied below:enter image description here

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Do you have a link to a higher res version. The link you gave is a directory. $\endgroup$
    – Christopher King
    Commented Oct 29, 2016 at 13:49
1
$\begingroup$

You can complement the image from Mark Kim with the algebraic structures between magmas and groups:

enter image description here

Source: https://en.wikipedia.org/wiki/Semigroup

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .