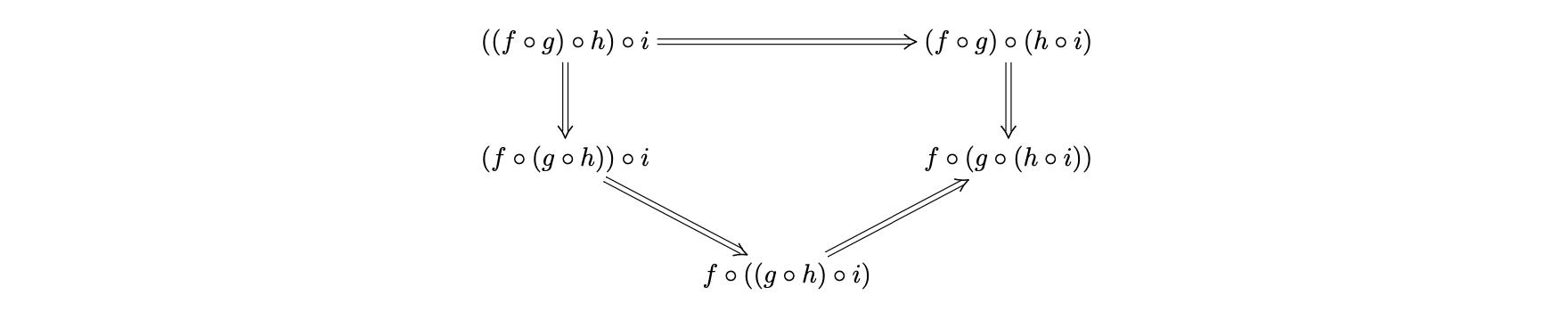
Assume we have a good working knowledge of $n$-dimensional category theory for some fixed $n$. It seems like it should be possible to 'predict' what coherence diagrams we're going to encounter in the next dimension from the current one, seeing as these coherence diagrams are essentially a byproduct of the differing ways we can combine the data we have in the current dimension. For example, the pentagon identity in dimension $2$ is a manifestation of the differing orders in which we can compose $4$ arrows in a $1$-dimensional category:
Similarly, the interchange law we encounter in dimension $3$ is a byproduct of the differing ways we can combine data in dimension $2$. My question is:
Has any work been done attempting to 'predict' the coherence diagrams in the next dimension from the one we're currently comfortable in?
I suspect this would be very difficult in full generality from personal coherence experience, old MO questions and comments like the one found in the introduction to Lukas Buhne's 2015 thesis 'Topics in Three Dimensional Descent Theory':
"This is because the definition of weak higher categories involves categorification, the process where axioms and more generally equations are replaced by new coherence isomorphisms subject to new axioms. It is a highly nontrivial task to identify which equations have to be replaced, and what kind of and how many axioms have to be enforced in order to allow for strictification."
This is related to an old unanswered question of mine; any tips or pointers are appreciated.