Bounding $\|X_1/(X_1+X_2) - Y_1/(Y_1+Y_2)\|_p$ by the closeness of $X$ and $Y$

Question

This question is inspired by the answer to this other question, but I have tried to make it self-contained and to zoom in on the counter-example from this answer.

Suppose $\{(X_n, Y_n)\}_{n=1}^2$ are two i.i.d. joint probability distributions (that is, $X_n$ and $Y_n$ are not i.i.d., but $(X_1, Y_1)$ is i.i.d. with regards to $(X_2, Y_2)$), with $0 < X_n, Y_n \le 1$. I am interested in the quantity $$ \left\|\frac{X_1}{X_1 + X_2} - \frac{Y_1}{Y_1 + Y_2}\right\|_p. $$ My intuition is that, as $X_n$ and $Y_n$ "get really close together" (i.e., $\|X - Y\|_p$ becomes smaller), this quantity should be upper-bounded by $C\|X-Y\|_p$ for some $C$ that depends on $X$. The previous question I asked on this forum, and which I referenced earlier, was concerned with a more general version of this quantity, but I studied this question's version in detail when trying to prove the more general one. I was unable to prove such a bound.

The reason was that such a bound does not exist; the answer I mentioned before gives a clever explanation of this based on a counter-example where $X_1,X_2$ are independent random variables each uniformly distributed on the interval $(0,1)$; $Y_1=h+(1-h)X_1$ and $Y_2=h+(1-h)X_2$, where $h\in(0,1)$. However, this reply made me wonder whether such a bound did exist for a lower power: can one prove that $$ \left\|\frac{X_1}{X_1 + X_2} - \frac{Y_1}{Y_1 + Y_2}\right\|_p \le C\|X-Y\|_p^\alpha \qquad \text{for some $0<\alpha<1$} $$ with $C$ a constant that depends on $X$? I investigated this question some more, but have not yet found any bound I can prove. I do have the following considerations, which seemingly conflict:

• In the counter-example, I do not see why the fraction $\frac1h$ could not be replaced by $\frac1{h^\alpha}$ with $0<\alpha<1$, which would invalidate the possibility of finding a bound such as the one I am looking for. I might, however, have missed a subtlety in the counter-example. EDIT: I now see why one cannot use $\frac1{h^\alpha}$ in that counter-example; disregard this bullet.
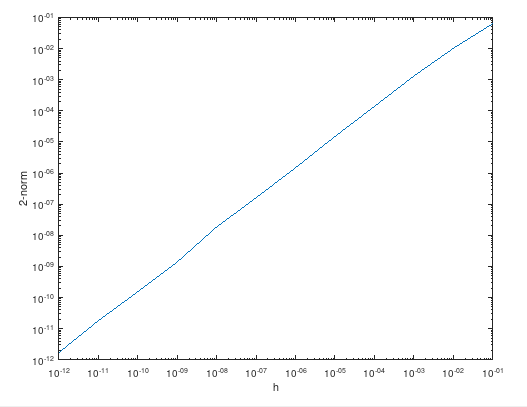
• On the other hand, I did some numerical tests that seemed to show very clean $\sim h$ (and thus $\sim \|X-Y\|_p$) convergence of the quantity of interest. I looked at its $(p=2)$-norm, which I estimated by using $10^6$ samples. The plot below suggests a very clean convergence to 0 at a rate $\sim h$.

I would be appreciative of any ideas as to whether this bound using $\|X-Y\|_p^\alpha$ can be attained. If no, how else could I quantify mathematically the intuitive and numerical fact that my quantity goes to zero as $\|X-Y\|_p$ does so?

Thank you for reading through the post! I look forward to hearing your ideas, especially given the detailed and helpful answer I received in response to my last question.

Answer 1

Such a bound does not exist.

This is shown by the following modification of the previous example:

Suppose that $X_1,X_2$ are independent random variables each with the pdf given by the formula \begin{equation*} f(x)=\frac c{x\ln^2 x}\,1(0<x<1/2) \tag{1}\label{1} \end{equation*} for $c:=1/\int_0^{1/2}\frac{dx}{x\ln^2 x}$; $Y_1=h+(1-h)X_1$ and $Y_2=h+(1-h)X_2$, where $h\in(0,1/2)$.

Then $0\le Y_1-X_1\le h$ and hence \begin{equation*} \|X_1-Y_1\|_r\le h \end{equation*} for all real $r>0$.

On the other hand, writing $A\gg B$ if $B=O(A)$, we get \begin{equation*} \Big|\frac{X_1}{X_1+X_2}-\frac{Y_1}{Y_1+Y_2}\Big| =\frac{h|X_1-X_2|}{(X_1+X_2)(2h+(1-h)(X_1+X_2))} \gg1 \end{equation*} on the event $\{X_2\le X_1/2\le h/2\}$. So, \begin{equation*} \Big\|\frac{X_1}{X_1+X_2}-\frac{Y_1}{Y_1+Y_2}\Big\|_p^p \gg\int_0^h dx_1\,f(x_1) \int_0^{x_1/2} dx_2\,f(x_2)\asymp\frac1{\ln^2 h}. \end{equation*} So, letting $h\downarrow0$, we see that the inequality \begin{equation*} \Big\|\frac{X_1}{X_1+X_2}-\frac{Y_1}{Y_1+Y_2}\Big\|_p\le C\|X_1-Y_1\|_r^\alpha \end{equation*} cannot hold for any real $C$ (even if $C$ depends on the distribution of $X_1$), any real $p>0$, any real $r>0$, any real $\alpha>0$, and all $h\in(0,1/2)$. $\quad\Box$

---

The OP asked in a comment if the answer would change if it is additionally assumed that $X_1=\exp(-U)$ and $Y_1=\exp(-V)$ for random variables $U\ge0$ and $V\ge0$ of which all finite moments exist.

Then the answer still remains negative, with \eqref{1} replaced by \begin{equation*} f(x)=\frac{1}{2 x}\,\exp\Big(-\sqrt{\ln\frac1x}\,\Big)\,1(0<x<1), \end{equation*} keeping essentially the same reasoning.