I am specifically referencing the property that, given a braided monoidal category with a braiding $c$ and left and right unitors $\lambda, \rho$, $$ \lambda_A \circ c_{A,I}=\rho_{A}, $$ for any object $A$. This equation is stated in almost every reference (first done so by Joyal and Street) defining a braided category, yet an explicit or reproducible proof is, to my knowledge, not given anywhere. The closest thing to a proof I can find is a series of hints on Mathematics SE asked in 2014, however this answer (by Turion) has several inconsistencies/typos, making it hard to understand. Is there some reference where this proof is actually 'spelled out'? How can one prove this relation given the braided monoidal category axioms?
-
3$\begingroup$ My guess is that this can be reconstructed from the version for a symmetric monoidal category, which is identity (8) in Kelly's On MacLane's conditions for coherence of natural associativities, commutativities, etc. doi.org/10.1016/0021-8693(64)90018-3 This is not an explicit proof, but at least it's published somewhere $\endgroup$– David Roberts ♦Commented Oct 20, 2021 at 3:19
-
2$\begingroup$ A completely spelled out diagrammatic proof is given in Proposition 1.3.21 of Volume II of Bimonoidal Categories, $E_n$-Monoidal Categories, and Algebraic $K$-Theory. $\endgroup$– EmilyCommented Oct 20, 2021 at 19:32
-
2$\begingroup$ @Emily: Why not make that comment an answer? It seems a full and excellent answer, publicising a really useful book! $\endgroup$– Peter LeFanu LumsdaineCommented Oct 23, 2021 at 7:01
-
1$\begingroup$ @PeterLeFanuLumsdaine Thanks! This is definitely a good idea; I hadn't thought about it as a chance to publicise Niles and Donald's really nice book(s)! $\endgroup$– EmilyCommented Oct 24, 2021 at 5:27
3 Answers
Using the notation of Joyal and Street §2, here’s a proof of $\newcommand{\r}{\rho}\newcommand{\l}{\lambda}\newcommand{\x}{\otimes}\newcommand{\comp}{\!\!\cdot\!}\r_A = \l_A \comp c_{A,I}$. Since $\l$ and $c$ are invertible, it suffices to prove $\r_A \comp (\l_A \comp c_{A,I} \x I) = \l_A \comp c_{A,I} \comp(\l_A \comp c_{A,I} \x I)$. Notation: I will write composition as $\comp$, and it binds tighter than $\x$; and will write all associators just as $\alpha$, to reduce clutter. I’ll black-box details of steps that are purely about symm. mon. cats., not involving the braiding — these are most easily seen using coherence for SMC’s.
$$\begin{align} \r_A \comp (\l_A \comp c_{A,I} \x I) &= (\l_A \comp c_{A,I}) \comp \r_{A \x I} & \text{naturality of $\r$} \\ &= \l_A \comp c_{A,I} \comp (A \x \r_I) \comp \alpha & \text{SMC facts}\\ &= \l_A \comp (\r_I \x A) \comp c_{A,I \x I} \comp \alpha & \text{naturality of $c$}\\ &= \l_A \comp (\r_I \x A) \comp \alpha \comp (I \x c_{A,I}) \comp \alpha \comp (c_{A,I} \x I) & \text{axiom (B1)} \\ &= \l_A \comp (I \x \l_A) \comp (I \x c_{A,I}) \comp \alpha \comp (c_{A,I} \x I) & \text{SMC facts}\\ &= \l_A \comp (I \x \l_A \comp c_{A,I}) \comp \alpha \comp (c_{A,I} \x I) & \text{functoriality of $\x$}\\ &= (\l_A \comp c_{A,I}) \comp \l_{A \x I} \comp \alpha \comp (c_{A,I} \x I) & \text{naturality of $\l$}\\ &= (\lambda_A \comp c_{A,I}) \comp (\lambda_A \x I) \comp (c_{A,I} \x I) & \text{SMC facts}\\ &= (\lambda_A \comp c_{A,I}) \comp (\lambda_A \comp c_{A,I} \x I) & \text{functoriality of $\x$} \end{align} $$
I found this proof using string diagrams, writing down (B1) for $A,I,I$ (as Joyal and Street suggest), and then contemplating how to connect that to the desired equation.
A completely spelled out diagrammatic proof is given in Proposition 1.3.21 of Volume II of Bimonoidal Categories, $E_n$-Monoidal Categories, and Algebraic K-Theory by Niles Johnson and Donald Yau.
-
2$\begingroup$ Incidentally, their other books are also very nice and extremely useful references; see Colored Operads, Homotopical Quantum Field Theory, Involutive Category Theory, 2-Dimensional Categories (with Niles Johnson), and Infinity Operads and Monoidal Categories with Group Equivariance. $\endgroup$– EmilyCommented Oct 24, 2021 at 5:28
-
$\begingroup$ After reading more of it, this text is a great resource aside from just this proof, thanks! $\endgroup$– naahivCommented Oct 24, 2021 at 13:10
-
1$\begingroup$ @naahiv It's my pleasure! I *really* like these three (and their other) books too :) $\endgroup$– EmilyCommented Oct 25, 2021 at 4:16
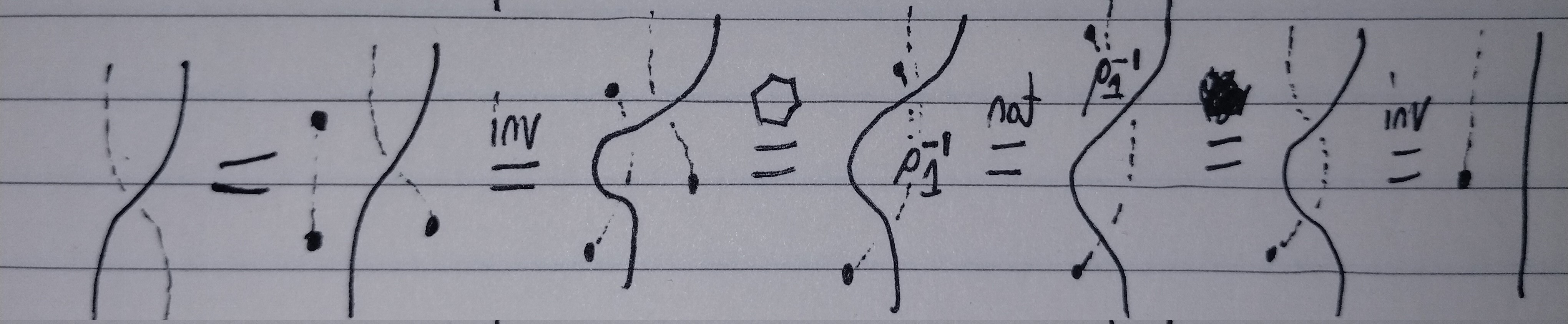
Here is a proof in string diagrams inspired by the proof in Johnson and Yau linked to in Emily's answer.
Read this diagram bottom to top; solid line represents A and dashed lines represent I. Coherence for the underlying monoidal category is assumed. The steps are labeled by 'inv' for invertibility of the braiding, 'nat' for naturality of the braiding, and a hexagon symbol for a hexagon identity.
Update - there is an error in the first diagram, the dashed line should start in a bold dot to represent the unitor - sorry.