Connective constant of a hexagonal lattice

Question

The connective constant of the honeycomb lattice equals $\sqrt{2 + \sqrt{2}}$ by Hugo Duminil-Copin and Stanislav Smirnov (arXiv:1007.0575) is the research paper I have read. My only doubt is equation 6, at page no. 7. How do we explicitly write that $$A_{T+1}^{x_{c}}-A_{T}^{x_{c}} \leq x_{c} (B_{T+1}^{x_{c}})^{2} ?$$

Answer 1

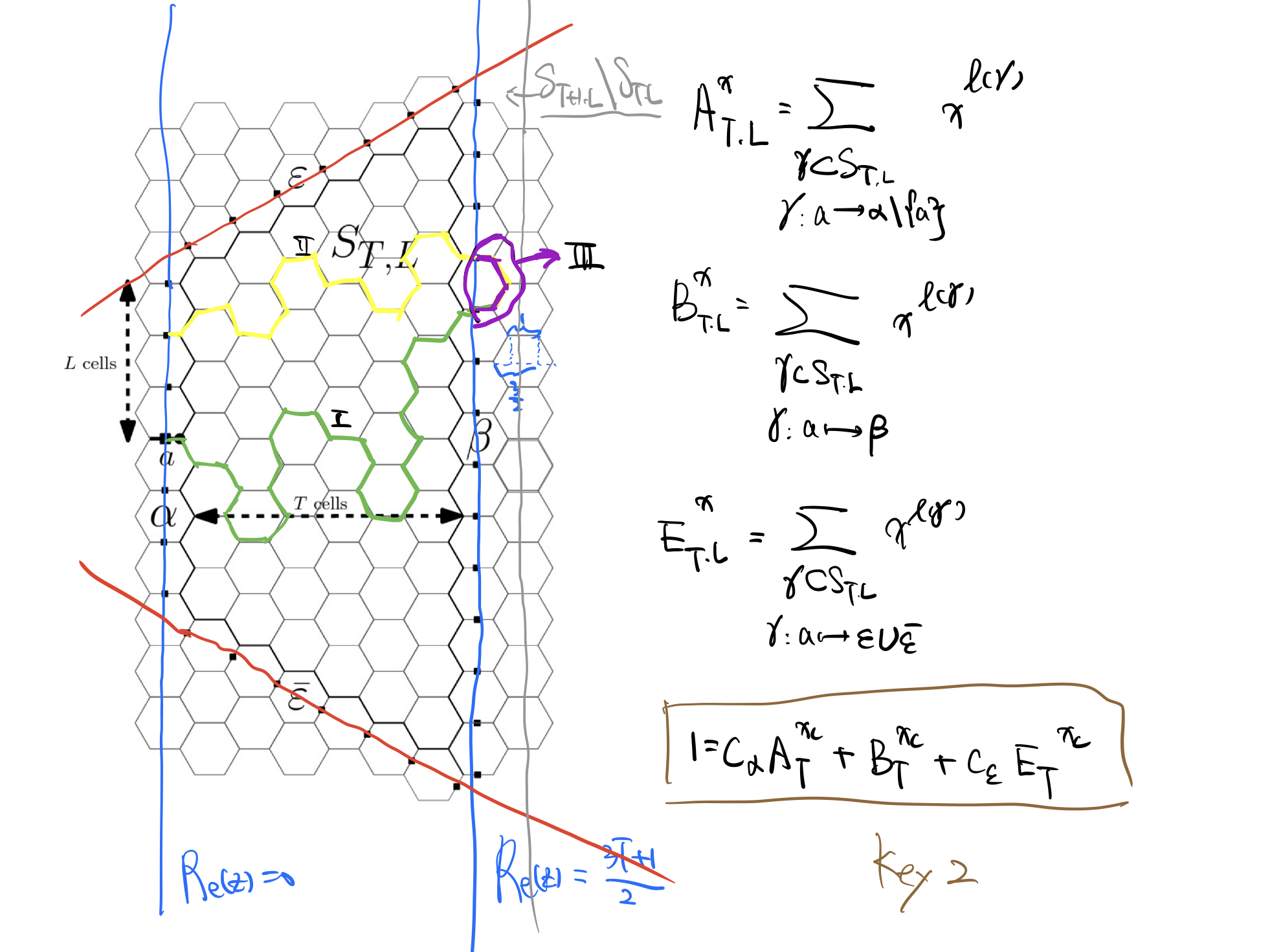Maybe this picture help to explain the argument, to prove $A_{T+1}^{x_{c}}-A_{T}^{x_{c}} \leq x_{c}\left(B_{T+1}^{x_{c}}\right)^{2}$, we try to estimate, $A_{T+1}^{x_{c}}-A_{T}^{x_{c}}$, just take one self-avoiding walk in the set, and split it into 3 parts, colored in yellow, green and purple in the graph, and the yellow part and green part can be bounded by $B^{x_c}_{T}$ and by translation invariant of $Z(x)=\sum_{\gamma: a \rightarrow H} x^{\ell(\gamma)}$ and purple part can be bounded by $x_{c}$ because every self-avoiding walk in the set at least intersection with the domain $S_{1+1, L} \backslash S_{T, L}$ with one piece.

For the yellow part and green part, we can bound $B_{T}\geq C_{x_c} B_{T+1}$ by directly expanding the self-adjoint walk sum, so finally we get $$ A_{T+1}^{x_{c}}-A_{T}^{x_{c}} \leq C_{x_c}x_{c}\left(B_{T+1}^{x_{c}}\right)^{2} $$ and $C_{x_c}$ is a constant unrelated with $T$, this is enough to do the same argument in the paper, the key point is the harmonic series is diverges, so $C_{x_c}$ is harmless.

---

I am curious whether the more concrete conjecture mentioned in this paper has been solved (or has some progress) by follow-up works,

conjecture Nienhuis proposed a more precise asymptotical behavior for the number of self-avoiding walks: $$ c_{n} \sim A n^{\gamma-1} \sqrt{2+\sqrt{2}}^{n} $$ with $\gamma=43 / 32$. Here the symbol $\sim$ means that the ratio of two sides is of the order $n^{o(1)}$ or perhaps even tends to a constant. Moreover, Nienhuis gave arguments in support of Flory's prediction that the mean-square displacement $\left\langle|\gamma(n)|^{2}\right\rangle$ satisfies $$ \left\langle|\gamma(n)|^{2}\right\rangle=\frac{1}{c_{n}} \sum_{\gamma n-\text { step SAW }}|\gamma(n)|^{2}=n^{2 \nu+o(1)} $$ with $\nu=3 / 4 .$

Is it possible to determine $A$? What method seems to be hopefully? it seems the complex structure can not tell us that and some new ingredients should be involved. At least twist the rotation number $$F(z)=F(a, z, x, \sigma)=\sum_{\gamma \subset \Omega: a \rightarrow z} \mathrm{e}^{-\mathrm{i} \sigma \mathrm{W}_{\gamma}(a, z)} x^{\ell(\gamma)}$$ is not concrete enough, because $$Z\left(x_{c}\right)=\sum_{\gamma \subset \Omega: a \rightarrow z} \mathrm{e}^{-\mathrm{i} \sigma \mathrm{W}_{\gamma}(a, z)} x_c^{\ell(\gamma)}=+\infty$$ maybe a mere concrete auxiliary function with some multilinear structure on the exponent part is helpful?