How did Gauss characterize the metrical relations in the uniform (4 4 4) tiling of the hyperbolic unit disk?

Question

My purpose is to verify an historical hypothesis I have on Gauss's tesselation of the unit disk as described in John Stilwell "Mathematics and its history". Looking at the relevant pages in Gauss's Nachlass (volume 8, p.102-105), I read that the commentor (Robert Fricke) on this fragment of Gauss says that Gauss's drawing (the (4 4 4) tesselation) is intended to be a geometrical illustration for composition of substitutions other then the fundamental generators of the modular group. The following sentences are a citation of Fricke about the substitutions Gauss used:

Gauss has repeatedly dealt with composition of other substitutions of the group defined from these generators. In addition to the information in fragment [i], the following formula should also be mentioned $$\frac{[\alpha, \beta,\dots,v]\theta + [\beta,\gamma,\dots,v]i}{-i[\alpha,\beta,\dots,\mu]\theta+[\beta,\gamma,\dots,\mu]}$$ which can be found in a booklet entitled “Cereri Palladi Junoni sacrum, Febr. 1805”. The continued fraction expansions of the two substitutions are given as examples:$$\frac{128\theta + 37i}{-45i\theta + 13}$$ $$ \frac{121\theta+36i}{-84i\theta+25}$$

Just to explain the words of Fricke, "the group defined from these generators" is the modular group (since the generators mentioned before this sentence are addition of $i$ and inversion with respect to the unit circle), and the $[,,\dots,]$ is the "Gaussian Brackets" notation (which Gauss defined in article 27 of Disquisitions Arithmeticae, and is connected directly to continued fractions). I think $\theta$ is just a notation for the complex variable that is transformed under the Mobius transformation.

Checking the determinants of these substitutions gave $-1$ for the first one and $+1$ for the second one, so this made me suspect that these are isometric Mobius transformations (the only thing that doesn't settle is that $a,b,c,d$ in the Mobius transformation should be real integers, not imaginary integers). One should also notice that the second Mobius transformation can be rewritten as:

$$\frac{121\theta+36i}{-84i\theta+25} = \frac{[3,2,1,3,3]\theta+[2,1,3,3]i}{-[3,2,1,3,2]i\theta+[2,1,3,2]}$$

, in exact accordance with Gauss's formula which uses the Gaussian Brackets notation (here $\alpha=3, \beta=2,\gamma=1, v=3, \mu=2$).

In addition, the diagonal elements of the second Mobius transformation ($121$ and $25$) are both reduced to $1$ modulo $12$, while the off-diagonal elements reduce to $0$ modulo $12$ (if one allows imaginary sizes for $b,c$). In other words, this shows that the second Mobius transform belongs to a congruence subgroup of level 12 in $SL_{2}(Z[i])$.

Although it seems at first that these Mobius transformations are just examples of a general principle of certain continued fractions developement, immediately after that Fricke says:

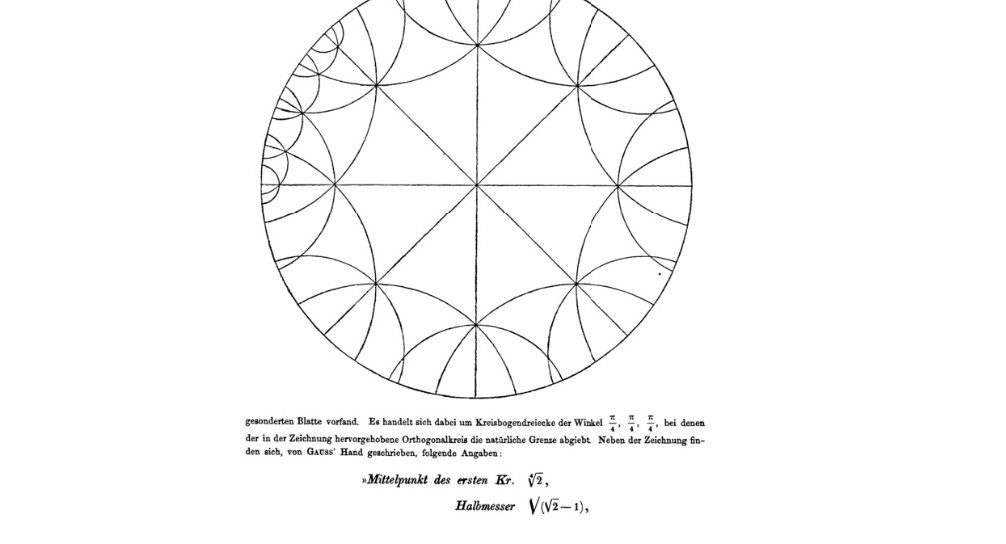
Both to explain the continued fraction development of the substitutions and to draw conclusions from the theory of functions, Gauss made use of the geometrical representation which has become the basis of the more recent theory of module functions. In the booklet just mentioned, Gauss drew the figure shown here. Since the above-mentioned continued fraction expansions of substitutions are also to be found, Gauss must have used the figure as a means to illustrate these continued fraction expansions. In fact, one has here the beginning of the well-known network of circular arc triangles, which is the basis of the theory of modulus functions. It's evident that Gauss generally understood the "principle of the symmetrical multiplication of curved triangles", which comes into consideration here, and even the character of the "natural limit" of a triangular network to be obtained in this way did not remain hidden... These are circular arc triangles of the angles $\frac{\pi}{4},\frac{\pi}{4},\frac{\pi}{4}$, and the orthogonal circle highlighted in the drawing represents their natural limit. In addition to the drawing, the following information was written by Gauss: "Center of the first circle: $2^{\frac{1}{4}}$, radius of the first circle: $\sqrt{\sqrt{2}-1}$, center of the second circle: $\frac{1}{2}(\sqrt{\sqrt{2}+1}+\sqrt{\sqrt{2}-1})$, radius of the second circle:$\frac{1}{2}(\sqrt{\sqrt{2}+1}-\sqrt{\sqrt{2}-1})$.

Therefore, it seems that these Mobius substitutions are actually the generators for some tiling of the hyperbolic disk. But this conclusion is a result of a very shallow reading of Fricke's comments and I lack the proffesional knowledge needed to verify my reading.

I also found another reference to Gauss's hyperbolic tiling in p.198 of Schlesinger's essay on Gauss's researches in function theory, where he says:

The extent to which Gauss must also be regarded as a precursor to the theory of automorphic functions is shown not only by his construction of the fundamental domain for the modular function, but also by his interpretation of the linear fractional substitution $$t'=\frac{(\alpha +\beta i)t-(\gamma + \delta i)}{(\gamma - \delta i)t+(\alpha-\beta i)}$$ as a rotation of the spherical surface in itself, which is discussed in two notes printed in volume 8, p.354-356 the works, and that relates to a case of transcendent triangular functions that do not correspond to the modular functions, a polygonal network, found in the p.104 of volume 8 of the works, after a statement on a seperate page.

In the introduction to the book "Papers on Fuchsian Functions" (which is available on internet archive), John Stillwell says several things that seem to me very helpful:

Triangle functions arise in connection with the hypergeometric differential equation of Gauss, as Gauss himself seems to have discovered. This can be guessed from Figure 3, which is an undated fragment found in his Nachlass (see Gauss's werke, volume 8, p. 102-105). The figure is a tessellation of the unit disk by curvilinear triangles with angles $\pi/4$, and it reflects the periodicity of the inverse to a certain quotient of solutions to the hypergeometric equation. The group in this case consists of certain linear fractional transformations which leave the unit disc invariant. Invariance under linear fractional transformations arises quite generally from second order linear differential equations as follows. If $y_1,y_2$ are two linearly independent solutions of $$\frac{d^2y}{dx^2}+p(x)\frac{dy}{dx}+q(x)=0$$ then any other solutions $y'_1,y'_2$ are linear combinations of $y_1,y_2$: $$y'_1 = ay_1+by_2$$ $$y'_2 = cy_1+dy_2$$ hence the quotient of the new solutions is related to the quotient of the old by a linear fractional transformation: $$\frac{y'_1}{y'_2} = \frac{a\eta+b}{c\eta+d}$$ where $\eta = \frac{y_1}{y_2}$. By inverting the quotient of solutions one obtains a function automorphic with respect to a certian group of linear transformations. In the case of the hypergeometric equations, one is led to groups of automorphisms of certain triangle tessellations of the unit disc. The Gauss's figure is one such tessellation. Others were found by Riemann in lectures of 1858-1859 (discovered only in 1897 and published in Riemann [1902]), and the general theory was worked out independently by Schwarz [1872].

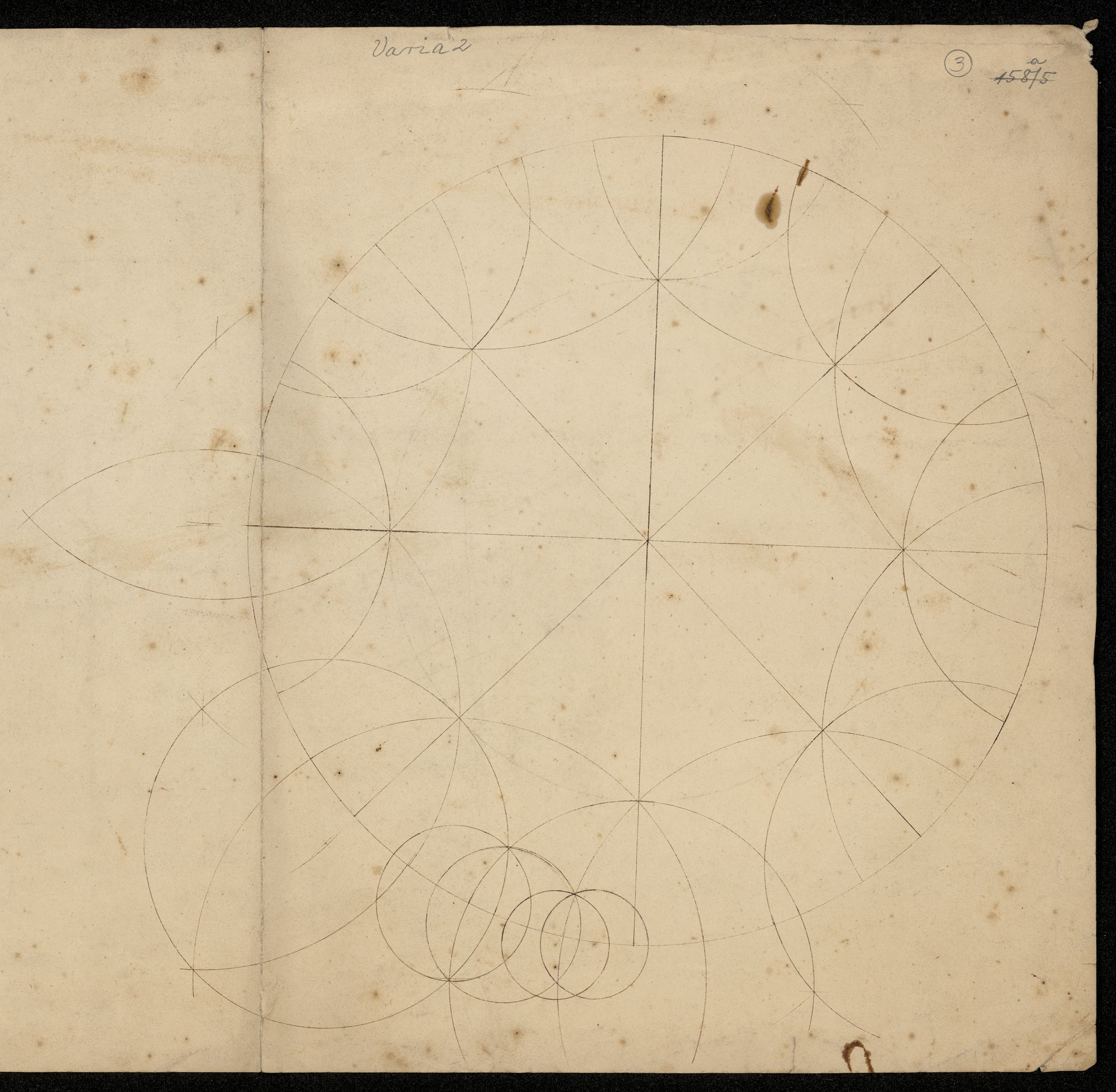
I don't now on what sources Stillwell bases his conjecture about the origin of Gauss's tessellation. After I asked people at the department for handwritten manuscripts and rare prints of the Gottingen university to search for the location of Gauss's drawing, they managed to find it after many efforts. Gauss's drawing (together with his related notes on the metrical relations) from p.104 of volume 8 of his works appears in an obscure notebook called Cod. Ms. Gauss Varia 2, as can be seen from this link.

After gaining access to this notebook, I saw only one more drawing in it related to this tessellation - but again, with no supporting context.

Therefore, I believe my attempts to answer that question arrived into a deadlock, and I don't understand how Stillwell came with his "hypergeometric function conjecture" about the origin of this tessellation. However, I still believe his notes on the mertical relation exhibit knowledge of the Poincare disk model (simply because I cannot explain their correctness in other ways).

Historical significance of Gauss's results:

Being the first drawing of it's kind, the tessellation drawed by Gauss and his related results have planted some the seeds of Felix Klein's "Erlangen program" (with the other influences being Galois's theory of equations and Riemann's geometric ideas). Klein read Gauss's fragments very closely and seems to have been influenced by them, so I think it's not an exaggeration to say that Gauss's drawing was one of his sources of inspiration.

Therefore I believe that for a correct historic appreciation of the roots of Erlangen program, it's important to know how Gauss arrived at the metrical relations in his tiling (the results stated by Gauss on the location and radiuses of the centres of the first and secondary circles in his tessellation are already confirmed by my posted answer - although it might not be the original method of Gauss).

Questions

Do Gauss's formulas for the radiuses of curvature of the first and second generation circles imply that he essentialy knew the Poincare disk model of the hyperbolic plane? I ask that because the derivation presented makes heavy use of the conception and machinery of this model. I find it unprobable, at least according to traditional accounts of Gauss's mathematical interests (the disk model is essentially a conformal model of the hyperbolic plane, and Gauss didn't think about conformal mappings until at least 1815).

It's more likely that he arrived at this result in the context of his analytic investigations, and not in the context of his "meditations" on non-Euclidean geometry.

Answer 1

This is an answer that is intended to verify the statements in Gauss's fragment - the four statements about the network of curved triangles. In order to facilitate the whole deduction of Gauss's formulas as presented in this answer, I added the original drawing of Gauss (to help visualize the geometric relations).

Radius and distance of the center of the first circles:

Theorem: Each of the eight curved triangles with one vertex at the origin $(0,0)$ of the Poincare unit disk has two straight sides which are portions of diameters of this disk and one curved side which is a portion of a circle orthogonal to the unit circle. The center of curvature of this orthogonal circle is at distance $2^{\frac{1}{4}}$ from the origin and it's radius of curature is $\sqrt{\sqrt{2}-1}$ (as in Gauss's fragment).

Proof:

The length $a$ of a side of an equilateral hyperbolic triangle with angles $\alpha=\pi/4,\beta=\pi/4,\gamma=\pi/4$ in the "real" hyperbolic plane (not the euclidean distance in the Poincare disk model of it) is according to the laws of hyperbolic trigonometry:

$$\cosh(a) = \frac{\cos\alpha}{1-\cos\alpha} = \frac {1}{\sqrt{2}-1} = \sqrt{2}+1\implies \tanh(a) = \sqrt{2}\sqrt{\sqrt{2}-1}\implies a = \frac{1}{2}\ln(\frac{1+\sqrt{2}\sqrt{\sqrt{2}-1}}{1-\sqrt{2}\sqrt{\sqrt{2}-1}})$$

The relation between the "euclidean" distance $d$ between each of the other two vertexes to the origin and the corresponding real hyperbolic distance $a$ is, according to the formulas of Cayley-Klein metrics:

$$\ln(\frac{1+d}{1-d}) = a \implies \frac{1+d}{1-d} = \sqrt{\frac{1+\sqrt{2}\sqrt{\sqrt{2}-1}}{1-\sqrt{2}\sqrt{\sqrt{2}-1}}}\implies d = \sqrt{\sqrt{2}-1}$$

Therefore the coordinates of the two other vertexes, after aligning the x-axis with one of the straight sides of the curved triangle, are:

$$(\sqrt{\sqrt{2}-1},0),(\frac{\sqrt{\sqrt{2}-1}}{\sqrt{2}},\frac{\sqrt{\sqrt{2}-1})}{\sqrt{2}})$$

Now the equation of any circle orthogonal to the unit circle is of the form:

$$x^2+y^2+ax+by+1 = 0$$

Substituting the x,y coordinates of the two points, one gets two linear equations with variables $a,b$, whose solution is:

$a = -\frac{\sqrt{2}}{\sqrt{\sqrt{2}-1}}, b = a(\sqrt{2}-1)$

Since the canonic form of the equation of the orthogonal circle is:

$$(x+a/2)^2+(y+b/2)^2= \frac{a^2+b^2}{4}-1 = r^2$$

where $r$ is the radius of curvature of the orthogonal circle, one gets the desired theorem by the following calculation:

$$r^2 = \frac{a^2+b^2}{4}-1 = \frac{a^2(1+(\sqrt{2}-1)^2)}{4}-1 = \frac{2\cdot(1+(\sqrt{2}-1)^2)}{(\sqrt{2}-1)\cdot 4}-1 = \frac{8-4\sqrt{2}}{(\sqrt{2}-1)\cdot 4}-1 = \sqrt{2}-1 \implies r = \sqrt{\sqrt{2}-1}$$

and the location of the center of curvature of this circle is at $(-\frac{a}{2},-\frac{b}{2})$, so its distance from the origin is:

$$\sqrt{\frac{a^2+b^2}{4}} = \sqrt{r^2+1} = 2^{\frac{1}{4}}$$

Q.E.D

Radius and distance of the center of the secondary circles:

The principle of generation of the curved triangles network is, as with all tesselations of the plane (whatever it's geometry is euclidean, hyperbolic or spherical), the successive reflection of the triangles with respect to their sides; in this way we fill the whole plane with triangles. In euclidean geometry, one can generate the tiling by simply reflecting the triangles with respect to the sides; however, in hyperbolic geometry one needs to generalize the notion of reflection from reflection with respect to line to reflection with respect to a circle.

The notion required is therefore that of inversion with respect to a circle; the secondary circular arcs (of the "second generation triangles") are inversions of the straight sides of the "first generation triangles" with respect to the first circular arcs. The formula for the radius $r'$ of the inversion of a circle with radius $r$ with respect to a smaller circle with radius $k$ whose center is at distance $d$ from the center of the inverted circle is:

$$r' = \frac{k^2r}{d^2-r^2} = \frac{k^2}{d^2/r-r}$$

In our problem: $k = \sqrt{\sqrt{2}-1}, d^2 = r^2+x^2 -2xr\cos(5\pi/8), x = \sqrt{\sqrt{2}}, -\cos(5\pi/8) = \sin(\pi/8) = \sqrt{\frac{1-\frac{1}{\sqrt{2}}}{2}} $.

In this notation, $x$ is the distance of the center of the first circles from the origin, and $d$ is derived using the euclidean cosine theorem. One needs to calculate $r'$ in the limit where $r$ tends to infinity since the straight sides of the first triangle can be thought of as circles with infinite radius.

Therefore:

$$r' = \lim_{r\to \infty}\frac{(\sqrt{\sqrt{2}-1})^2}{(r^2+x^2 -2xr\cos(5\pi/8))/r - r} = \frac {\sqrt{2}-1}{-2x\cos(5\pi/8)} = \frac{\sqrt{2}-1}{(\sqrt{2-\sqrt{2}})\cdot\sqrt{\sqrt{2}}} = \frac{\sqrt{2}-1}{\sqrt{2(\sqrt{2}-1)}} = \frac{\sqrt{\sqrt{2}-1}}{\sqrt{2}}$$

one can easily verify that the result for $r'$ is equal to the formula given by Gauss (simply by squaring both sides). The result for the distances $x'$ of the centers of the secondary circles from the origin is then easily obtained if we keep in mind that, for any $n$th generation circles: $x^2_n-r^2_n = 1$.

Final remarks:

The derivation presented here involves several conceptual/technical steps:

• Calculation of the length of sides of the curved triangles in the real hyperbolic plane - this is done by using the laws of hyperbolic geometry (the hyperbolic cosine theorem). This step alone shows Gauss thought of the network of curved triangles as a tiling of the hyperbolic plane by hyperbolic triangle.

• The use of Cayley-Klein metric - the whole procedure of generation of this curved triangles network can be viewed through euclidean eyes; one needs the concept of inversion of circle in a smaller circle to get a recursive definition of the network. However, Gauss deliberately gives sizes of the first triangles that correspond to tessellation of the unit disk (and not, for example, to a disk of radius 2), and i can't see any way to calculate the required size of the first triangles (required in order to make the unit circle the natural border of this network) without using the Cayley–Klein metric.

• The conception of a "model" of the hyperbolic plane - the final step makes use of the fact that the straight lines of the Poincare disk model are circular arcs orthogonal to the natural border of the network (the unit circle), and this is exactly the defining property of this model (This fact is used in the derivation of the equation of circle orthogonal to the unit circle).

Goettingen State and University Library, Cod. Ms. Gauß Varia 2, p. 5.

I added here another drawing from the notebook in which he drew his $(4\ 4\ 4)$ tessellation - in this drawing the completion of the sides of triangles to full circles orthogonal to the natural border (the unit circle) is shown more clearly. This drawing, together with the calculations involved, certainly proves he conceived of straight lines as circular arcs perpendicular to the unit circle, with exact correspondence with Poincare disk model.

Remaining questions

I never read any claim that Gauss knew this model by any of the reliable sources on the history of mathematics. In addition, those results of Gauss appear isolated from his body of mathematical research, and that is why I think there is a need to place it in the right context.

The remaining questions are:

• How he originally found those results?
• How he was led to consider this particular tiling?

I know those questions are very hard to answer without access to his original notebook, but maybe an expert in this field can place Gauss's results in the right context by a kind of "extrapolation" on all known sources.