Games and Ramsey's theorem

Question

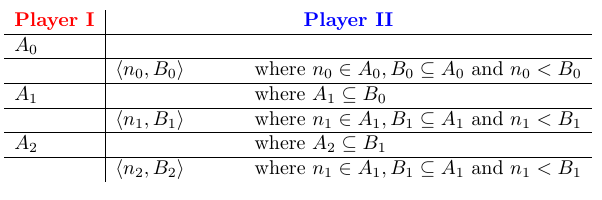
Here, we identify subsets of $\mathbb{N}$ with sequences obtained by listing the members of the set in strictly increasing order. Suppose that we have some set $\mathcal{F}$ of sets (sequences) of the form $(m_n, l_n)_{n=1}^t$. We let $\mathcal{U}$ denote the set of all $(m_n, l_n)_{n=1}^\infty$ such that $(m_n, l_n)_{n=1}^t\in \mathcal{F}$ for all $t\in\mathbb{N}$. We let $\mathcal{V}$ denote the set of all $(m_n)_{n=1}^\infty$ such that there exists $(l_n)_{n=1}^\infty$ such that $(m_n, l_n)_{n=1}^\infty \in \mathcal{U}$.

It is easy to see that $\mathcal{U}$ is closed and $\mathcal{V}$ is analytic (in either the Cantor or Ellentuck topology). Suppose also that for every infinite subset $K$ of $\mathbb{N}$, there exists a further, infinite subset $M$ of $L$ such that every infinite subset of $M$ lies in $\mathcal{V}$.

Is it known that the latter property is equivalent to a winning strategy for a certain player in some two player game?

Answer 1

If you mean completely Ramsey sets there is the following characterization using the Kastanas game. Let's start with some definitions :

1. Here the letters $a, b, c, ...$ vary over finite subsets of $\mathbb{N}$ and $A, B, C, ...$ over infinite subsets of $\mathbb{N}$.

   • Let $a$ and $A$, we write $a<A$ if $a=\emptyset$ or $max(a)<min(A)$.

   • For $a<A$, let $$[a, A]=\{S\in [\mathbb{N}]^{\aleph_{0}} : a\subseteq S\subseteq a\cup S \}= \{a\cup C\in [\mathbb{N}]^{\aleph_{0}} : a<C\subseteq A \}$$ Note that $[\emptyset, A]=[A]^{\aleph_{0}}$

   • A set $X\subseteq [\mathbb{N}]^{\aleph_{0}}$ is called Ramsey if there is $A$ with $[\emptyset, A]\subseteq X$ or $[\emptyset, A]\subseteq\hspace{0.1cm}\sim X$, here $\sim X$ denotes the complement of $X$.

   • A set $X\subseteq [\mathbb{N}]^{\aleph_{0}}$ is called completely Ramsey if for every $a<A$ there is $B\subseteq A$ with $[a, B]\subseteq X$ or $[a, B]\subseteq\hspace{0.1cm} \sim X$.

2. Let $A \subseteq [\omega]^{\omega}$ = the set of infinite sets of integers. Then $A$ has a homogeneous set $H$ if, by definition, $H \in [\omega]^{\omega}$ and either every infinite subset of $H$ belongs to $A$ or every infinite subset of H belongs to the complement of $A$. $A$ has the Ramsey property iff it has a homogeneous set.

3. Kastanas's game. For $\varphi\subseteq [\omega]^{\omega}$ we define the game $G_{\varphi}$ as follows :

Player I wins if $\{n_{0}, n_{1}, \cdots \}\in\varphi$

Theorem (Kastanas)

a.) Player I has a winning strategy in $G_{\varphi}$, iff there is a homogeneous set in $\varphi$ (i.e. an infinite $H$ such that every infinite subset of it belongs to $\varphi$)

b.) Player II has a winning strategy in $G_{\varphi}$ iff for every $A$ there is a subset of it homogeneous in $[\omega]^{\omega}\setminus \varphi$.

For the information of the bibliography we have the following article "On the Ramsey Property for Sets of Reals" Author: Ilias G. Kastanas.