14
$\begingroup$

I am preparing the notes for a course in Algebraic Topology, so I decided to borrow some of the material from the classical (and wonderful) book by G. Bredon Topology and Geometry.

Looking at the part regarding the orientation of a topological $n$-manifold $M^n$, at page 341 we find the following well-known result, with its usual proof (Proposition 7.1):

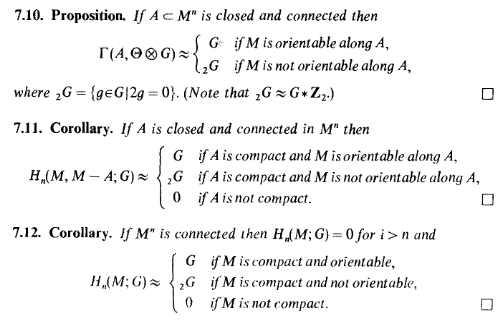
enter image description here

So far, so good. However, after five pages we find what follows:

enter image description here

This makes me confused, for at least two reasons:

Point 1. The Note after the statement of Proposition 7.10 does not make any sense to me. As defined, the symbol ${}_2G$ denotes the $2$-torsion part of the abelian group $G$, so if $G$ is torsion-free (for instance, if $G=\mathbf{Z}$) then ${}_2G=0$. This is clearly very different from the free-product $G \ast \mathbf{Z_2}$ (here $\ast$ seems to denote the free-product, see pages 158-159).

Point 2. In Corollary 7.11, take $A=\{x\}$ and $G=\mathbf{Z}$. Then, when $M$ is not orientable one finds $H_n(M, \, M-\{x\}, \, \mathbf{Z})=0$, and this contradicts Proposition 7.1, that yields the (correct, as far as I know) result $H_n(M, \, M-\{x\}, \, \mathbf{Z})= \mathbf{Z}$.

Question. Are the issues risen in Points 1, 2 above really mistakes in Bredon's book, or perhaps am I missing something trivial?

Improve this question
$\endgroup$
13
  • 2
    $\begingroup$ I suspect that the words "orientable along $A$" mean something like "a neighbourhood of $A$ is orientable", in which case corollary 7.11 would be correct $\endgroup$
    – Denis Nardin
    Commented Jan 24, 2019 at 11:47
  • 4
    $\begingroup$ Every point has an orientable neighborhood (say, a ball), hence $M$ is always orientable along $\{x\}$, so corollary 7.11 says that for every manifold $M$ the formula you give holds. $\endgroup$
    – Denis Nardin
    Commented Jan 24, 2019 at 11:59
  • 9
    $\begingroup$ Also it seems that Bredon indicates with $\ast$ what I would call $\mathrm{Tor}_1$, so in particular $A\ast \mathbb{Z}/n$ is exactly the $n$-torsion of $A$. $\endgroup$
    – Denis Nardin
    Commented Jan 24, 2019 at 12:07
  • 3
    $\begingroup$ Well, at page 158 it also indicate by $*$ the free product, and in a book of 550 pages it is not easy to understand where the same notation indicates two very different things. Now it makes sense, thanks! $\endgroup$
    – Francesco Polizzi
    Commented Jan 24, 2019 at 12:09
  • 6
    $\begingroup$ @GeraldEdgar: Bredon died in 2000, and there is no webpage available. On the Springer's webpage there is no errata, either. Actually, on the web I found nothing (well, maybe I did not look well enough). $\endgroup$
    – Francesco Polizzi
    Commented Jan 24, 2019 at 14:51

2 Answers 2

Reset to default
35
$\begingroup$

Star (in older topology texts) often indicate torsion product of abelian groups, that is, $A * B := \operatorname{Tor}_{\Bbb Z}(A, B)$. Usually it is clear from the context whether free product or torsion product is meant.

Improve this answer
$\endgroup$
7
  • 8
    $\begingroup$ Thanks. I was not aware of this (old) notation. $\endgroup$
    – Francesco Polizzi
    Commented Jan 24, 2019 at 13:34
  • 5
    $\begingroup$ (This notation is also used in Spanier's text, for example.) $\endgroup$
    – Pedro
    Commented Jan 24, 2019 at 13:51
  • 9
    $\begingroup$ @FrancescoPolizzi I'm allegedly a specialist in Algebraic Topology, and I didn't know either, so don't feel too bad :) $\endgroup$
    – Denis Nardin
    Commented Jan 25, 2019 at 12:22
  • 3
    $\begingroup$ Bredon carefully defines A*B on page 278 in a section called More homological algebra and even says that it is often denoted Tor(A,B). $\endgroup$
    – Nicholas Kuhn
    Commented Jan 25, 2019 at 17:31
  • 7
    $\begingroup$ Yes, but again: the book is 550 pages long, one that is just interested in one topic cannot read carefully all of it. Since at page 158 Bredon uses $*$ to indicated to free product, I supposed (maybe ingenuously) that this notation would have been kept in all the book, even because I never met the notation $*$ for $\mathrm{Tor}_1$ before. $\endgroup$
    – Francesco Polizzi
    Commented Jan 25, 2019 at 18:55
9
$\begingroup$

I think that you are missing the definition of 'orientable along $A$'. I haven't got that book of Bredon to hand, but presumably 'orientable along $A$' means that if you move a local orientation of $M$ around a closed path that stays in $A$ then it will come back to the same local orientation. In particular, in the case when $A$ is a single point, then $M$ will always be orientable along $A$, regardless of whether $M$ is orientable or not, so the case that you view as wrong doesn't arise.

I agree with Denis T's interpretation of the notation $A*B$.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Yes, definitely I was confused about the definition of "orientable along $A$". And I was unaware of the old notation $A*B$ for $\mathrm{Tor}_1(A, \, B)$. $\endgroup$
    – Francesco Polizzi
    Commented Jan 25, 2019 at 17:27

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .