Antipode of Hopf algebra in braided monoidal category is an algebra antihomomorphism?

Question

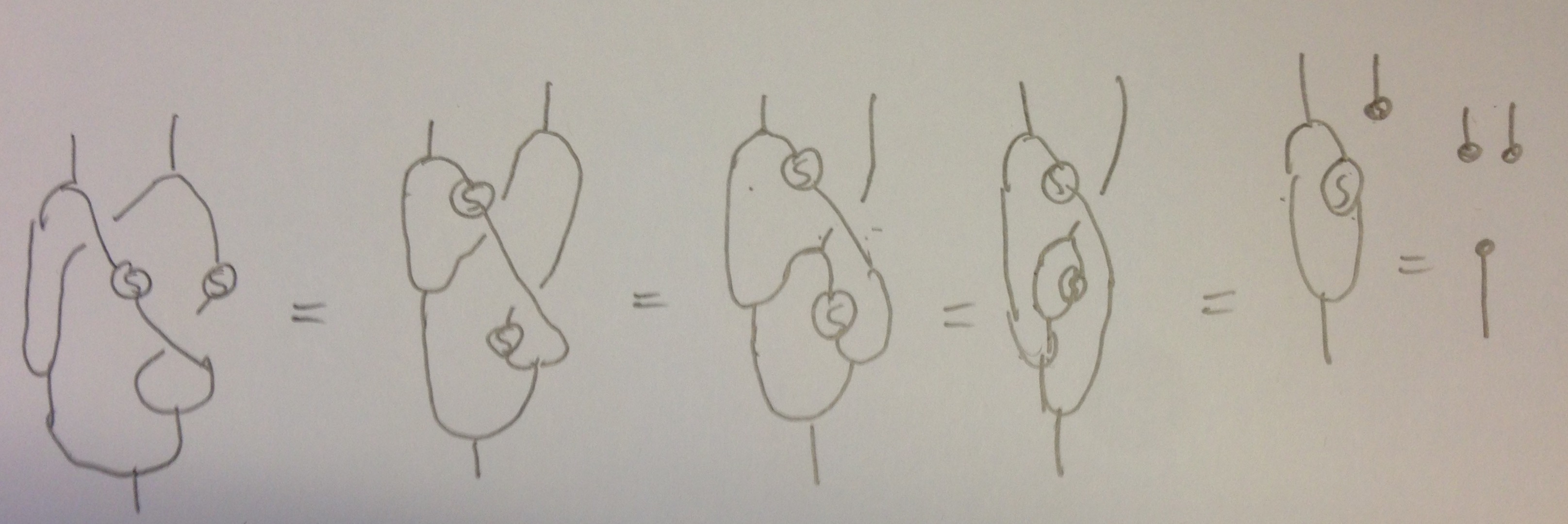
I realize that the question posed in the title has already been addressed here: Identities that connect antipode with multiplication and comultiplication, where the graphical calculus proof provided by Majid is cited. However, I am wondering if there is a more algebraic proof that can be formulated without the use of graphical calculus.

If we have a Hopf algebra $(H, \mu, \eta, \Delta, \varepsilon, S)$ in the category of vector spaces, where $\mu$ is the multiplication map, $\eta$ is the unit map, $\Delta$ is the comultiplication, $\varepsilon$ is the counit, and $S$ is the antipode, then one can show that $S \circ \mu$ is a left convolution inverse of $\mu$ and that $\mu \circ \tau \circ (S \otimes S)$ is a right convolution inverse of $\mu$ (where $\tau$ is the flip map). From here, then $S \circ \mu = \mu \circ \tau \circ (S \otimes S)$.

I am aiming to replicate the above proof for an arbitrary braided monoidal category, replacing the flip map $\tau$ with a braiding $\sigma$. Showing that $S \circ \mu$ is a left convolution inverse of $\mu$ is easy, just using that the comultiplication $\Delta$ is an algebra homomorphism. I can not seem to get that $\mu \circ \sigma \circ (S \otimes S)$ is a right convolution inverse of $\mu$, however. We should have \begin{align*} &\mu \ast (\mu \circ \sigma \circ (S \otimes S))\\ &= \mu \circ (\mu \otimes \mu) \circ (\operatorname{id} \otimes \operatorname{id} \otimes \sigma) \circ (\operatorname{id} \otimes \operatorname{id} \otimes S \otimes S) \circ (\operatorname{id} \otimes \sigma \otimes \operatorname{id}) \circ (\Delta \otimes \Delta) \end{align*} but I can not see how to get anywhere with this, particularly trying to figure out what to do with the map $\operatorname{id} \otimes \operatorname{id} \otimes \sigma$. It seems as though the antipode equation for $H \otimes H$ should be used, but I am not quite sure how to implement it. Any ideas would be appreciated.

Answer 1

This idea should work and Majid's proof uses this. I think your reasoning illustrates how the proof is related to the one in the (non braided) Hopf algebra case.

You can verify that $\mu\circ \sigma\circ(S\otimes S)$ indeed is a right convolution inverse of $\mu$ as follows. First, by naturality of $\sigma$ twice you get \begin{align*} &\mu \circ (\mu \otimes \mu) \circ (\operatorname{id} \otimes \operatorname{id} \otimes \sigma) \circ (\operatorname{id} \otimes \operatorname{id} \otimes S \otimes S) \circ (\operatorname{id} \otimes \sigma \otimes \operatorname{id}) \circ (\Delta \otimes \Delta)\\ &=\mu \circ (\mu \otimes \mu(S\otimes\operatorname{id})) \circ (\operatorname{id} \otimes \operatorname{id} \otimes \sigma) \circ (\operatorname{id} \otimes \sigma \otimes \operatorname{id}) \circ ((\operatorname{id}\otimes S)\Delta \otimes \Delta). \end{align*} Then apply naturality of braiding (after using the Yang-Baxter equation of the braiding) to $\Delta$, yielding that the above equals (if you also use associativity of multiplication twice) \begin{align*} \mu\circ(\mu\otimes \operatorname{id})\circ(\operatorname{id}\otimes \mu(\operatorname{id}\otimes S)\Delta\otimes \operatorname{id})\circ(\operatorname{id}\otimes \sigma)\circ((\operatorname{id}\otimes S)\Delta \otimes \Delta). \end{align*} Here, you can find the term $\mu(\operatorname{id}\otimes S)\Delta$ which equals $1\circ\varepsilon$ and simplifies everything (under use of the axioms of the unit), to $$(\mu(\operatorname{id}\otimes S)\Delta)\otimes \varepsilon$$ which now gives $1\circ(\varepsilon\otimes \varepsilon)$.

You need to convince yourself that if a map has a right and left convolution inverse, they are equal. The same proof of this fact also works in a braided monoidal category as it only uses (co)associativity. Note that this does not require that $H\otimes H$ is a Hopf algebra (which it is not in general). However, $H\otimes H$ is both a coalgebra and algebra and this is all we need.

The above reasoning will again be more clear to me when using graphical calculus. Without it, I would have a hard time coming up with the steps necessary:

(Note that the functional identities above do repress $\circ$ when it appears within tensor product legs)

Answer 2

You could also look at Proposition 1.22, and the commutative diagram on page 15 of Aguiar and Mahajan's book "Monoidal Functors, Species, and Hopf Algebras":

http://www.math.cornell.edu/~maguiar/a.pdf

Admittedly, I find the graphical calculus arguments easier to follow, but if you want to work in a braided monoidal category, you have to work directly with morphisms, and sometimes it is hard to keep track of domains and codomains, so a commutative diagram approach might be easier to parse.

In general, the graphical calculus is actually very useful for figuring out the necessary intermediate steps.