Describing the shape of orthogonal complements to points in arc

Question

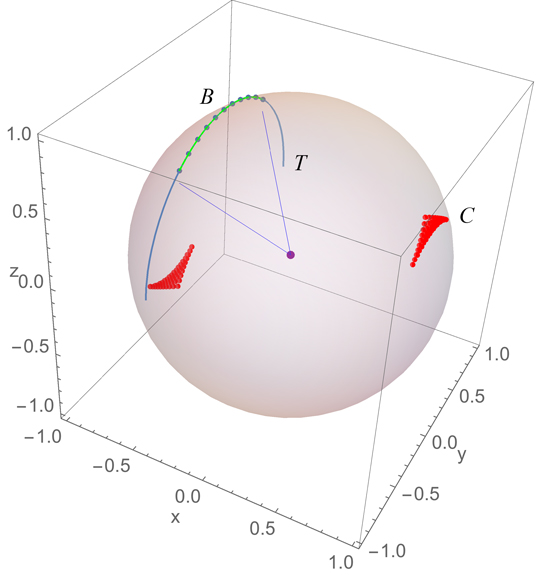
I define a circle $T$ in $\mathbb{R}^3$ with equations $y^2+z^2=3/4$ and $x=-1/2$. This circle lies on the unit sphere $S$. I define the set $B$ to be all points on $T$ with y-values between $\epsilon$ and $-\epsilon$ and positive z-values (that is, a small arc at the top of $T$). For every two points in $B$, consider their corresponding vectors, and find the unit vector orthogonal to both. (Assume we choose the orthogonal vector with positive x-value). Let's call the set of all of these orthogonal vectors $C$. (I will also use $C$ to describe the set of points in $\mathbb{R}^3$ corresponding to these vectors).

My question is, what does $C$ look like (as a set of points)? Is it also an arc of a circle? Perhaps a circle parallel to the xy-plane? For my research problem I'm trying to show that we can choose $\epsilon$ small enough that the points of $C$ will be very close together (this seems obvious to me but I'm having trouble showing it). Instead of defining $T$ as is, is it better to define a larger circle closer to the Prime Meridian (so to speak) of $S$? Thank you.

Answer 1

Not a precisely quantitative answer. Just attempting to track the specified geometry. I used $\epsilon=0.1$ radians to delimit the subset $B \subset T$.

My question is, what does $C$ look like (as a set of points)?

It "looks like" as indicated below:

---

 

---

Pardon that I did not

(Assume we choose the orthogonal vector with positive x-value)

but instead showed both $\pm$.

$C$ appears to be bounded by a circular arc connected to a V whose angle is determined by $\epsilon$, in particular, the straight-line boundaries of $C$ are orthogonal to the $\epsilon$ extremes of $B$, as is the circular arc boundary, more clearly seen from $(+\infty,0,0)$:

---

---

In any case, have a nice day! :-)