I'm not too familiar with quasi-crystals, but I was recently playing around with a particular discrete function and I got the following neat pattern:
This is just a small segment, but as far as I could tell it does not become periodic. So I am wondering whether this is a quasi-crystal. More exactly:
- How would I go about figuring out whether or not this is a quasi-crystal?
- Does anyone recognize this pattern?
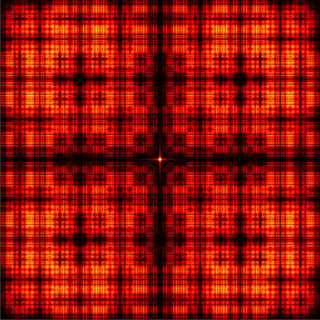
Knowing of the peculiar diffraction patterns of quasi-crystals, I took the Fourier transform of the above and obtained the following (again just showing a segment):
(Details: this is in log-scale, with bright colors denoting high values, dark low values)
I find this quite beautiful and hence I'd love to have a better understanding of what's going on. In particular it seems to me that this might be a fractal. Again I am wondering how to formalize this and/or what the best way would be to advance if I want to 'understand' either of these patterns. Basically I'm not sure what to 'look for', which also makes it hard to make my question very concrete.