Why does vertical multiplication in 2-groups not follow the same order as horizontal one when constructed from crossed modules?

Question

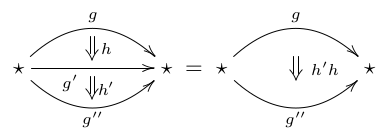
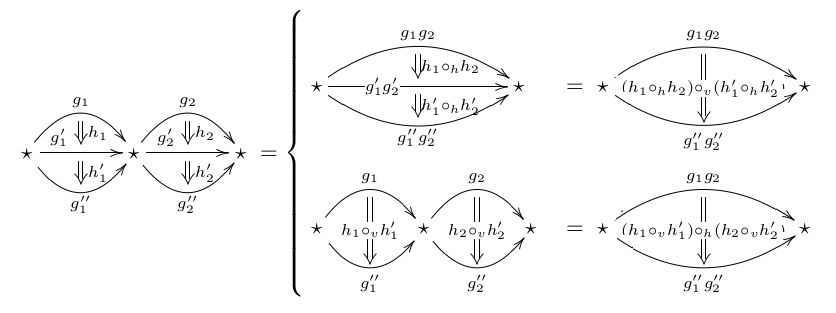
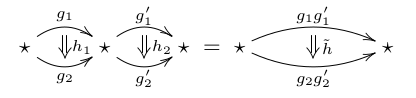Consider a 2-group (seen as a 2-category with only one object $\star$) constructed from a crossed module $(G,H,t,\rhd)$ ($\circ$ will denote 1-morphisms composition, $\circ_{h}$ 2-morphisms horizontal composition, $\circ_{v}$ 2-morphisms vertical composition, when composed using them, symboles will mean (1,2)-morphisms, otherwise, they will simply mean elements of the groups $G$ and $H$), choosing a given convention we get horizontal composition of 1-morphisms:

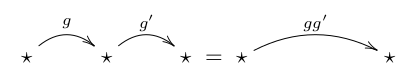
with $gg'=g\circ g'$ is done using composition law of $G$. We get also, using the same convention, horizontal compostion of 2-morphisms:

with $\tilde{h}=h_{1}(g_{1}\rhd h_{2})=h_{1}\circ_{h}h_{2}$ is done using both action of $G$ on $H$ and compostion law in $H$. In the two precedent compositions, “order” of elements is preserved when composing. Why then the same thing does not happen in vertical composition of 2-morphisms? Indeed,

where $h'h=h\circ_{v}h'$ is done with composition law in $H$. Knowing that, $\circ_{v}$ has the same “origin” as $\circ$, in the sens that, $\circ_{v}$ is the composition law (function) in the category internal to the category having $\circ$ as its composition law (functor).

Answer 1

The answer lies in the so called interchange law, which is nothing more than the functoriality condition of $\circ$, indeed,

which means that,

$(h_{1}\circ_{h}h_{2})\circ_{v}(h_{1}'\circ_{h}h_{2}')=(h_{1}\circ_{v}h'_{1})\circ_{h}(h_{2}\circ_{v}h'_{2})$ $[h_{1}(g_{1}\rhd h_{2})]\circ_{v}[h'_{1}(g'_{1}\rhd h'_{2})]=(h_{1}\circ_{v}h'_{1})g_{1}\rhd(h_{2}\circ_{v}h'_{2})$ $[h_{1}(g_{1}\rhd h_{2})]\circ_{v}[h'_{1}((t(h_{1})g_{1})\rhd h'_{2})]=(h_{1}\circ_{v}h'_{1})(g_{1}\rhd h_{2}\circ_{v}g_{1}\rhd h'_{2})$ $[h_{1}(g_{1}\rhd h_{2})]\circ_{v}[h'_{1}(t(h_{1})\rhd(g_{1}\rhd h'_{2}))]=(h_{1}\circ_{v}h'_{1})(g_{1}\rhd h_{2}\circ_{v}g_{1}\rhd h'_{2})$ $[h_{1}(g_{1}\rhd h_{2})]\circ_{v}[h'_{1}(h_{1}(g_{1}\rhd h'_{2})h_{1}^{-1})]=(h_{1}\circ_{v}h'_{1})(g_{1}\rhd h_{2}\circ_{v}g_{1}\rhd h'_{2})$

If $h\circ_{v}h'=hh'$ we get:

$h_{1}(g_{1}\rhd h_{2})h'_{1}h_{1}(g_{1}\rhd h'_{2})h_{1}^{-1}\neq h_{1}h'_{1}g_{1}\rhd h_{2}\circ_{v}g_{1}\rhd h'_{2}$

whereas if $h\circ_{v}h'=h'h$ we get the correct equality:

$[h_{1}(g_{1}\rhd h_{2})]\circ_{v}[h'_{1}(h_{1}(g_{1}\rhd h'_{2})h_{1}^{-1})]\stackrel{?}{=}(h_{1}\circ_{v}h'_{1})(g_{1}\rhd h_{2}\circ_{v}g_{1}\rhd h'_{2})$ $h'_{1}h_{1}(g_{1}\rhd h'_{2})h_{1}^{-1}h_{1}(g_{1}\rhd h_{2})\stackrel{?}{=}(h'_{1}h_{1})(g_{1}\rhd h'_{2})(g_{1}\rhd h_{2})$ $h'_{1}h_{1}(g_{1}\rhd h'_{2})(g_{1}\rhd h_{2})=h'_{1}h_{1}(g_{1}\rhd h'_{2})(g_{1}\rhd h_{2})$