7
$\begingroup$

Let $U^* \rightarrow H^* $ be the homomorphism describing the complex orientation of $H^* $ from complex cobordism. Let $t_1, t_2, ...$ be indeterminates.

My question is: Does there exist an integral cohomology operation $H^* (X) \rightarrow H^*(X)[\mathbf{t}]$ that makes the following diagram commute for any $X$? If so can we describe it in a way that doesn't involve complex cobordism?

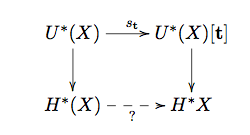
alt text

Here $s_{\mathbf{t}}$ is the total Landweber-Novikov operation.

In particular, since the Landweber-Novikov operation satisfies the Riemann-Roch type formula for proper, complex-oriented maps $$ s_{\mathbf{t}} f_{*}x = f_{*}(c_{\mathbf{t}}(\nu_{f}) \cdot s_{\mathbf{t}}x)$$ (where $\nu_{f}$ is the virtual class $1-\nu_i$ and $\nu_i$ is the stable normal bundle of the proper, complex-oriented map $f: Z \rightarrow X$) we would expect ths operations to satisfy something similar.

EDIT: Whoops, original title didn't have to do with the question :) But it did have to do with the motivation behind asking it! Maybe another time...

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
5
$\begingroup$

This is more of a comment than an answer. I just wanted to point out that such an operation, if it were to exist, cannot be stable.

This follows from the answer to Integral cohomology (stable) operations, which asserts that the set of stable cohomology operations in integral cohomology of a given degree $2k>0$ is finite, and the observation that $s_{(k)}(cf_1) = cf_1^{k+1}$, where $cf_1\in U^2(BU)$ is the first universal Conner-Floyd-Chern class.

To see this, suppose that there is a stable operation $\sigma_{(k)}:H^\ast(-)\to H^{\ast+2k}(-)$ which extends the stable operation $s_{(k)}:U^\ast(-)\to U^{\ast+2k}(-)$. Then we would have $$ \sigma_{(k)}(c_1)= c_1^{k+1}, $$ where $c_1\in H^2(BU)$ is the first universal Chern class. But since the sum of stable operations is stable, we would have $$ n\sigma_{(k)}(c_1)=0 $$ for $n$ sufficiently large, contradicting the fact that $nc_1^{k+1}\neq 0$.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Alternatively, since $BU=K(\mathbb{Z},2)$, you can simply observe directly that the operation $x\mapsto x^{k+1}$ for $|x|=2$ does not extend to a stable operation. $\endgroup$
    – Eric Wofsey
    Commented Dec 17, 2012 at 20:46
  • 1
    $\begingroup$ Certainly $BU$ is not a $K(\mathbb{Z}, 2)$, Eric... It has all sorts of homotopy groups... $\endgroup$
    – Dylan Wilson
    Commented Dec 17, 2012 at 20:51
  • 1
    $\begingroup$ Oops, sorry, my brain is not functioning. I read that as $BU(1)$. $\endgroup$
    – Eric Wofsey
    Commented Dec 17, 2012 at 20:54
  • $\begingroup$ A variant of this argument also shows that you can't have an unstable operation: just look at $\Sigma c_1$ in $\Sigma BU$. Since all unstable operations on odd-degree classes are torsion, no unstable operation can take $\Sigma c_1$ to $\Sigma(c_1^{k+1})$. $\endgroup$
    – Eric Wofsey
    Commented Dec 17, 2012 at 20:56

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .