[Edited Jan 23, 2021]
Let $D^n$ be the $n$-dimensional unit radius disk in euclidean $\mathbb{R}^n$.
Larry Guth's Sponge Problem asks: Does there exist a constant $\epsilon=\epsilon_n$ such that every open subset $U\subset \mathbb{R}^n$ satisfying $vol(U)< \epsilon_n$ admits an expanding embedding $f: U\hookrightarrow D^n$?
Recall $f$ is expanding embedding (EE) iff $f$ is a continuous embedding and the symmetric matrix ${}^tDf\cdot Df$ has all eigenvalues $\geq 1$. This last local condition holds iff $||D_xf(v)||\geq ||v||$ for every $x\in U$ and tangent vector $v\in T_x U$. Equivalently $f$ is (EE) iff $f$ increases the induced path length of all curves in $U$, where the path length is defined by continuous paths contained in the image $f(U)$.
My previous best estimate $\epsilon_n^*$ for $\epsilon_n$ comes from obvious example of two disks $D_1, D_2$ of radius $1/2$ kissing, which disks $U':=D_1\cup D_2$ cannot be properly expanded embedded into $D$. (The two kissing disks barely embed into $D$, even though they occupy only $1/2^{n-1}$ a fraction of the volume of $D^n$). Similar examples arise from "truncated" Apollonian packings, e.g. the first figures of Sarnak's MAA address http://web.math.princeton.edu/sarnak/InternalApollonianPackings09.pdf
Possible counterexamples arise among low density disk packings which are strongly jammed, as investigated by S. Torquato and F.H. Stillinger, e.g. consider the following figures which are reproduced from Figure 2, https://arxiv.org/abs/cond-mat/0112319 . In the figure 2 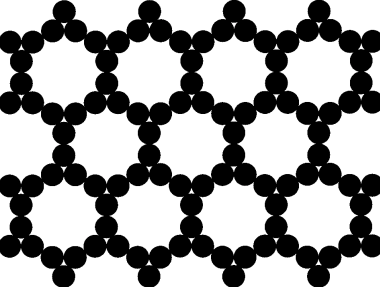
we see a packing which is rigidly jammed, and which cannot be anywhere locally expanded embedded into a smaller disk (or rectangle in this case).
The construction of such rigidly jammed packings which have arbitrarily small density is described in https://www.degruyter.com/view/journals/zkri/220/7/article-p657.xml
For example, consider the figure 2 in Fischer, reproduced below 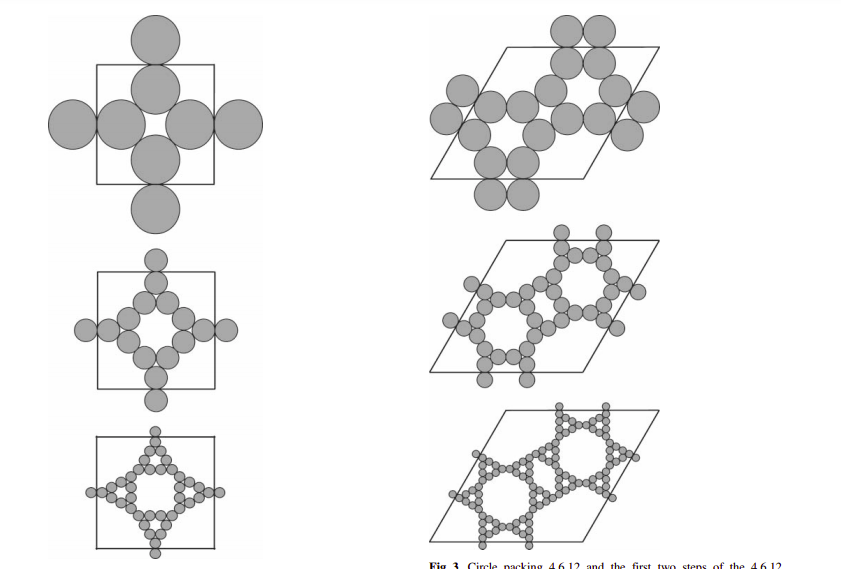 The rigidity of these packings is a form of incompressibility which can possibly lead to counterexamples to the Sponge Problem.
The rigidity of these packings is a form of incompressibility which can possibly lead to counterexamples to the Sponge Problem.
We emphasize that the packings in the above figures cannot be compressed (contained within a smaller boundary) via a global expanding-embedding. For example, there are no volume preserving rigid motions which preserve tangencies between all the disks, and which compresses the packing into a smaller volume.
Question: Can anybody provide further update on the status of L.Guth's Sponge Problem? Are better approximations $\epsilon^*$ known, or candidate open sets $U\subset \mathbb{R}^n$ with $vol(U)<\epsilon_n^*$ which cannot be expanded embedded into $D$?
