Let $S,T$ be abstract simplicial complexes.
Is there a (unique) abstract simplicial complex that gives me the most of what is in common with $S$ and $T$?
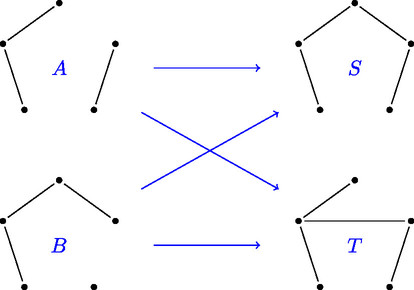
I'm thinking of this as an "intersection," or "refinement" of the two complexes, maybe a "core" that has the most properties of both. I could take the trivial simplicial complex on the smaller number of vertices of the two, but this misses larger simplices. Better, I could take the largest simplicial complex $A$ smaller than both $S$ and $T$ for which there are injective simplicial maps $A\to S$ and $A\to T$, sort of as here. But this is not unique:
$A$ and $B$ can't be compared by set inclusion. I can't think of any "good" construction of such an intersection, I can't capture all the similarities. For example, there is a simplicial map $A\to B$ that is injective on 1-simplices, but no such map $B\to A$, so $A$ could be considered "smaller" than $B$. But this seems roundabout. I'd be glad for any insight into why such a construction can / cannot work.
Maybe I should look in a different category or use a different construction? Move to homology? My larger goal is to build a constructible sheaf valued in simplicial complexes. I know what its value should be at every stalk (some simplicial complex), but I'm not sure how I should be defining the sheaf on larger open sets that intersect several strata.