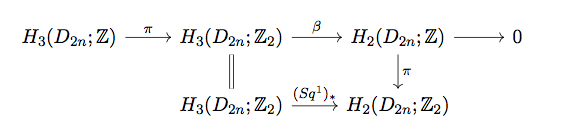9
$\begingroup$

As the tile suggests, I'm interested in computing the action of the Steenrod Algebra on $H^*(D_{2n};\mathbb{Z}_2)$, for $n=0 \pmod{4}$. Let us start with some definitions/facts: $$D_{2n} = \langle x,y \mid x^n=y^2=1, xyx=y \rangle$$

$$ H^*(D_{2n};\mathbb{Z}_2)\cong \mathbb{Z}_2[x,y,w]/(xy+x^2)$$ where $|x|=|y|=1$ and $|w|=2$. $w \in H^2(D_{2n};\mathbb{Z}_2)$ is the class represented by the standard representation of $D_{2n}\to O(2)$ as the group of symmetries of the regular $n$-gon.

I need to determine $Sq^1(w)$.

Using the Bockstein l.e.s. for $Z \to Z \to Z_2$ and $Z_2 \to Z_4 \to Z_2$ I have the following commutative diagram:

enter image description here

Since on the paper I'm reading it's claimed that $H_2(D_{2n};\mathbb{Z})\cong \mathbb{Z}_2\langle w_* \rangle$ and that $H^3(D_{2n};\mathbb{Z}_2)\cong \mathbb{Z}_2\langle x^3,y^3,wx,wy\rangle$, my intuition tells me that $Sq^1(w)=wx+wy$. This is just an intuition and I have no idea how to prove it. I think much could be said if one knows the generators of $H_3(D_{2n};\mathbb{Z})\cong \mathbb{Z}_2\oplus \mathbb{Z}_2 \oplus \mathbb{Z}_n$. I tried looking on the reference given by the paper . It's mentioned to look at page 38-39 for a proof of all the facts I mentioned above, but I'm not able to see anything useful.

So here is my question again, how to compute $Sq^1(w) \in H^3(D_{2n};\mathbb{Z}_2)$?

Thanks for any help and I take this opportunity to wish you a merry Christmas and a happy new year!

EDIT: I found out that for group representations $\rho \colon G \to GL_n$ we have that $w_1(\rho)=\det(\rho)$ See here. Using this it's easy to see that $w_1(\rho)=y$, where $\rho$ is the representation used for defining $w$. Now using Charles Rezk's comment, one sees that $Sq^1(w)=w\cup y$

Improve this question
$\endgroup$
4
  • 4
    $\begingroup$ So $w$ is the pullback of the second Stiefel-Whitney class $w_2\in H^2(BO(2),Z_2)$? Then the "Wu formula" computes Steenrod squares in $H^*(BO(2))$. I believe in this case $Sq^1(w_2)=w_1w_2+w_3=w_1w_2$ (since $w_3=0$ in $O(2)$). You didn't characterize $x$ and $y$ enough so that I can say what $w_1$ pulls back to. $\endgroup$
    – Charles Rezk
    Commented Dec 24, 2016 at 15:34
  • $\begingroup$ @CharlesRezk Using the iso $H^1 \cong \hom(H_1,\mathbb{Z}_2)$, we can define $x,y$ as the dual of the respective generators of the abelianization of $D_{2n}$. If I'm following your reasoning, everything boils down to prove what is the image of the first SW class of the representation $\rho \colon D_{2n} \to O(2)$ which defines $w$? $\endgroup$
    – Riccardo
    Commented Dec 24, 2016 at 16:20
  • $\begingroup$ Yes yup yes yup $\endgroup$
    – Charles Rezk
    Commented Dec 24, 2016 at 16:25
  • 1
    $\begingroup$ @CharlesRezk I think I managed to prove everything. Thanks a lot. Do you mind making your first comment the answer so I can close this question? $\endgroup$
    – Riccardo
    Commented Dec 24, 2016 at 19:27

0

Reset to default

You must log in to answer this question.