I will give partial answer in the following particular case:
Assume you have only one closed space curve $\gamma(t)=(f_{1}(t),f_{2}(t),f_{3}(t)) \in C^{3}([0,1])$. Let $\tau$ be its torsion and let $k$ be its curvature. Assume that $k$ never vanishes, and $\tau$ is not identically zero on any subinterval of $[0,1]$. Assume also that the plane curve $(f_{1}(t),f_{2}(t))$ is convex. Let $n(\tau)$ be the number of sign changes of torsion $\tau$.
Theorem
If $n(\tau)\leq 4$ then
$$
conv(\gamma)=\cup_{a,b\in \gamma}\{a\lambda+b(1-\lambda):0\leq \lambda \leq 1\}.
$$
Remark: It is known fact that $n(\gamma)\geq 4$, therefore in the theorem one should think that $n(\gamma)=4$.The proof can be extracted from this paper, see Section 3 and 4. In fact, what you can actually extract is that
\begin{align*}
\partial[conv(\gamma)]=\cup_{a,b\in \gamma}\{a\lambda+b(1-\lambda):0\leq \lambda \leq 1\}.
\end{align*}
Here $\partial \Omega$ denotes boundary of the domain $\Omega$. Then it is not hard to show that this implies the theorem.
I can sketch the idea: We are going to look to the boundary of $conv(\gamma)$. You can think that it has two boundaries: upper one and lower one. What does it mean? The upper one is a graph of a minimal concave function $B^{min}(x,y)$ defined in the plane domain bounded by $(f_{1}(t),f_{2}(t))$, and boundary of the graph $B$ is $\gamma$ i.e., $B^{min}(f_{1}(t),f_{2}(t))=f_{3}(t)$. Similarly the lower boundary is maximal convex function $B^{max}$ graph of which is attached to $\gamma$.
Now by Caratheodory's theorem it is enough to show that the graph of $B^{min}$ does not contain domains of linearity (triangles!), and this will mean that it consists only by chords $\{a\lambda +b(1-\lambda), 0\leq \lambda\leq 1\}$ for some $a,b\in \gamma$.
Suppose it contains triangles. Then let us consider any side of the triangle. Let it be the chord with endpoints $\gamma(a)$ and $\gamma(b)$ for some $a,b\in [0,1]$.
Notice that this chord will be tangent to the curve $\gamma$ (this is not a difficult observation). In other words this means that there exists a plane containing the chord $[\gamma(a),\gamma(b)]$ and such that the curve $\gamma$ lies to one side of the plane. It is the same as to say that
$$
\det(\gamma’(a), \gamma’(b), \gamma(b)-\gamma(a))=0 \quad (1)
$$
Now if you play with this equation for a while, you will see that the torsion must change the sign from + to - on both of the side of its chord (moving counterclockwise). Since triangle has 3 sides in total you will have 3 times changing of signs of $\tau$ from + to - and this implies that $n(\tau)\geq 6$.
In other words every time whenever you draw a such chord (bitangent line) torsion changes sign on its sides. And this finishes the proof.
Now the question remains: what happens in theorem if we assume that $n(\tau)>4$. Of course theorem is not true anymore, the quantity $n(\tau)$ does not give you any information about the structure of the graph $B^{min}$. There is another object (smooth transformation of torsion) which we call force function which gives the answer: there exists a source such that coming force have full tails, but this is different story (some language was developed here)
Roughly peaking at the point where the torsion changes sign from + to -, you can (locally) construct tangent chords (a cup) $[\gamma(a),\gamma(b)]$. Now take one of them an try to extend them through out the curve (I mean foliate the domain, bounded by the curve $(f_{1}(t),f_{2}(t))$, by chords so that they will not intersect each other but will fill out the domain).
Intuitively it means that you take this closed space curve (say closed wire), drop it on the ground, and try to roll it, so that in the beginning ground touches the wire exactly at one point (where torsion changes sign) and then it can be completely rolled over the ground (so that wire will never touch the ground at triangle) and eventually it will finish touching again at only one point (and again torsion will change the sing at that final point as well). This is possible if and only if you can extend equation (1) say by implicit function theorem throughout the curve $\gamma$, and there is one simple answer to the question when is it possible, it is possible if and only if there exists a force function with full tails. And this should be true for both: for $B^{min}$ and for $B^{max}$.
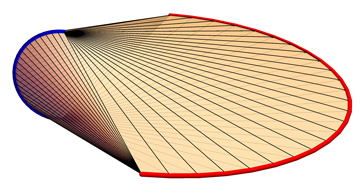
Update: To illustrate what I wrote above I am attaching this picture. On this picture torsion of the curve changes sign $n(\tau)= 8$ times (4 times from + to - minus), and on each point where it changes sign from + to - you see the cup : family of chords coming from there. And you see that there are two triangular domains (domains of linearity: places without any thread): one close to you and one from another side which is not completely exposed on the picture. By the way this is how the upper boundary of the convex hull ($B^{min}$) look like for this space curve (closed wire).