10
$\begingroup$

The lattice $\mathbb{Z}^n$ has an essentially unique (up to permutation) minimal periodic coloring for all $n$, namely the "checkerboard" 2-coloring. Here a coloring of a lattice $L$ is a coloring of the graph $G = (V,E)$ with $V = L$ and $(x,y) \in E$ if $x$ and $y$ differ by a reduced basis element. (NB. I am not quite sure that this graph is the proper one to consider in general, so comments on this would also be nice.)

The root lattice $A_n$ has many minimal periodic colorings if $n+1$ is not prime (I have sketched this here, and some motivation is in the last post in that series); if $n+1$ is prime, then it has essentially one $n+1$-coloring. Two minimal periodic colorings for $A_3$ are shown below (for convenience, compare the tops of the figures):

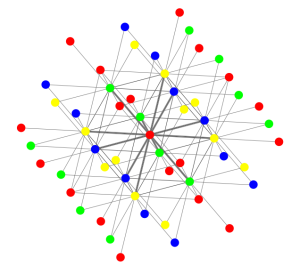alt text The generic ("cyclic") coloring.

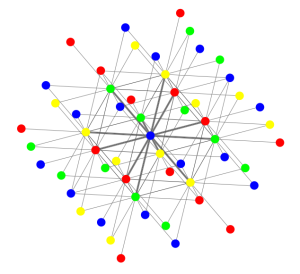alt text A nontrivial example.

The lattices $D_n$ are also trivially 2-colored.

So: are there other lattices that admit more than one minimal periodic coloring? I'd be especially interested to know if $E_8$ or the Leech lattice do.

(A related question: does every minimal periodic coloring of $A_n$ arise from a group of order $n+1$?)

Improve this question
$\endgroup$

1 Answer 1

Reset to default
4
$\begingroup$

When you say "reduced basis", I assume you mean that two lattice points are connected in your graph if the distance between them is the minimum distance of the lattice (i.e. the shortest distance between any two lattice points).

There is a simple way to generate a $24$ colouring of $E_8$ using the $8$ colouring you have for $A_8$. It so happens that $E_8$ is isomorphic to the union of 3 translations of $A_8$,

$E_8 = A_8 + (A_8 + g) + (A_8 + 2g)$

where $g = \left( \tfrac{8}{3}, -\left(\tfrac{1}{3}\right)^8 \right)$. That is, $g$ is a vector with one $\tfrac{8}{3}$ and eight $-\tfrac{1}{3}$'s. See Martinet, Perfect Lattices in Euclidean Spaces. So you just need 3 independently coloured $A_8$'s. The resultant colouring will be periodic if the colourings for $A_8$ are periodic. It's likely that this is not the best colouring possible for $E_8$.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Robby--Even if this isn't a minimal coloring, it is still interesting. Thanks for your answer. $\endgroup$
    – Steve Huntsman
    Commented Apr 27, 2010 at 13:46
  • $\begingroup$ Dear Robby, have you looked at this one? mathoverflow.net/questions/22777 I suggested you as someone likely to know. Will $\endgroup$
    – Will Jagy
    Commented Apr 28, 2010 at 18:58

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .