Reconstructing a morphism of exact triangles in the homotopy cat. of a dg-cat. using a "functorial cone map"

Question

I set this problem in the framework of (pretriangulated) dg-categories; everything can probably be translated in the world of stable $(\infty,1)$-categories.

Let $\mathcal A$ be a pretriangulated dg-category. It is known that the homotopy category $H^0(\mathcal A)$ is triangulated, with exact triangles coming from the "pre-triangles" in $\mathcal A$. Assume we have a diagram in $\mathcal A$:

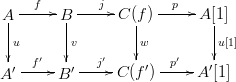
_{(source: presheaf.com)}
,

where the rows are pretriangles in $\mathcal A$, the vertical arrows are closed and of degree $0$, and everything is commutative in $H^0(\mathcal A)$. This diagram, in other words, induces a morphism of exact triangles in $H^0(\mathcal A)$.

Now, let $h : A \to B'$ be a degree $-1$ morphism such that \begin{equation} dh = vf - f'u, \end{equation} which exists by hypothesis. In the dg-category $\mathcal A$, there is a canonical (closed, degree $0$) morphism $C(u,v,h) : C(f) \to C(f')$ induced (functorially!) between the cones. This morphism also makes the diagram

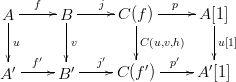
_{(source: presheaf.com)}

commute in $H^0(\mathcal A)$. My question is the following: is it true or false, in general, that $[w] = [C(u,v,h)]$ as morphisms in $H^0(\mathcal A)$? Actually, there are some subtleties. Better said: can I choose $u': A \to A'$, $v': B \to B'$ closed of degree $0$ such that $[u]=[u']$, $[v]=[v']$, and $h' : A \to B'$ closed of degree $-1$ with $dh' = v'f - f'u'$, such that $[w] = [C(u',v',h')]$? I believe the answer of the above question is false, but perhaps it is true with some hypothesis on $\mathcal A$?

Answer 1

The following example comes from Neeman's ``Some new axioms\dots" (J. Algebra, 1991). A triangle is contractible if it is a direct sum of (translations of) triangles of the form $0\rightarrow X\rightarrow X \rightarrow 0$. Contractible triangles are exact, but exact triangles are seldom contractible. Assume that $X\rightarrow Y\rightarrow Z\rightarrow\Sigma X$ is not a contractible exact triangle. Then $$\begin{array}{ccccccc} X&\stackrel{f}\rightarrow&Y&\stackrel{i}\rightarrow&Z&\stackrel{q}\rightarrow&\Sigma X\\ {\scriptstyle 0}\downarrow&&{\scriptstyle 0}\downarrow&&{\scriptstyle q}\downarrow&&{\scriptstyle 0}\downarrow\\ Y&\stackrel{i}\rightarrow&Z&\stackrel{q}\rightarrow&\Sigma X&\stackrel{-\Sigma f}\rightarrow &\Sigma Y \end{array}$$ is a morphism of triangles and $q$ cannot be obtained in the way you described. Otherwise, $h$ would be simply a morphism $h\colon \Sigma X\rightarrow Z$ and, up to sign, $qhq=q$ necessarily. This leads to contractibility of the initial exact triangle.

This argument is very general, so we rather see it `in action' in $D(\mathbb Z)$. We can take the exact triangle associated to the short exact sequence $\mathbb Z/2\hookrightarrow \mathbb Z/4\twoheadrightarrow \mathbb Z/2$

$$\begin{array}{ccccccc} \mathbb Z/2&\stackrel{f}\rightarrow&\mathbb Z/4&\stackrel{i}\rightarrow&\mathbb Z/2&\stackrel{q}\rightarrow&\Sigma \mathbb Z/2\\ {\scriptstyle 0}\downarrow&&{\scriptstyle 0}\downarrow&&{\scriptstyle q}\downarrow&&{\scriptstyle 0}\downarrow\\ \mathbb Z/4&\stackrel{i}\rightarrow&\mathbb Z/2&\stackrel{q}\rightarrow&\Sigma \mathbb Z/2&\stackrel{-\Sigma f}\rightarrow &\Sigma \mathbb Z/4 \end{array}$$ Here $q\in \operatorname{Ext}_{\mathbb Z}^1(\mathbb Z/2,\mathbb Z/2)\cong \mathbb Z/2$ is the generator, but there are not degree $-1$ maps $\mathbb Z/2\rightarrow \mathbb Z/2$, i.e. the only map $\Sigma\mathbb Z/2\rightarrow\mathbb Z/2$ is the trivial map. Therefore, the standard completion of the first commutative square would be the trivial one, not the former.

The last explicit counterexample turned out to be more obvious than I remembered.