0
$\begingroup$

Fix a field extension $k\subseteq K$ (assume that the fields have characteristic $0$) and consider the two following definitions:

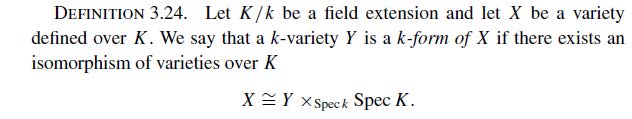
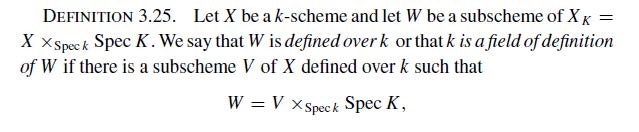
enter image description here enter image description here

Now let's restrict our attention to a closed subscheme $X\subseteq\mathbb P^n_K$ (i.e. embedded projective variety), I have basically two questions:

  • Definition $3.24$ in other terms means that I can find some homogeneous polynomials with coefficients in $k$, whose common zeros set is isomorphic to $X$. Now I don't understand what is the practical meaning of definition $3.25$. I'd like a description in terms of common zeros of polynomials (remember: only in the case of embedded projective varieties).
  • In definition $3.25$ it is very strange the fact that one requires the equality $$W= V\times_{\operatorname{Spec}k} \operatorname{Spec} K$$ but what happens if one requires the equality up to isomorphism? Namely: $$W\cong V\times_{\operatorname{Spec}k} \operatorname{Spec} K$$ (It seems that in our case the two definitions coincide.)
Improve this question
$\endgroup$
4
  • $\begingroup$ To understand what can go wrong, consider the case of a complex non-real line inside the complex plane. $\endgroup$
    – Jérôme Poineau
    Commented May 15, 2014 at 7:56
  • 1
    $\begingroup$ Two subschemes of a given scheme may, or may not, be equal; "isomorphic subschemes" does not make much sense. $\endgroup$
    – abx
    Commented May 15, 2014 at 8:55
  • $\begingroup$ @abx Yes you are right, this is the point. Two schemes $Y$ and $Y'$ are equal as subschemes of $X$ if they are isomorphic and this isomorphism commutes with two embeddings in $X$ $\endgroup$
    – Dubious
    Commented May 15, 2014 at 9:15
  • $\begingroup$ @abx: I think it depends on how you define a subscheme. Whenever there is a sheaf $I$ with an injective map $I \to O_X$, I'd like to think of it as an ideal sheaf, regardless of whether it is in fact set-theoretically a subsheaf of $O_X$. If you used the functor of points approach, you might say that a subscheme is a special type of subfunctor, but I'm not sure there is much to gain from that point of view. In the end, isn't it just better to define a subscheme as some $Y$ together with an embedding into $X$? $\endgroup$
    – bananastack
    Commented May 15, 2014 at 14:46

1 Answer 1

Reset to default
1
$\begingroup$

presumably the reason there is an equality in 3.25 instead of an isomorphism is that you don't want $V_K$ to be abstractly isomorphic to $W$, but you want them to be equivalent subschemes of $X_K$ (ie isomorphic in the category of pairs (scheme, embedding)).

At least that's how I would interpret it, but I might be wrong. Also, take $X = P^N$. Saying that $W \subset X_K$ is defined over $k$ means precisely that you can find equations cutting out $X_K$ with coefficients in at most $k$.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged .