3
$\begingroup$

Browsing an old technical studies pupil's school book, I have found the description of a method to place at equal distance $N$ points on the circumference of a circle. I am looking for a proof of this method, but it doesn't look obvious to me... and I haven't been able to find one on the internet.

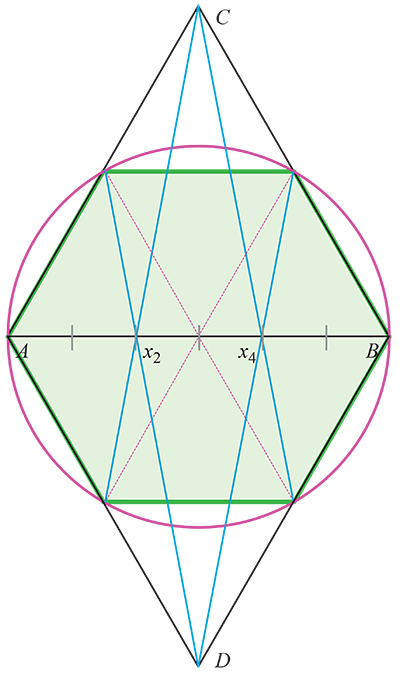
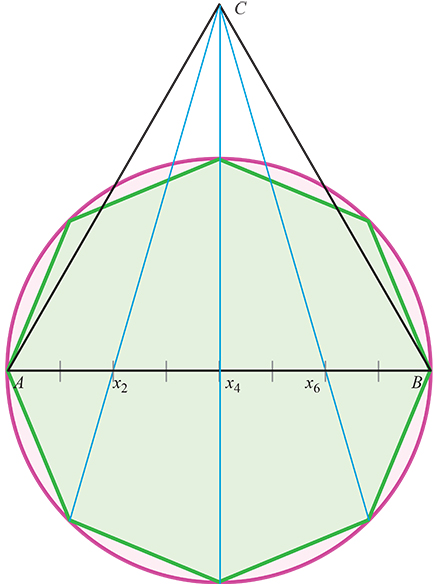
The algorithm: draw a circle of center $O$ and diameter $AB$, which is partitioned in $N$ parts $A=X_0$ and $B=X_N$ (using a ruler or any other method). Draw the equilateral triangles $ABC$ and $ABD$. Then the book claims that the intersections $X'_i$ of the circle and lines $CX_{2i}$ outside of segment $CX_{2i}$, $i\leq N/2$ and same with $DX_{N-2i}$, define an equipartition of the circle circumference.

I have tried to prove it starting with the dot product of $OX'_i$ and $OX'_{i+1}$ and trying to show it's not dependent on $i$, but have not succeeded.

Is there a classical proof? Ideas?

Improve this question
$\endgroup$
7
  • 5
    $\begingroup$ This would imply constructibility of a heptagon by compass and straightedge, a contradiction. I think the method produces just a good approximation. $\endgroup$
    – The Masked Avenger
    Commented Dec 23, 2013 at 19:21
  • $\begingroup$ @TheMaskedAvenger --- I presume the construction of the OP allows one to use a compass with a marked ruler (not just an unmarked straightedge); then heptagons can be constructed (and I would think all regular polygons as well) --- en.wikipedia.org/wiki/Heptagon $\endgroup$
    – Carlo Beenakker
    Commented Dec 23, 2013 at 19:37
  • $\begingroup$ The marked ruler is needed for dividing AB into 7 equal segments, I believe. $\endgroup$
    – Joce
    Commented Dec 23, 2013 at 20:21
  • 1
    $\begingroup$ Except I can I can mark off with a compass 7 equal length segments on another line, then use parallel lines to divide the diameter correspondingly. I maintain that the construction described in the question is equivalent to a compass and straightedge construction. $\endgroup$
    – The Masked Avenger
    Commented Dec 23, 2013 at 20:34
  • 1
    $\begingroup$ More precisely, my reading of the described construction (which is supported by Joseph's picture) is so equivalent... . $\endgroup$
    – The Masked Avenger
    Commented Dec 23, 2013 at 20:39

2 Answers 2

Reset to default
2
$\begingroup$

Not an answer; just an illustration of the construction for $n=6$ (now updated to show $ABD$ as well):
     CircPart
(Added.) Here is the (top-half of the) construction for $n=8$:
     CircPart8

Improve this answer
$\endgroup$
3
  • $\begingroup$ What about the point $D$ from the algorithm? I think the algorithm just does not work. $\endgroup$
    – Wlodek Kuperberg
    Commented Dec 23, 2013 at 22:58
  • $\begingroup$ I tried to add this point D as described by the OP, but indeed, I don't see how this would give 5 or 6 equidistant points --- ilorentz.org/beenakker/MO/circle_triangle.jpg $\endgroup$
    – Carlo Beenakker
    Commented Dec 23, 2013 at 23:23
  • $\begingroup$ I think it "works" for $n$ even. I added the (green) hexagon for $n=6$, and now the octagon for $n=8$. $\endgroup$
    – Joseph O'Rourke
    Commented Dec 24, 2013 at 1:50
1
$\begingroup$

Not quite an answer, but food for thought.

Consider a curve with N (let's say distinct) distinguished points on it. Call two such C and D PR (or C PR D, for projectively related) if there is a point O and N lines such that OX_iY_i are on one of the N lines, where the X's are distinct from the Y's and O and are the distinguished points of C respectively D. I should think C PR D implies the N points of C are constructible iff the N points of D are constructible. (Some tweaking of PR may be needed to handle translations and rigid rotations of curves.) Further some nice version of the relation PR may be equivalent to constructibility.

Anyway, this is the notion behind my comments saying that the described construction is an approximation.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged .