21
$\begingroup$

The following question resisted attacks at Math SE, so I thought I would try posting it here.

Is the following conjecture true or false:

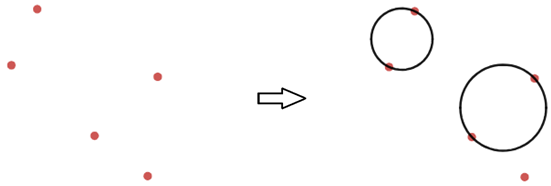
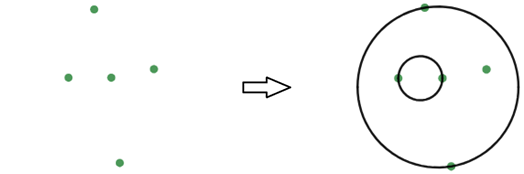
Given any five coplanar points, we can always draw at least one pair of non-intersecting circles coplanar with the points, such that two of the given points are diameter endpoints of one circle, and another two of the given points are diameter endpoints of the other circle.
Tangent circles are considered to be non-intersecting. Coincident circles are considered to be intersecting.

Example:

Example 1

Another example:

Example 2

I cannot find a counter-example, nor can I prove the conjecture.

Remarks:

I made a generator of five (pseudo)random points.

If, instead, we were given four points, the conjecture would not be true: for example, if the four points were the vertices of an equilateral triangle plus the centre, then we could not draw a pair of non-intersecting circles.

The generalized conjecture with $2n+1$ points and $n$ circles is not true.

I have asked, and answered, a similar question, which might provide ideas for this question.

Context:

I was thinking about this question about random points in a disk. Staring at various sets of five points, I came up with this conjecture.

Improve this question
$\endgroup$
3
  • 3
    $\begingroup$ Note that since we permit tangent circles, any counterexample will be robust to epsilon perturbations, so we can safely assume any "generic" conditions we like. $\endgroup$
    – RavenclawPrefect
    Commented Nov 28, 2023 at 8:42
  • 3
    $\begingroup$ If five points are a counterexample, then any subset of four points are a counterexample to the 4 points and 2 circles version of the problem. So perhaps it is worth better understanding the condition on 4 points which makes them a counterexample. $\endgroup$
    – Jack Huizenga
    Commented Nov 28, 2023 at 22:16
  • $\begingroup$ @JackHuizenga I think to understand the condition it can be helpful to read the answer from the MSE thread linked by OP. But although I did this, I think that some pieces of the puzzle are still missed. $\endgroup$
    – Alex Ravsky
    Commented Nov 29, 2023 at 2:43

1 Answer 1

Reset to default
24
$\begingroup$

A counterexample is given by the following five points: $$(0,0),(1,0), \Big(-\frac{64867}{77629},\frac{3389}{60094}\Big), \Big(\frac{5981}{56176},\frac{32211}{34172}\Big), \Big(\frac{5925}{117812},-\frac{103221}{116516}\Big).$$

Calculations and the way this example was found are presented in this pdf image of a Mathematica notebook. In particular, it is noted there that a certain "defect" of this example, which makes it a counterexample, is very small, about $3\times10^{-6}$. So, one can have here two "very slightly intersecting" circles, their intersection certainly invisible to the eye if both circles are fully shown.

Improve this answer
$\endgroup$
9
  • 1
    $\begingroup$ @Dan : I have addressed your comments. $\endgroup$
    – Iosif Pinelis
    Commented Nov 29, 2023 at 3:58
  • $\begingroup$ Bravo! Here is the desmos graph I used to check all fifteen cases. $\endgroup$
    – Dan
    Commented Nov 29, 2023 at 4:00
  • 7
    $\begingroup$ One can even choose $2$ points on the $x$-axis and $2$ points on the $y$-axis. An example in particularly small numbers is $(-330, 0)$, $(330, 0)$, $(0, -333)$, $(0, 329)$, $(26, 25)$. $\endgroup$
    – Peter Mueller
    Commented Dec 2, 2023 at 8:57
  • 5
    $\begingroup$ ... and another one is $(-236, 0)$, $(236, 0)$, $(0, -235)$, $(0, 237)$, $(22, 1)$. $\endgroup$
    – Peter Mueller
    Commented Dec 2, 2023 at 15:02
  • 2
    $\begingroup$ I got the first one from a numerical optimization and then rationalizing. The second one from a direct search of integer points $(-a,0), (0,b), (0,-c), (0,d), (e,f)$ where $a,b,c,d$ are positive, $|a-b|<6$, $|c-d|<6$ and $|e|,|f| < a/5$. $\endgroup$
    – Peter Mueller
    Commented Dec 2, 2023 at 15:19

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .