For $\mu$-a.e. $x \in X$, the sequence $(f_n(x, \cdot))_n$ is Cauchy in $L^1 (Y)$. Then $(f_n)$ is Cauchy in $L^1 (X \times Y)$

Question

Below we use Bochner measurability and Bochner integral. Let

• $(X, \mathcal A, \mu)$ and $(Y, \mathcal B, \nu)$ be complete $\sigma$-finite measure spaces,
• $(E, | \cdot |)$ a Banach space,
• $S (X)$ the space of $\mu$-simple functions from $X$ to $E$,
• $L^0 (X)$ the space of $\mu$-measurable functions from $X$ to $E$,
• $L^1 (X)$ the space of $\mu$-integrable functions from $X$ to $E$,
• $\mathcal C :=\mathcal A \otimes \mathcal B$ the product $\sigma$-algebra of $\mathcal A$ and $\mathcal B$,
• $\lambda := \mu \otimes \nu$ the product measure of $\mu$ and $\nu$.

Theorem 4.4.1 at page 98 of Martin Väth's monograph Ideal Spaces suggests that

Theorem Let $\mu, \nu$ be finite measures, i.e., $\mu(X) + \nu (Y)< \infty$. Let $f_n:X \times Y \to E$ be $\lambda$-simple for all $n \in \mathbb N$. Assume that for $\mu$-a.e. $x \in X$, the sequence $(f_n(x, \cdot))_n$ is a Cauchy sequence in $L^1 (Y)$. Then $f_n$ is a Cauchy sequence in $L^1 (X \times Y)$.

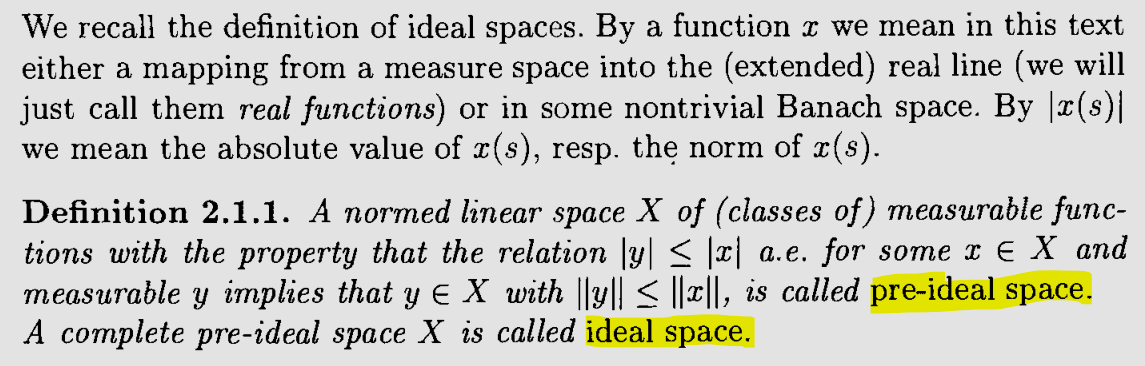
The author's proof is in the context of pre-ideal spaces, which is unfamiliar to me. Here I work with the usual Bochner spaces.

Could you elaborate on how to prove above Theorem?

---

Below I add relevant paragraph in the book.

Answer 1

Väth only claims that the sequence $y_n$ is Cauchy in the space of measurable functions with the topology (or rather, uniformity) of convergence in measure which, for finite measures, is given by the complete (semi- or pseudo-) metric $d(y,z)=\int\min\{|y-z|,1\} d\mu$. A sequence $y_n$ converges to $y$ if and only if $\mu(|y_n-y|>\varepsilon)\to 0$ for all $\varepsilon>0$ if and only if every subsequence $n(k)$ has a further subsequence such that $y_{n(k(\ell))}\to y$ $\mu$-a.s.