This is actually a poorly camouflaged attempt to use the answers to When is the opposite of the category of algebras of a Lawvere theory extensive? (all very interesting) for the purposes of my Equational theories determined by "identities without variables".
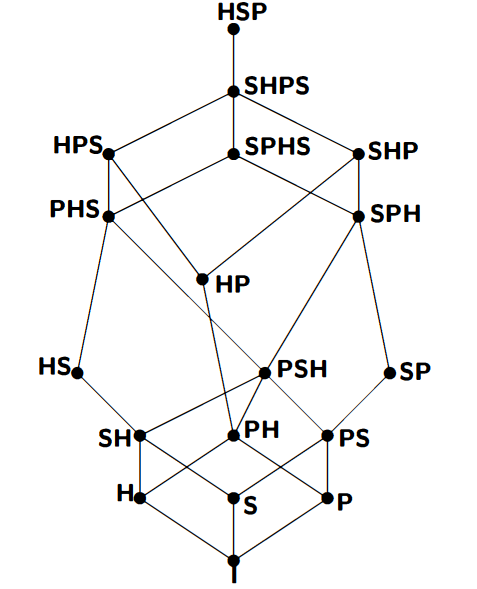
I learned, again somewhere here on MO, but unfortunately cannot remember where, that Pigozzi in 1972 computed all possible combinations of taking homomorphic images, subalgebras and products in a variety:
Pigozzi, D., On some operations on classes of algebras, Algebra Univers. 2, 346-353 (1972).
Here is the diagram reproduced from Clifford Bergman's book
Fortunately of all these I am only interested in the following question:
Is there a nice characterization of the varieties of algebras $\mathscr V$ with the following property: for any class $\mathscr K$ of algebras such that $\mathscr V=\mathsf{HSP}(\mathscr K)$, one also has $\mathscr V=\mathsf{SPH}(\mathscr K)$?
Spelling out, this means that for any collection $\mathscr K$ of algebras that generate $\mathscr V$ as a variety, actually every algebra in $\mathscr V$ embeds in a product of quotients of members of $\mathscr K$.
My motivation is unfortunately very vague so far. I have suspicion that this has somehow to do with coextensivity of $\mathscr V$ from that question I linked, and that for the purposes of my other question I linked too it could be useful to first restrict it to the varieties with the above property.
Maybe few more words about why I have this suspicion. Looking at the duals, in sheaves over a space, which for me are the "best" extensive categories, you can obtain the étale space of each sheaf by gluing together a bunch of open subsets of your space. So you perform something dual of SPH rather than HSP, which would be taking instead open subsets of gluings of étale spaces of constant sheaves.