Let $A$ be an arbitrary symmetric matrix and $B$ be a random GUE matrix. I would like to know. Are there any results on the minimal eigenvalue distance between two distinct eigenvalues of $A+B$? I think it is easy to see they are almost surely all pairwise distinct. But can one say anything more refined without imposing too much on $A$?
-
$\begingroup$ the probability distribution of the spacing $s$ of subsequent eigenvalues will vanish as $s^2$ for small $s$, I don't think you can say more than that in general. $\endgroup$– Carlo BeenakkerCommented Jun 15, 2022 at 21:06
-
$\begingroup$ @CarloBeenakker can you make this result somehow precise, ideally with a reference? $\endgroup$– Guido LiCommented Jun 15, 2022 at 21:36
-
$\begingroup$ I have added a calculation, which gives an expected minimal distance that decays $\propto n^{-1/3}$ for large dimensionality $n$ of the matrix. $\endgroup$– Carlo BeenakkerCommented Jun 16, 2022 at 7:40
-
$\begingroup$ I think the problem is already nontrivial and interesting for $A\equiv 0$ --- I have asked at mathoverflow.net/q/424831/11260 $\endgroup$– Carlo BeenakkerCommented Jun 16, 2022 at 13:40
1 Answer
The distribution of the spacings of the eigenvalues of the GUE matrix $B$ is given by the Wigner surmise, $$p(s)=(32s^2/\pi^2) \exp \left(-4 s^2/\pi\right),$$ where the spacing $s>0$ of adjacent eigenvalues is measured is units of the mean spacing. The spacing distribution of the sum $A+B$, with $A$ a fixed symmetric matrix, is assumed to be well described by $p(s)$. There is some evidence for that, see Universality of the local spacing distribution in certain ensembles of hermitian Wigner matrices.
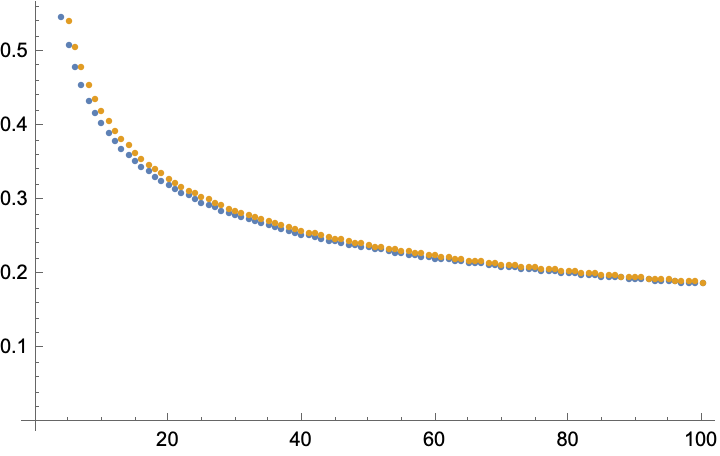
Eigenvalues that are not adjacent are only weakly correlated, I will assume that different spacings are independent. Then the expectation value of the minimal spacing $s_{\rm min}$ for $n\times n$ matrices is given by $$\mathbb{E}[s_{\rm min}]=\int_0^\infty [1-c(s)]^n\,ds,$$ with $c(s)=\int_0^s p(s')ds'$ the cumulative spacing distribution. This integral does not have a closed form expression, for large $n$ it tends to $$\mathbb{E}[s_{\rm min}]\rightarrow c n^{-1/3},\;\;c=\tfrac{1}{2}(\pi/6)^{2/3}\Gamma(1/3)=0.870.$$ Below is a plot up to $n=100$, gold is the integral, blue is the asymptotic.
It would be of interest to see if the $n^{-1/3}$ scaling survives an exact calculation in the GUE (not making the assumption of independent spacings). With the same assumption, in the GOE I would find $\mathbb{E}[s_{\rm min}]\rightarrow n^{-1/2}$.