1
$\begingroup$

I was reading an old article in IEEE Education magazine by Robbins and Fawcett titled "A Classroom Demonstration of Correlation, Convolution and the Superposition Integral" DOI: 10.1109/TE.1973.4320783.

The article is interesting but the definitions employed by author are somewhat non-conventional and this is not a typographical error.

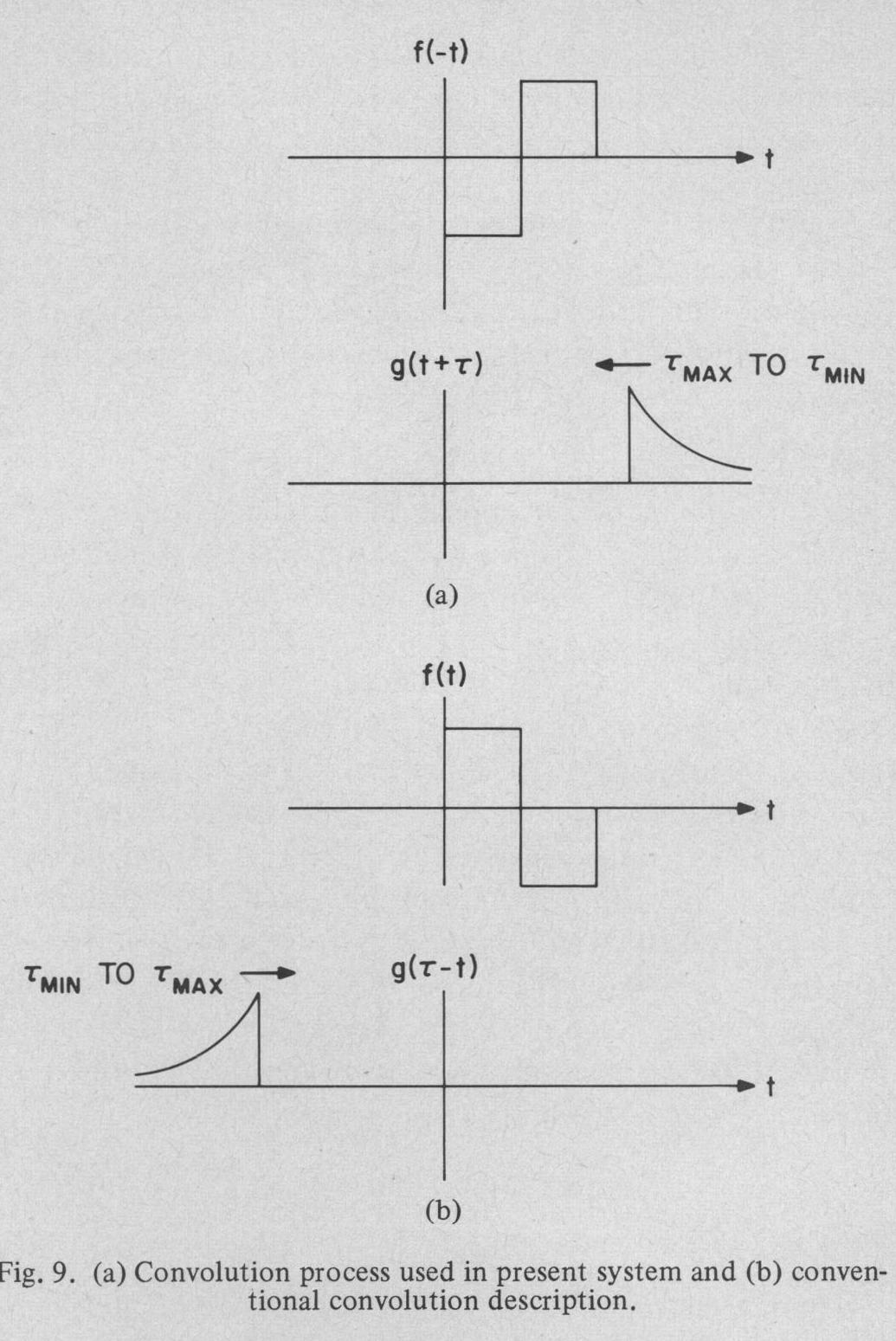
For example, the author defines the convolution of two periodic functions as:

$$\theta(\tau)=\frac{1}{T} \int_{0}^{T} f(t) g(t+\tau) d t$$

and the next lines says "or"

$$\theta(\tau)=\frac{1}{T} \int_{0}^{T} f(-t) g(t+\tau) d t.$$

enter image description here

I have not seen convolution integral with a positive sign but the second one is more conventional. The negative sign does the "folding" job in graphical convolution, so I thought the negative sign is necessary.

Similarly, correlation of two periodic functions is defined there as

$$\varphi(\tau)=\frac{1}{T} \int_{0}^{T} f(t) g(t-\tau) d t$$ and the pictorial representations seems more like what traditional convolution process is graphically shown.

How is this $\theta(\tau)=\frac{1}{T} \int_{0}^{T} f(t) g(t+\tau) d t$ representation, a convolution?

Improve this question
$\endgroup$
6
  • $\begingroup$ How do you know it is not a typographical error? As you say, the context suggests that it is. $\endgroup$
    – LSpice
    Commented May 16, 2022 at 23:06
  • 1
    $\begingroup$ The first equation and second are not generally equivalent... Makes a person think there's a typo. But, unsurprisingly, on the real line or on circles (for periodic things) the group is abelian, so "inverse" is an automorphism, and it doesn't matter toooo much. $\endgroup$
    – paul garrett
    Commented May 16, 2022 at 23:18
  • $\begingroup$ @Lspice, these equations appear in separate sections under different headings with examples. $\endgroup$
    – ACR
    Commented May 17, 2022 at 0:00
  • $\begingroup$ On second glance (assuming, as @paulgarrett mentioned, that we are working on an Abelian group), the definition of $\varphi(\tau)$ is closer to that of the first $\theta(-\tau)$ than it is to the second $\theta(\tau)$. @‍paulgarrett, I think the difference is significant even for Abelian groups: consider, for example, the convolution of $\chi : t \mapsto e^{2\pi i t}$ with itself according to the $\varphi$ definition (giving $0$), and the usual definition (giving $\chi$). $\endgroup$
    – LSpice
    Commented May 17, 2022 at 1:17
  • $\begingroup$ @LSpice, I agree, there are arguments in favor of this-and-that... depending on what ambient structure we want. But/and, then, for me, for clarity, I'd want to hear what the structural property is (your comment) first, and then see how to write a formula... that achieves it. E.g., for a group $G$ acting continuously on a TVS $V$, continuous compactly-supported functions naturally act by the integrated form. Ok. Then "convolution" of those functions $f,F$ should be such that $f\cdot (F\cdot v)=(f*F)\cdot v$ for all vectors $v$. From this we "discover" (two) formula(s) for convolution... :) $\endgroup$
    – paul garrett
    Commented May 17, 2022 at 1:22

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .