The construction of the free category on a quiver is standard in category theory.
Is there some $2$-dimensional analog of a quiver such that each $2$-quiver freely gives rise to a $2$-category? How about for $n>2$?
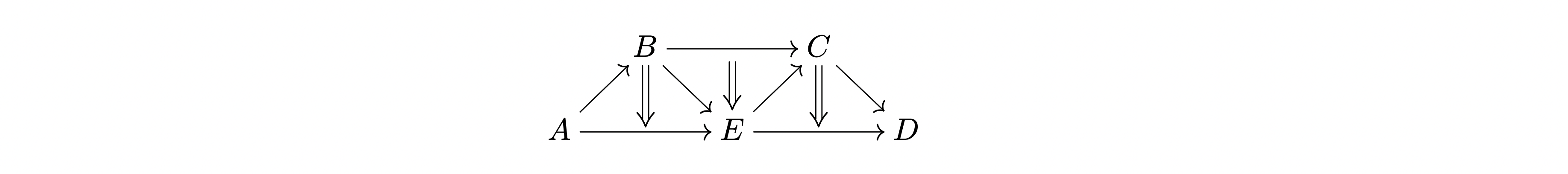
If a graph is a set of edges and a set of vertices, it seems reasonable that we could consider a set of edges, a set of vertices and and a set of 'faces', continuing on to 'volumes' and 'hypervolumes' etc., where we formalize it all using ordered pairs of lists. For example, the $2$-graph underlying the $2$-cell diagram
could be defined by setting the vertices $V$, edges $E$, and faces $F$ by
$$V=\{A,B,C,D,E\},$$
$$E=\{(A,B),(B,C),(C,D),(A,E),(E,D)\},$$
$$F=\Big\{\Big(\big((B,E),(A,B)\big),\big((A,E)\big)\Big),\Big(\big((B,C)\big),\big((E,C),(B,E)\big)\Big),\Big(\big((C,D),(E,C)\big),\big((E,D)\big)\Big)\Big\}.$$
The 'free $2$-category' on this $2$-graph would have $V$ for objects, lists of composable edges for $1$-cells, and lists of vertically composable faces for the $2$-cells in each category (where we add 'identity faces' and 'faces' corresponding to whiskerings with these identities).
I believe graphs are usually defined with so-called incidence functions instead of ordered pairs as above, but the incidence functions here are simply given by projection onto the first coordinate for sources and projection onto the second coordinate for targets.
Despite this, most attempts I'm familiar with to formalize the graph theory underlying pasting diagrams and such rely on geometric realizations and topological methods in dimension $2$, and Whitney stratifications in higher dimensions. It looks like CW complexes or abstract simplical complexes might be key words close to what I'm looking for. Has an approach similar to the above been developed anywhere in the literature? Any assistance is appreciated.