Claim. The thief $T$ can escape if $C$ is a circle, with a simple strategy of dribbling left and right each policeman at a time in such a way that he is left out of reach of the thief no matter what the future dribbles will be.
Proof.
The key insight is due to Pietro Majer: the thief can approach $C$ in such a way that its shadow $S$ (closest point) on $C$ always moves at speed faster than $1$.
Assume $C$ has radius $1$ and pick a number $r$ once and for all, with $1/2<r<1$.
At any time there are exactly two circles $C_1$, $C_2$ of radius $r$, tangent to $C$ and contaning $T$. The strategy of the the thief is to always run at speed $1$, alternating between these two ways:
$-$ either move right on $C_1$ towards $A$ (dragging $C_2$ and $B$ along)
$-$ or move left on $C_2$ towards $B$ (dragging $C_1$ and $A$ along).
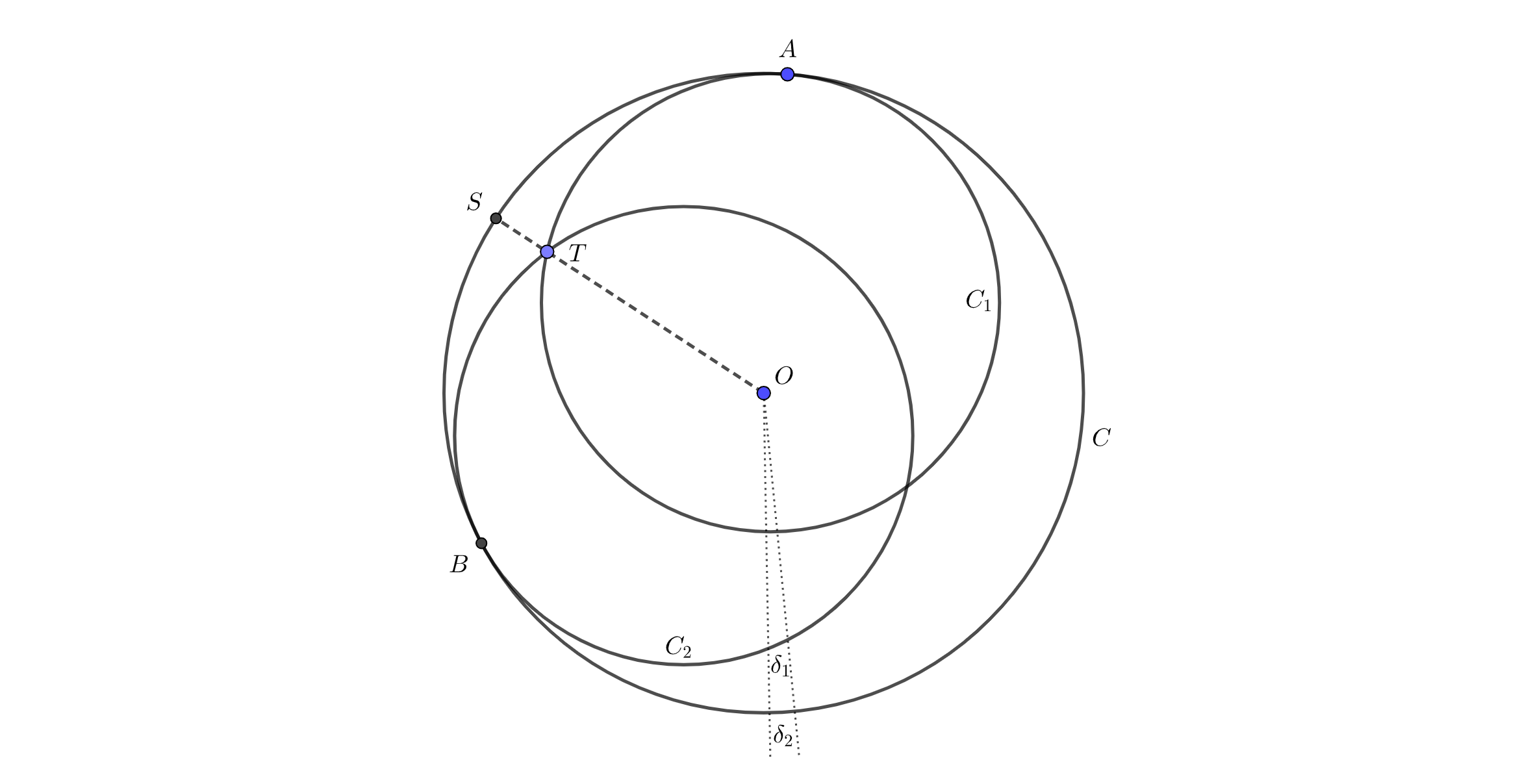
No matter how $T$ zigzags left and right, the symmetry between $C_1$ and $C_2$ with respect to $T$ guarantees that the path followed by $T$ has the same total length $\overset{\frown}{TA}=\overset{\frown}{TB}$. Therefore $T$ will land on $C$ in finite time.
As for the shadow $S$, $r>1/2$ implies that it always moves at speed $>1$, i.e. the (infinitesimal) arc length inequality $\delta_1<\delta_2$ always holds (see figure). This is a tedious but elementary trigonometric inequality, better left to the reader.
Before detailing how the thief's zigzags are decided, we need to notice that any policeman to the right of $A$, or to the left of $B$, by strictly more than $\overset{\frown}{SA}$, is inactive, in other words he can never catch $T$, even if $T$ runs towards him all the way to $C$.
Finally, enumerate all the policemen $P_1, P_2, P_3 \dots$
Take the first active policeman on the list, say $P_{i_1}$. If $P_{i_1}$ is to the left of $S$ (or on $S$) $T$ chooses to run right towards $A$ at speed $1$. (Similarly if $P$ were to the right, $T$ would choose to run left towards $B$.) Because $S$ moves at speed $>1$ at some point $P_{i_1}$ permanently falls behind $S$ by some amount $\epsilon_1$ (it doesn't matter how small). However $P_{i_1}$ may still be within the active range, so $T$ keeps running towards $A$ until $\overset{\frown}{SA}<\epsilon_1/3$. Since $\overset{\frown}{SB}=\overset{\frown}{SA}$, now $P_{i_1}$ is behind $B$ by strictly more than $\epsilon_1/3$. This renders $P_{i_1}$ permanently inactive!
Similarly, take the next active policeman $P_{i_2}$ on the list. Again $T$ runs in the direction away from him, until $P_{i_2}$ is inactive too. There at most countably many active policemen and one by one they all become inactive, guaranteeing that $T$ lands on a police-free point of $C$.$\quad \blacksquare$
This proof should easily extend to any curve $C$, since $T$ can first move close to a point of positive curvature, where locally the curve can be approximated well by a circle.

