3
$\begingroup$

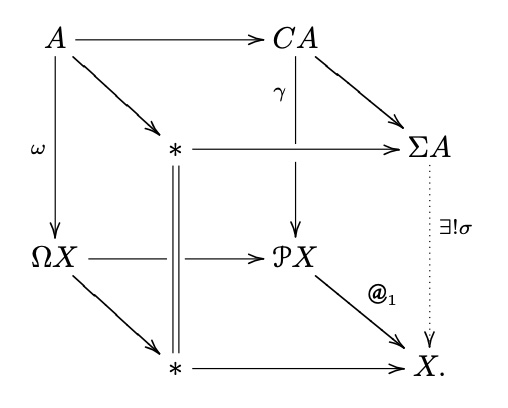
Given a map $\omega: A\to \Omega X$, one can set up the diagram enter image description here

and construct the map $\sigma : \Sigma A\to X$. It's pretty easy to check that the homotopy classes of $\omega$ and $\sigma$ correspond under the isomorphism $[A, \Omega X]\cong [\Sigma A, X]$ given by the exponential law. I'd like a good reference for this fact.

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ This is essentially a basic Peterson-Stein formula. See John Harper's Secondary Cohomology Operations chptr. 3.4 for a much more general statement (and a very careful treatement of signs!). $\endgroup$
    – Tyrone
    Commented Jun 18, 2021 at 11:53
  • $\begingroup$ Ok! I wouldn't have thought to check there, thanks! $\endgroup$
    – Jeff Strom
    Commented Jun 18, 2021 at 11:59

0

Reset to default

You must log in to answer this question.