The Newton Method over $\mathbb{R}$ has the property that the precision is doubled (under some continuous differentialbedifferentiable assumption) in each iteration. For the ring $\mathbb{Z}_p$ of $p$-adic integers, we also have the Hensel's lemma, but the precision is increaseincreased by 1 in each iteration. The proof about the double precision in the case over $\mathbb{R}$ uses the Taylor expansion and its remainder, see http://www.answers.com/topic/newton-s-method#Analysis here (Wayback Machine).
In a special case that is finding square root, one can use $x_{n+1} := \frac{1}{2} x_n - \frac{3}{2} x_n^3 $ ( by considering $x^{-2}-c^{-1}$ instead of $x^2-c$) and one can show that the precision is doubled in the case over $\mathbb{Z}_p$.
In general, is the precision is doubled in each iteration in the case over $\mathbb{Z}_p$, under some conditions?
=======================================================================================================
Sorry, I just fondfound that in fact the precision is doubled in $p$-adic case, without further assumptionassumptions. I mentioned the precision increaseincreased by 1, since this is written in "A first course in $p$-adic Analysis" by Alain M. Robert.
alt text http://i41.tinypic.com/f1d3qh.png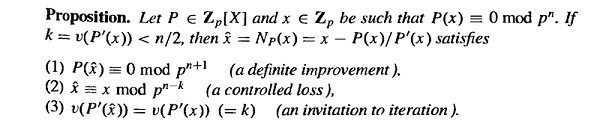
But if one examines the proof ,we we have $p(\hat{x}) \equiv 0 \ \mathrm{mod} \ p^{n-2k}$. And if $k = 0$, the precision is doubled.
