I don't think so. This boils down to finding a retraction from the group
$\langle a,b,c,d,q,r,t\rangle/\langle\langle tQabRcdT=QbaRdc\rangle\rangle$
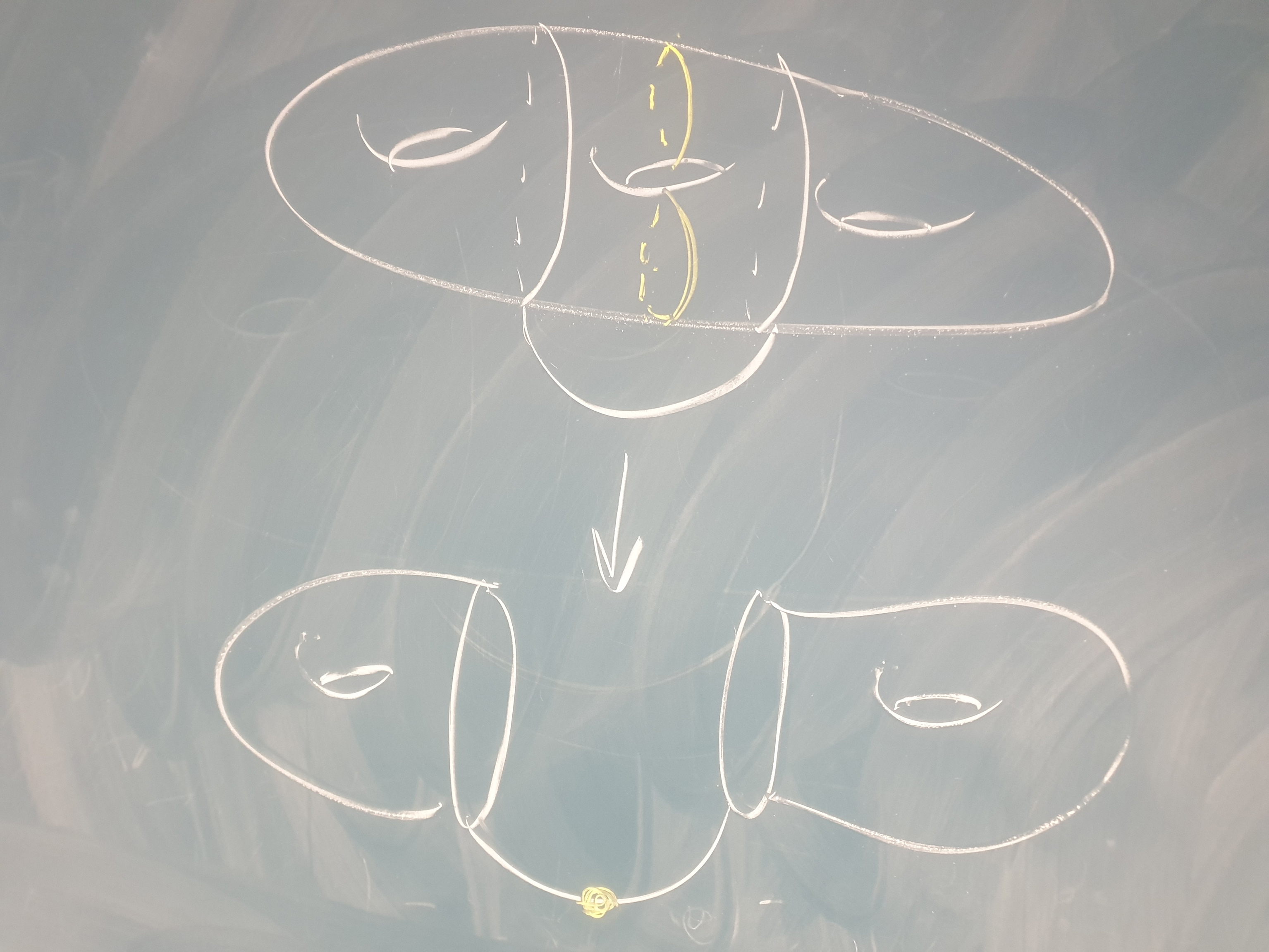
to the subgroup $\langle a,b,c,d\rangle$. The Whitehead graph of the word $tQabRcdTCDrABq$ has two connected components, both circles, and the presentation complex is a genus three surface with two points identified. It's not so easy to type into this comment box, but after a little fiddling around such a retraction would put you in the situation of the following picture, which is impossible:
We have blown up the bouquet of 7 circles corresponding to the generators $a,b,c,d,q,r,t$ by introducing a new edge, so that the blown up complex is genuinely a genus three surface with an edge attached. The $a,b$ and $c,d$ subgroups each create a handle, and the lower part of the picture represents this subgroup. The yellow point separates, and the preimage of this point under a (suitably chosen) retraction is a family of simple closed curves which separate the two handles. If this could happen then at least one of $[a,b]$ and $[c,d]$ would both have to die, but they don't, since it is a retraction.