Equation in the conjugacy class of a free group

Question

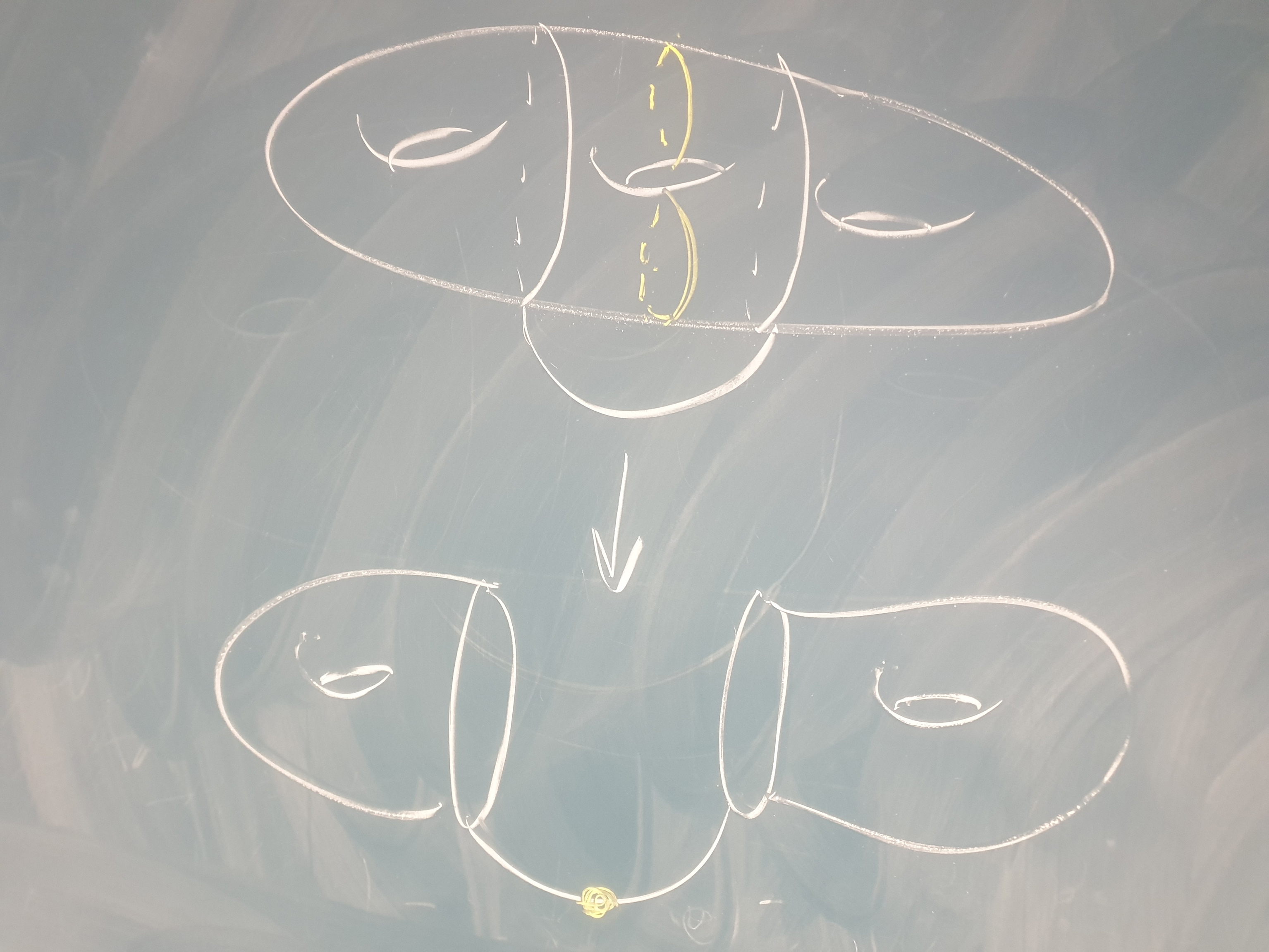
I will pose the question in the form in which it originally appeared to me:

Let $a,b,c,d$ be different letters in a finite alphabet $\mathcal{Z}$. Let $Q$ and $R$ be finite words with letters from $\mathcal{Z}$. Consider the words $QabRcd$ and $QbaRdc$ as being cyclic, e.g. by placing them on separate circles.

Do there exist different letters $a,b,c,d$ and words $Q,R$ such that the cyclic word $QabRcd$ can be obtained from $QbaRdc$ by rotation?

The appropriate context for this questions seems to be whether this equation has a solution in a conjugacy class of the free group generated by the letters of $\mathcal{Z}$, and hence the title.

Answer 1

I don't think so. This boils down to finding a retraction from the group

$\langle a,b,c,d,q,r,t\rangle/\langle\langle tQabRcdT=QbaRdc\rangle\rangle$

to the subgroup $\langle a,b,c,d\rangle$. The Whitehead graph of the word $tQabRcdTCDrABq$ has two connected components, both circles, and the presentation complex is a genus three surface with two points identified. It's not so easy to type into this comment box, but after a little fiddling around such a retraction would put you in the situation of the following picture, which is impossible:

We have blown up the bouquet of 7 circles corresponding to the generators $a,b,c,d,q,r,t$ by introducing a new edge, so that the blown up complex is genuinely a genus three surface with an edge attached. The $a,b$ and $c,d$ subgroups each create a handle, and the lower part of the picture represents this subgroup. The yellow point separates, and the preimage of this point under a (suitably chosen) retraction is a family of simple closed curves which separate the two handles. If this could happen then at least one of $[a,b]$ and $[c,d]$ would have to die, but they don't, since it is a retraction.