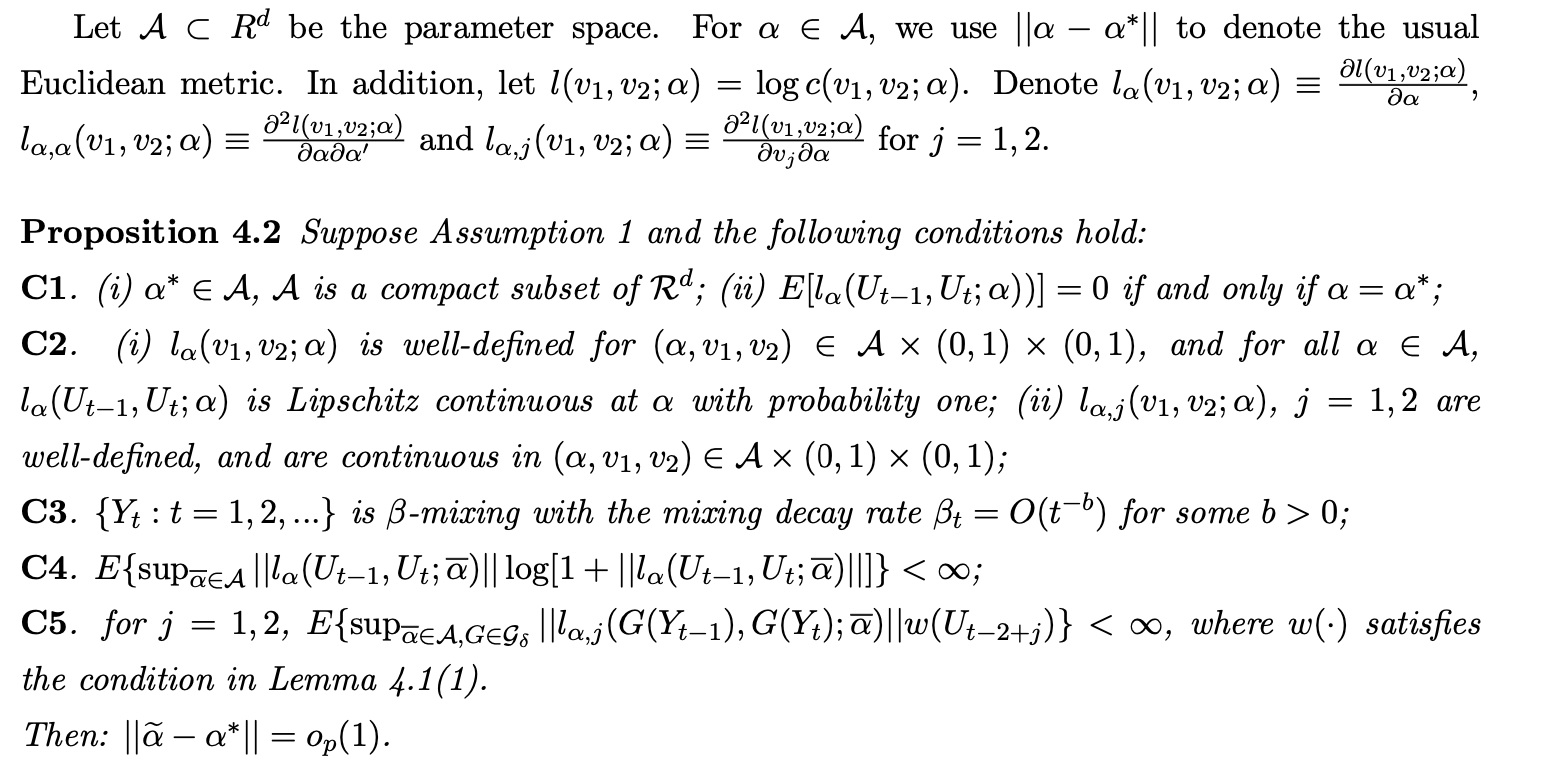
I am reading a well-known paper about copulas by Chen and Fan (2006). Specifically, Proposition 4.2 (see attached), in which all the arguments are uniform $U_t$$U_{t-1}, U_t$. However, when the copula is estimated the ECDF (in the empirical integral transform) should be used $\hat{G}_n(Y_t)$ as arguments, which is NOT uniformly distributed (only asymptotically).
I do not understand, why is it sufficient to prove Proposition 4.2 for uniform arguments, because from my point of view some sort of convergence should be proved:
$$\mathbb{E}l_\alpha \left(\hat{G}_n(Y_{t-1}), \hat{G}_n(Y_t) \right) \to \mathbb{E}l_\alpha(U_{t-1}, U_t;\alpha), \quad n \to \infty$$$$\mathbb{E}l_\alpha \left(\hat{G}_n(Y_{t-1}), \hat{G}_n(Y_t) ;\alpha \right) \to \mathbb{E}l_\alpha(U_{t-1}, U_t;\alpha), \quad n \to \infty$$
Which is completely not obvious for me.