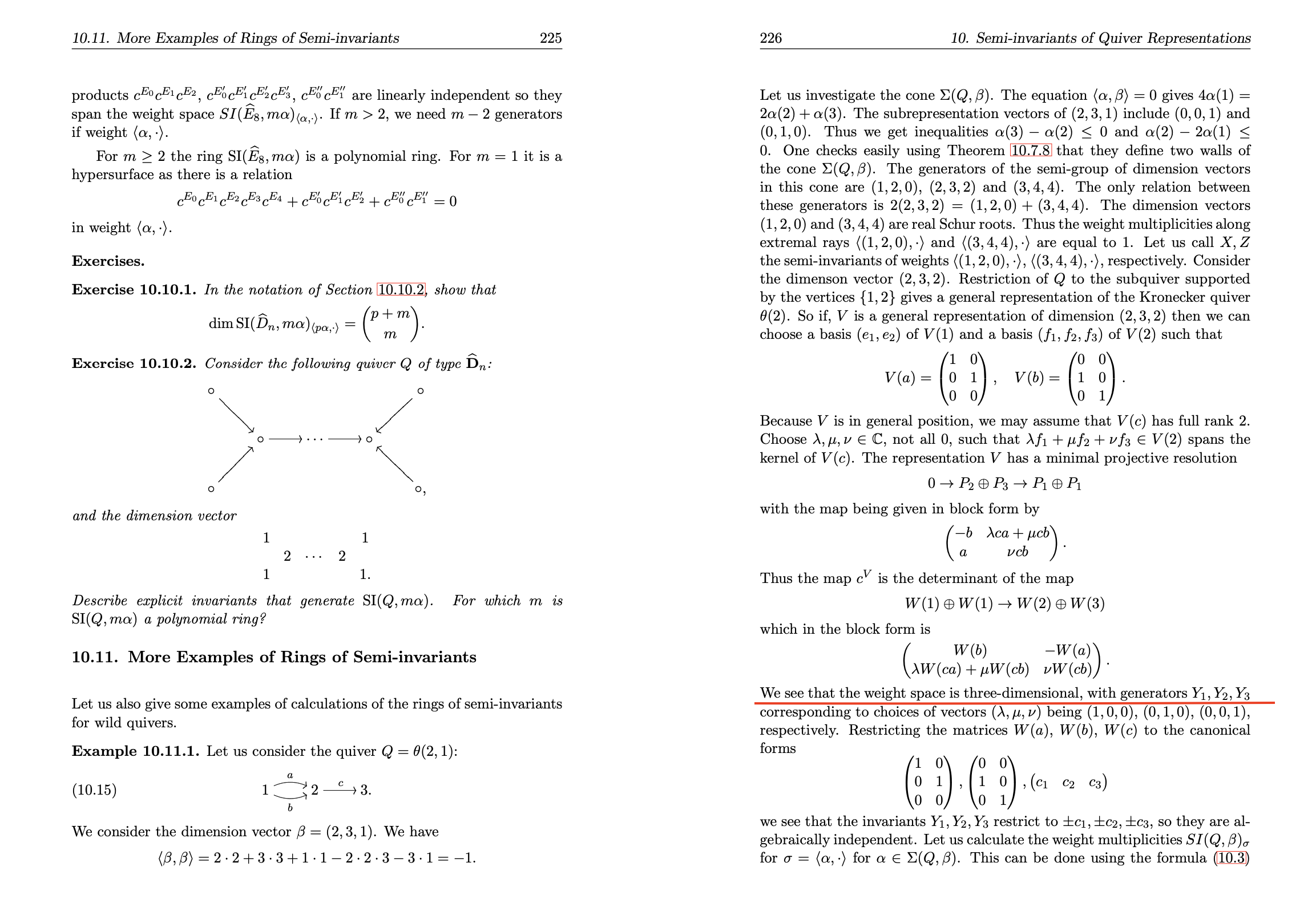
I'm trying to understand Example 10.11.1 on page 225 of the book "An introduction to quiver representations" by Harm Derksen and Jerzy Weyman (see the attached screenshot below)
I want to understand the line underlined in red.
How does the choice of vectors $(\lambda,\mu,\nu)$ being $(1,0,0),(0,1,0),(0,0,1)$ give us a basis for the weight space $\mathrm{SI}(Q,\beta)_{\langle(2,3,2),\cdot\rangle}$?
They are basically saying that the Schofield semi-invariants $c^{V}$ that you get by choosing these three vectors form a basis for the weight space $\mathrm{SI}(Q,\beta)_{\langle(2,3,2),\cdot\rangle}$ in the semi-invariant ring. This is the fact that I don't understand.