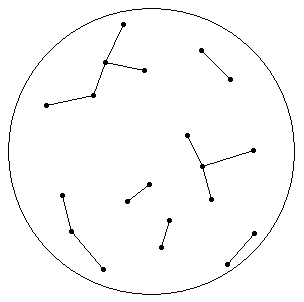
A disc contains $n$ independent uniformly randomdistributed points. Each point is connected by a line segment to its nearest neighbor, forming clusters of connected points.
For example, here are $20$ random points and $7$ clusters, with an average cluster size of $\frac{20}{7}$.
What is the expectation of the average cluster size, as $n\to\infty$ ?
I made a random point generator that generates $20$ random points. The expectation of the average cluster size seems to be approximately $3$.
This question was posted on Math SE. This answer provides useful context (but does not answer the question).
This question was inspired by the Math SE question Stars in the universe - probability of mutual nearest neighbors.