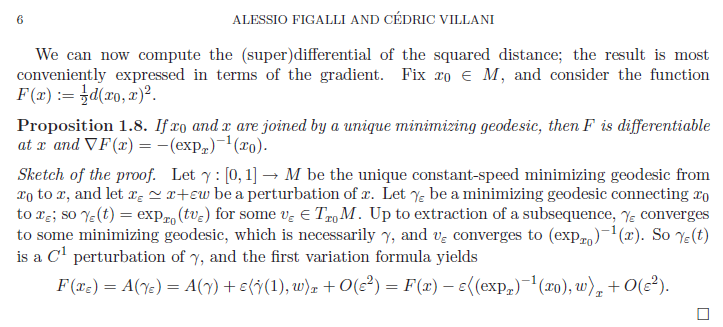
Motivation for the question: This is a question that I encountered when reading Proposition 1.8 of the paper "Optimal transport and curvature" by Alessio Figalli and Cedric Villani, whose proof is only outlined and involves construction of a $C^1$ variation of the unique, minimal geodesic connecting 2 distinct points. This proposition asserts that the squared Riemannian distance function from a fixed point to another is differentiable at the other point if there is a unique minimizing geodesic connection these two points. However, my question is a specific scenario of the original setting of this proportion, and it is also motivated by my study on first conjugate points with unique minimal geodesics (in a related post here: Differentiability of distance function at first conjugate point) and on the structure of cut loci in Riemannian manifolds with non-negative sectional curvature.
Description of question: Let $M$ be a complete Riemannian manifold with distance function $d$. Fix two distinct points $p, q \in M$, and consider the case where there is a unique, minimizing geodesic $\gamma$ connection $p$ and $q$, such that $q$ is the first conjugate to $p$ along $\gamma$. Here "minimizing" means "length or distance minimizing", i.e., $d(\gamma(0),\gamma(t))=t$ for $t \in [0,d(p,q)]$. Such a setting exits on, e.g., a paraboloid; see, e.g., Figure 2 of the paper "Spectral Flow, Maslov Index And Bifurcation Of Semi-Riemannian Geodesics" by Paolo Piccione, Alessandro Portaluri, And Daniel V. Tausk.
How to construct a $C^1$ variation $c(s,t)$ of $\gamma$ for $(s,t) \in (-a,a) \times [0,d(p,q)]$ for some $a>0$, such that the following hold:
- $c(0,t)=\gamma(t)$ for all $t \in [0,d(p,q)]$, and $c(s,0)=p$ for all $s \in (-a,a)$, and
- for each fixed $s \in (-a,a)$, $c(s,t)$ is a minimizing geodesic connecting $p=c(s,0)$ and $c(s,d(p,q))$, and
- for each fixed $t \in [0,d(p,q)]$, $c(s,t)$ is continuously differentiable in $s$ for $s \in (-a,a)$?
An answer to this question basically provides the needed details in the proof of Proposition 1.8 mentioned above.
Background research: There is a post here under the title "https://math.stackexchange.com/questions/3726838/constructing-a-proper-variation-through-geodesics-at-cut-locus". But the discussion for it was diverted and the question in title was never discussed or answered.
Also, I have looked through 3 textbooks (John M. Lee's "Introduction to Riemannian Manifolds", Takashi Sakai's "Riemannian Geometry", and Peter Petersen's "Riemannian Geometry") for a hint on how to construct such a variation but found none. All these textbooks provide variations of the form $c(s,t)= \exp_{\lambda(s)}(t \mathbf{w}_s)$, which do not satisfy item 2 given above.
The key difficulty I see to constructing a $C^1$ variation of $\gamma$ when $q$ is in the cut locus of $p$ is the non-uniqueness of geodesics connection $p$ and a point in any neighborhood of $q$ (except possibly $q$ itself), since Theorem 2.1.12 on page 133 of W.A. Klingenberg's book "Riemannian Geometry" states that, within any neighborhood of the first conjugate point $q$, there exists a point $z$ such that there are at least two distinct geodesics that connects $p$ and $z$.
For $c(s,t)$ to be a $C^1$ variation of $\gamma$, its corresponding initial speed vectors have to be continuous in $s$ for $s \in (-a,a)$. This continuity is a sufficient condition to pass differentiation (with respect to $s$) under the integral sign (with respect to $t$) when proving the first variation formula, and of course, a weaker sufficient condition is to assume that the supremum norm of the partial derivative (here as covariant derivative along $\gamma$) in $s$ of $c(s,t)$ is upper bounded by a Lebesgue integrable function $Q(t)$ and then apply the dominate convergence theorem to pass differentiation under the integral sign in order to prove the first variation formula.
Here is what I tried:
- Take a geodesic $h(s)$ such that $h(0)=q$ and $h'(0)=\mathbf{w}$ for $s \in (-a,a)$ for some $a>0$;
- For each $s$, let $K_s$ be the set of initial velocity vectors (in $T_p M$) of all the minimizing geodesics that connect $p$ and $h(s)$. Note that $K_s$ is non-empty by Hopf-Rinow theorem, and that $K_s$ is a compact subset of $T_p M$ for each $s \in (-a,a)$;
- Now the key issue of picking initial velocity vectors. Fix an $s$, let $\mathbf{v}_s$ be an element of $K_s$ that has the smallest angle with $\gamma'(0)=\mathbf{v}$. Set $\sigma_s(t)$ to be the minimizing geodesic connecting $p$ and $h(s)$ such that $\sigma'_s(0)=\mathbf{v}_s$.
Missing part in my trial: The above construction only asserts that $\mathbf{v}_{s_{j}} \to \mathbf{v}$ along each sequence $s_j \to 0$ (because $\gamma$ is the unique, minimizing geodesic connecting $p$ and $q$), and it does not imply that $\mathbf{v}_s$ is continuous in $s$ for $s \in (-a,a)$. Here continuity is defined with respect to the Riemannian metric on $T_p M$ and the canonical norm (i.e., absolute value of difference) on $(-a,a)$. Note that in the proof sketch of Proposition 1.8 of the paper "Optimal transport and curvature" by Alessio Figalli and Cedric Villani, the authors mentioned "Up to extraction of a subsequence".
Any suggestions or points would be appreciated. Thank you.