This is a paper written by Series "THE MODULAR SURFACE AND CONTINUED FRACTIONS".
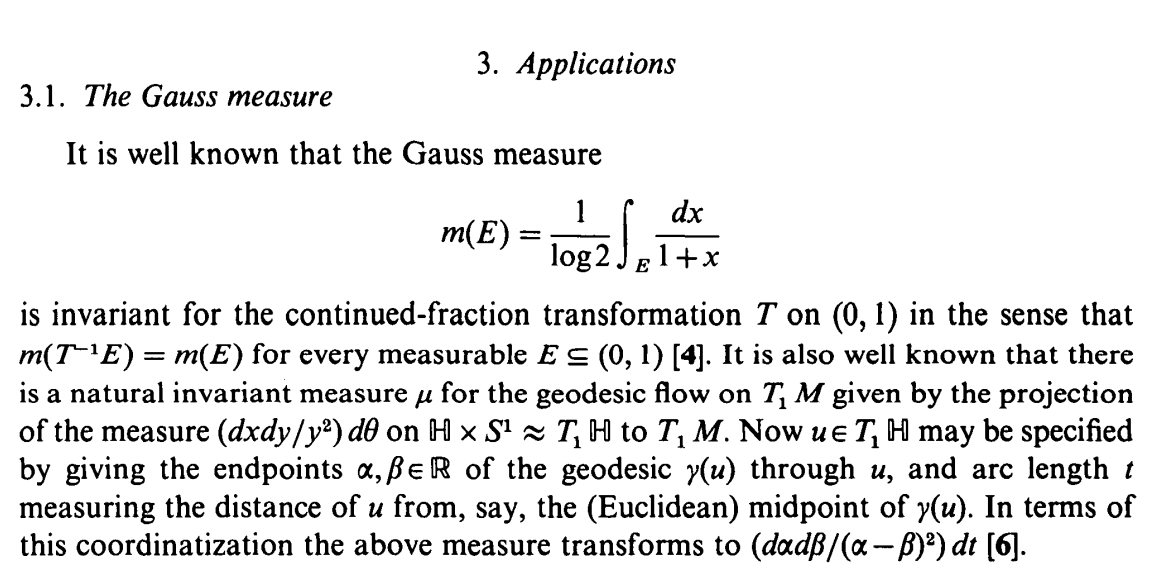
I want to know about above construction natural invariant measure $\mu$ for the geodesic flow on $T_{1}M$ where M is the modular surface. Moreover, I want to know this coordinatization following measure. [6] is an article by E. Hopf, 'Ergodentheorie', Abh. Sächs. Akad. Wiss. Leipzig, 91 (1939), but the construction of this measure does not seem to go into much detail. If anyone knows more about it, I'd like to know how to construct it and whether the product measure is an invariant measure. Is this method of construction well known?