Consider the algebraic group $G:=\operatorname{SL}_{2}\times\operatorname{SL}_{2}$ acting on $V:=\operatorname{Mat}_{2\times 2}\oplus\operatorname{Mat}_{2\times 2}$ via the action $(A,B)\,\cdot\,(X,Y)=(BXA^{-1},BYA^{-1})$. I'm interested in computing the invariant ring $\mathbb{K}[V]^{G}$. For this purpose I'm using the "InvariantRing" package in Macaulay2 (see here)and I did the following 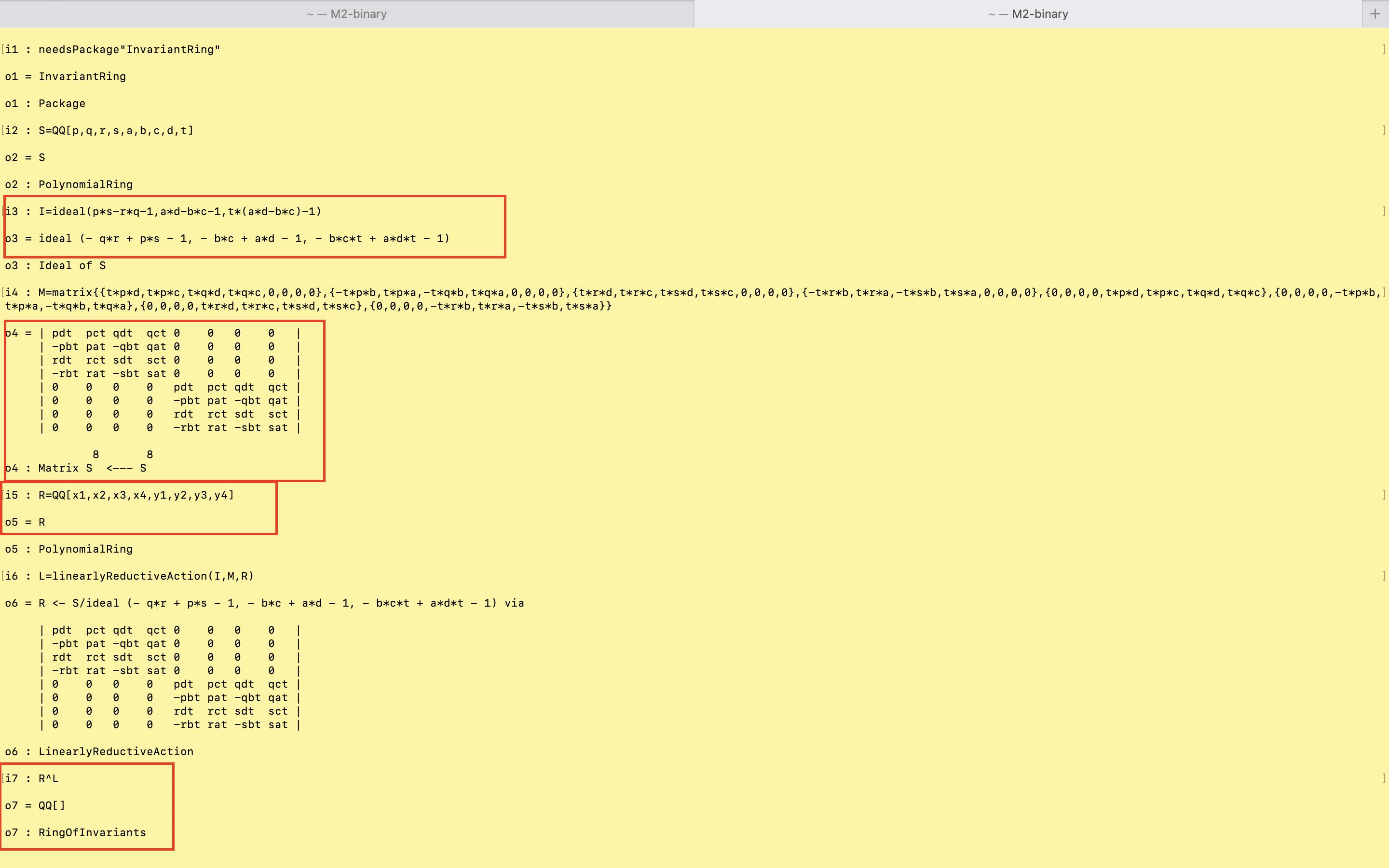 But for some reason it is giving the wrong output. It returns the invariant ring to be trivial, which I know for sure is not the case. I repeated all of these over $\mathbb{C}$ and still got the same output.
But for some reason it is giving the wrong output. It returns the invariant ring to be trivial, which I know for sure is not the case. I repeated all of these over $\mathbb{C}$ and still got the same output.
I'm confused where is the mistake. I would highly appreciate your time and effort!!
For people who are familiar with quiver representations, I'm looking at Kronecker quiver with dimension vector (2,2).
Edit: I have done the above computation without taking $t$, i.e., by taking the ideal to be $(ps-rq-1, ad-bc-1)$ and without $t$ in the matrix, I still got the same output.
