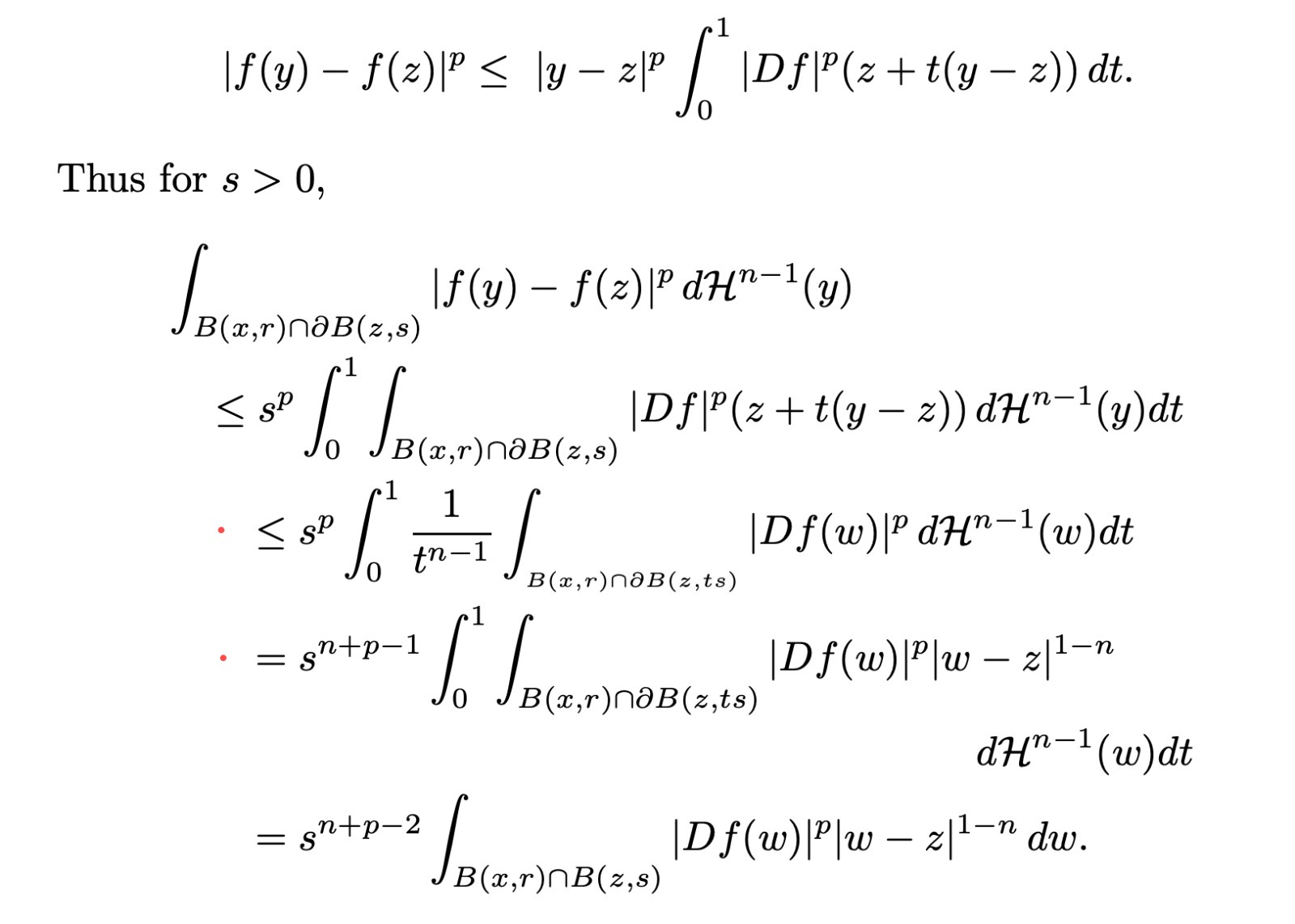I am reading at Evans' book Measure Theory and Fine Properties of Functions, Revised Edition, p. 165 and I can't see how one gets the transition from the first dotted (🔴) integral to the second one --
-- here $Df$ is just the gradient of $f$ and $d H^{n - 1}$ is the Hausdorff surface measure.
Any help would be much appreciated!