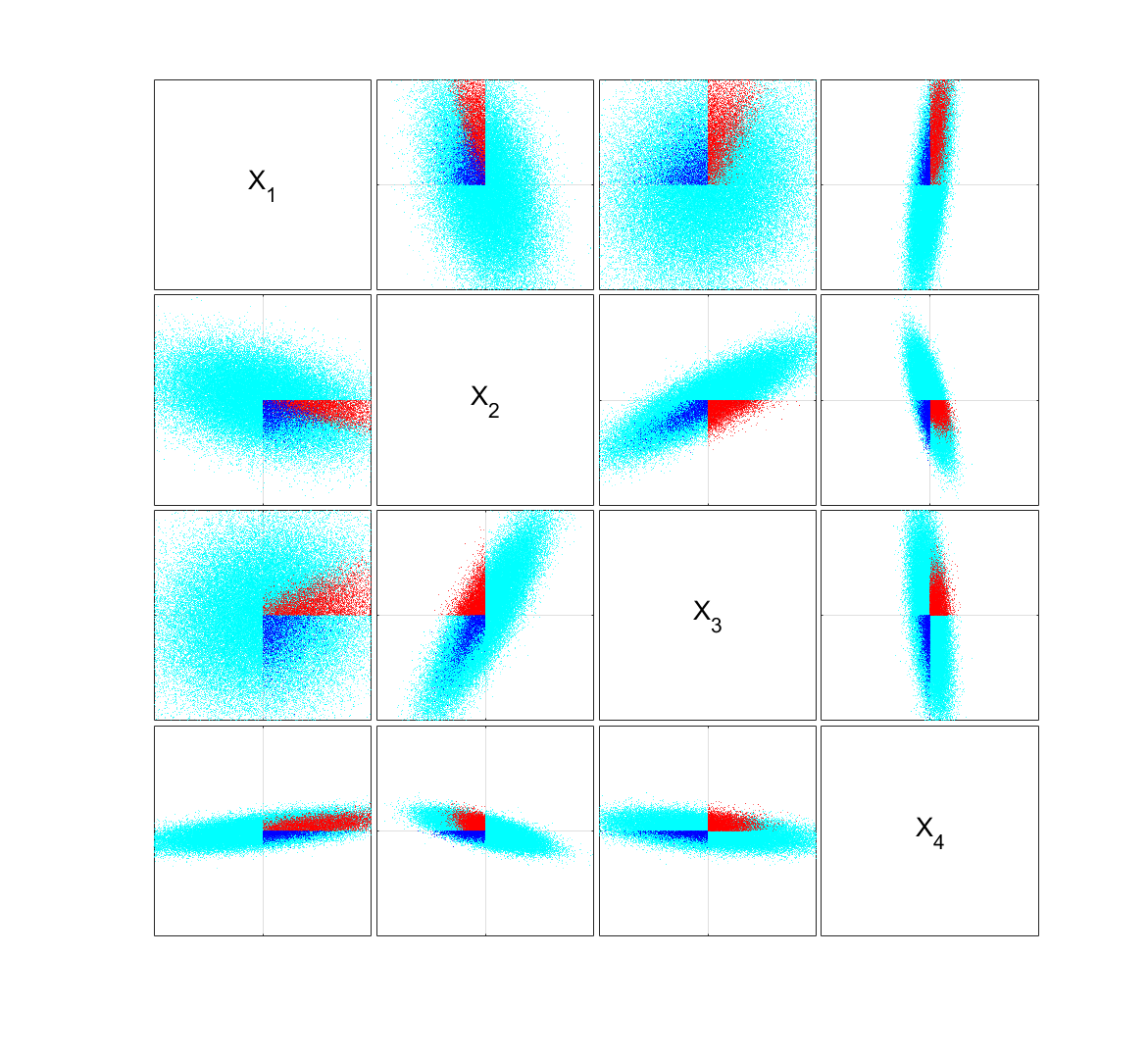
Comparing orthants 4 and 7, we observe the latter has greater $\lVert v_1 \rVert$, but clearly smaller probability. I calculated the probabilities with two methods: p is from MATLAB mvncdf which claims absolute error tolerance $10^{-4}$, and p_MC is from Monte-Carlo integration with $10^7$ points. The probabilities differ already in the second decimal, so I'm pretty confident that it is not just a numerical roundoffan artefact of numerical calculation.
More counterexamples can be generated by making random instances and checking the orthant probabilities: the conjecture fails every now and then, although it holds in most of the cases. Interestingly, in 3 dimensions I have not found any counterexample in thousands of random instances (either in the prolate case = large eigenvalue is single and small eigenvalue is double, or in the oblate case = large is double and small is single).
Each subplot shows a 2D projection to two coordinate axes, in the same scale (each: each box ranges from $-20$ to $+20$ in both directions). Note that the two contending orthants are in the same quadrant of $X_1,X_2$, and in opposite quadrants of $X_3,X_4$.