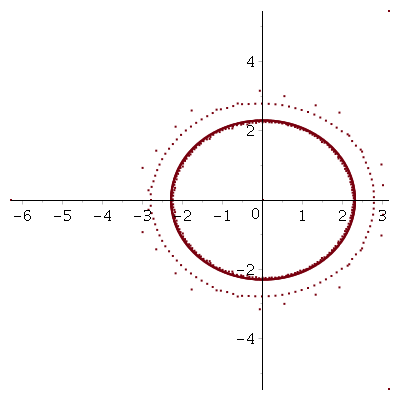The series $$\sum_{n=1}^\infty\frac{z^{p_n-n}}{n!}$$ associated to the
sequence $p_1=2,p_2=3,p_3=5,p_4=7,p_5=11,\ldots$ of prime numbers
defines a holomorphic function in the open disc of radius $e$.
Its roots have a curious onion-like structure. Cutting at $n=100$
(corresponding to $p_{100}=541$) yields a polynomial of degree $541-100=441$
with complex roots forming the picture
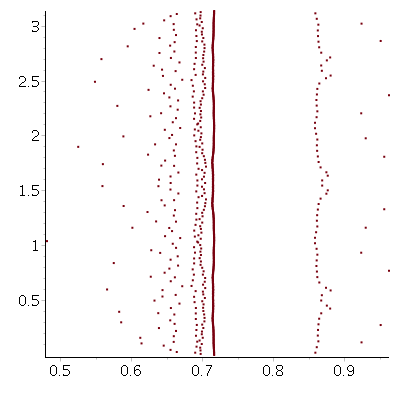
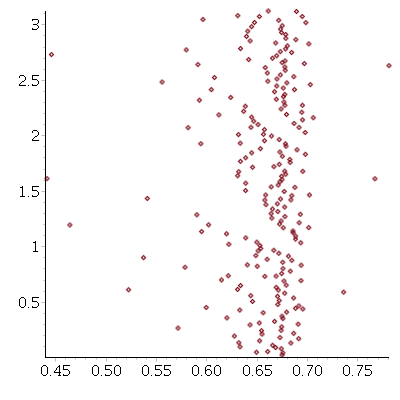
The onion-like structure of these roots is even more apparent
when taking logarithms of all non-zero roots. Logarithms of roots
in the open upper half-plane form the picture

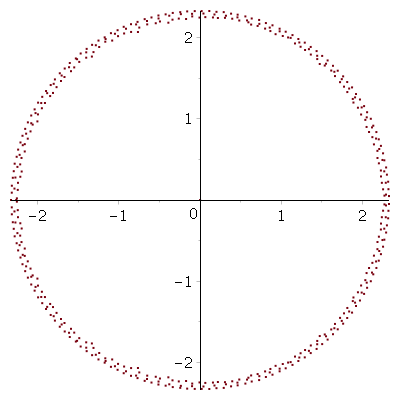
In order to check that primes lead to especially nice roots, one can also
consider the series
$$\sum_{n=3}^\infty \left(\lfloor n/\log n)\rfloor-\lfloor (n-1)/\log n-1\rfloor\right)\frac{z^{n-\lfloor n/\log n\rfloor}}{n!}$$
with roots for the polynomial of degree roughly 440 forming the picture
which lacks the onion-structure.
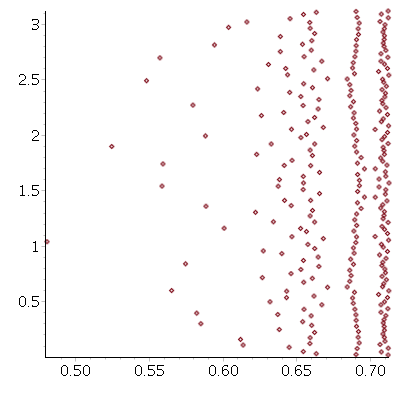
Logarithms of roots in the upper half-plane give rise to
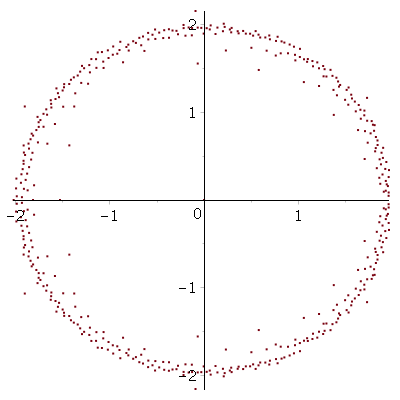
The series $\sum_{n=1}^\infty \frac{z^{p_n}}{n!}$ seems (perhaps) also a bit
less interesting. Cutting at $n=100$, we get the roots
with logarithms of roots in the upper half-plane given by
The onion-structure of the initial series
could of course be an artefact due to rounding errors or
due to truncation of the series at $n=100$.
I can exclude rounding errors with reasonable confidence
since I did the computations with a precision of 1500 digits.
The onion-structure of root-sets seems to persist by
setting the cutoff $n$ to higher values.
A somewhat arbitrary convention is the indexing of primes.
Series of the form $\sum_{n=1}^\infty\frac{z^{p_n-(n+\tau)}}{(n+\tau)!}$
for small $\tau=1$ or $\tau=-1$ behave however similarly. (The onion-structure
is slightly messier for $\tau=-1$ and roughly equivalent for $\tau=1$.)
Is there an explanation for this phenomenon?
Obvious remark: Shells of the limit onion-structure (in case of existence)
are finite (since roots of holomorphic
functions are discrete in their domain of holomorphicity).
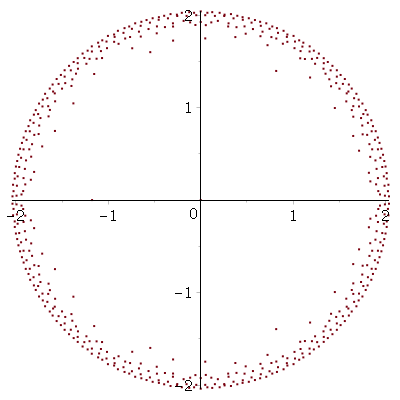
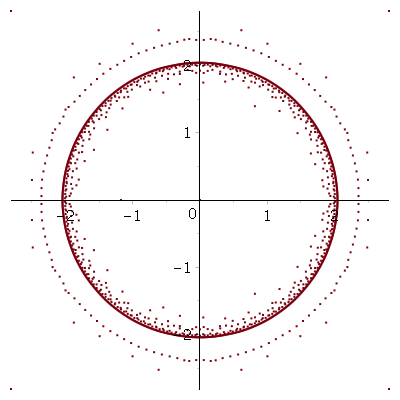
Added: Computations using 800 digits and ending with $p_{250}=1883$$p_{250}=1583$ yield pictures
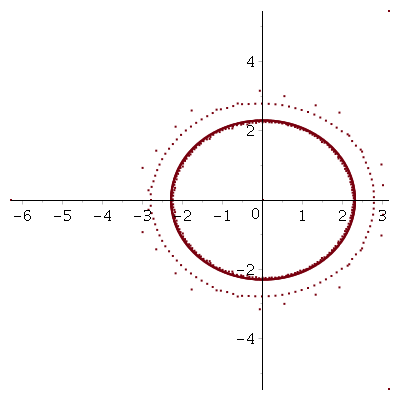
for roots of $\sum_{n=1}^{250} z^{p_n-n}/n!$.
There is a shell of roots and a few 'isolated' roots outside the domain of convergency.
Logarithms of roots in the upper open halfplane and in the convergency domain are given by
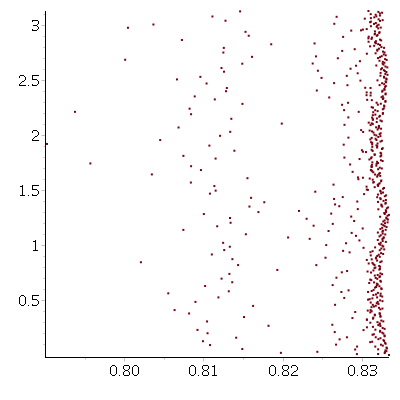
For the polynomial $\sum_{n=1}^{250}z^{p_n}/n!$
roots (computed again with Digits set to 800 in Maple) are
There are again a few roots outside of the convergency domain but there seems to be
an additional shell of roots (artefact due to insufficient precision or real roots?).
Logarithms of roots in the convergency domain and in the upper open halfplane are given by