In the excellent "A Handbook of Model Categories" (2021), cofibrant and fibrant homotopies are defined exactly as it seemed natural to me: immediately through functorial cylindrical objects / path space objects (hereinafter I will only talk about cylindrical objects), and the concept of non-functorial cylindrical objects in general not mentioned. But for proofs of elementary statements such as "cofibrate homotopy is an equivalence relation if dom is a cofibrant" Scott Balchin refers to Hovey, who proves symmetry and transitivity by constructing new cylindrical objects.
I would like to dispense with the notion of a non-functorial cylindrical object altogether. Instead, I am attracted by the search for natural operations on functorial cylindrical objects. For example, applying the cofibrate functorial factorization to the automorphism $\rm{swap}$ (of the codiagonal morphism) defines a natural automorphism of a cylinder, which gives a natural proof of the symmetry of cofibrate homotopy.
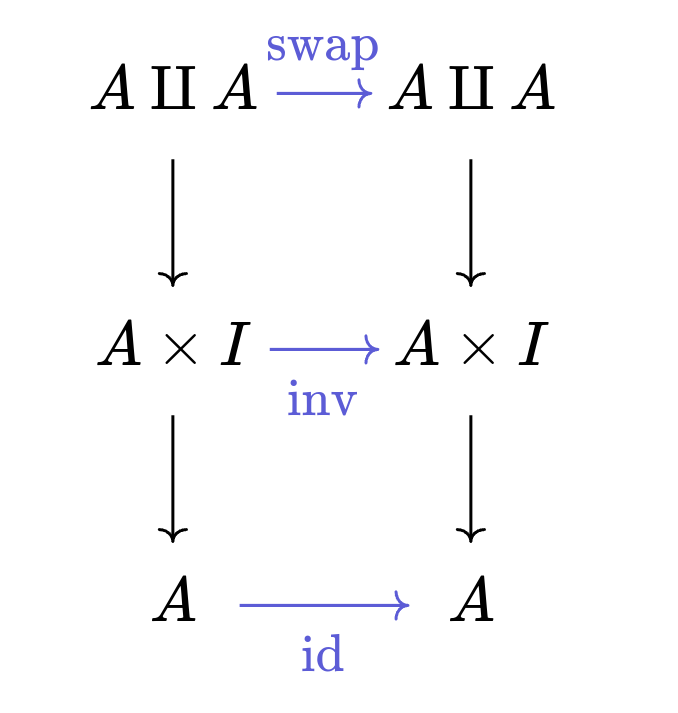
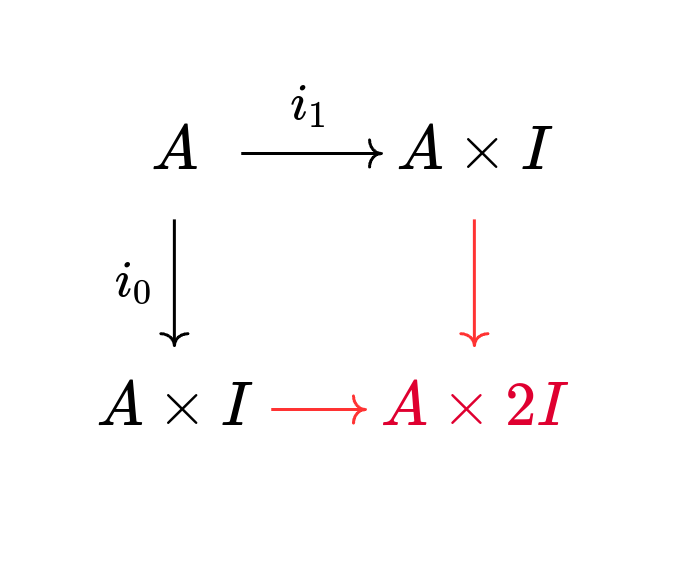
To prove transitivity, it is natural to glue two cylinders along the upper and lower bases and construct a natural isomorphismmorphism $\alpha \colon A \times I \to A \times 2I$ such that $i_0 \circ \alpha = i_0 \circ s_0$ and $i_1 \circ \alpha = i_1 \circ s_1$. Here $i_0, i_1 \colon A \to A \times I$ are compositions of the resulting 'long cylinder'inclusions $A \times 2I$$A \to A \amalg A$ with the standard cylindercanonical embedding $A \times I$. It is easy to see that this is sufficient for gluing homotopies$A \amalg A \to A \times I$, i.e. transitivityand $s_0, s_1$ are push-out arrows.
In an arbitrary model category, such an isomorphism definitely cannot exist: say, in a trivial model structure $\rm{Cofib} = \cal{C}, \rm{Fib} = \rm{Iso}~\cal{C}, \rm{WeakEq} = \cal{C}$, it is easy to see that that $A \times I = A \amalg A, \; A \times 2I = A \amalg A \amalg A$ (although all cylindrical objects in any model category are weakly equivalent). Is there a natural wide class of model categories (possibly with an additional structure) in which it is possible to construct a natural isomorphism ofsuch morphism $A \times I \to A \times 2I$ (at least for cofibrates $A$)? Or does the desire to limit ourselves to such categories select convenient model categories for some interesting homotopy categories? (I'm just starting to learn model categories)
P.S. Of course, I write function composition in direct order (less common).
UPD. The question has been slightly edited, see version history for the context of Mike Shulman's answer.
