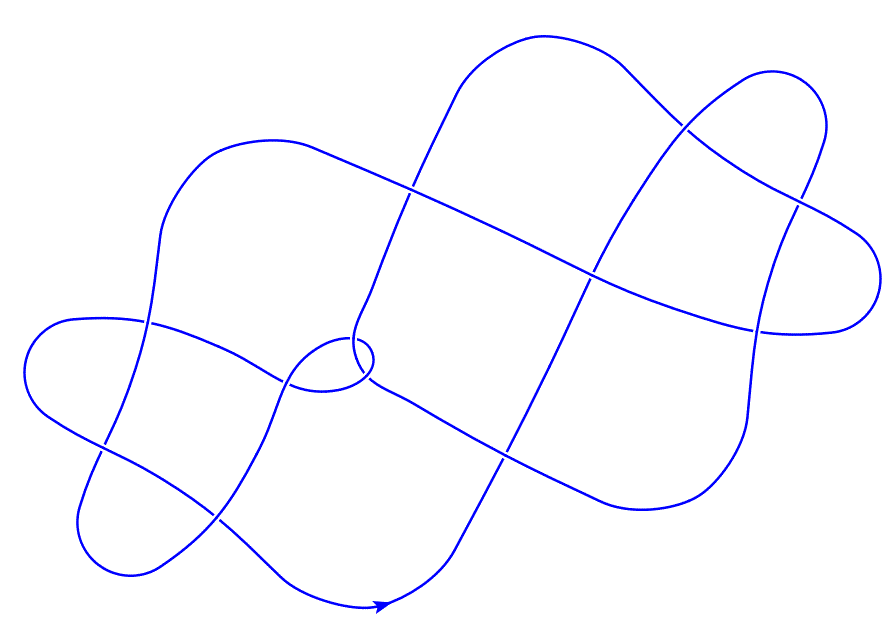
Here is a strongly invertible diagram of 10_49. The symmetry axis is almost horizontal.
This was created by loading the 'standard' diagram of 10_49 to KLO and then using KLO's simplifying method together with some work by hand. (KLO's simplifying method seems to prefer symmetric diagrams this is why I would expect the method to work in most cases.)
We have used similar methods for example here to create strongly invertible surgery diagrams. I would expect that the same works for the other 7 knots from your list. If you need help with that let me know.
EDIT: For 10_62 and 10_65the other knots it works similar. Here are diagrams of them:.
10_62
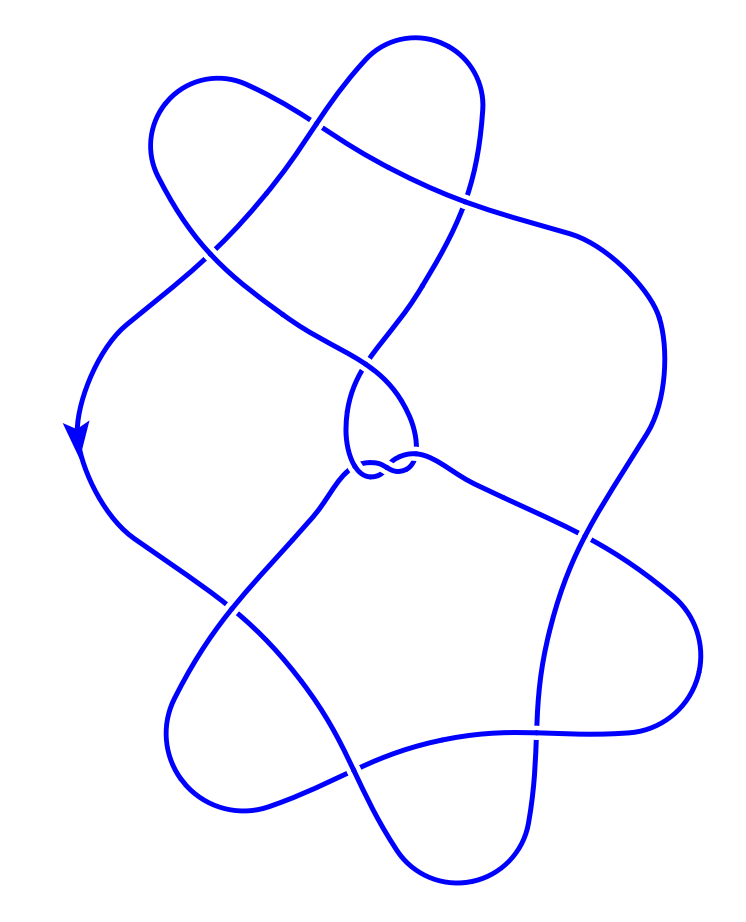 10_65
10_65
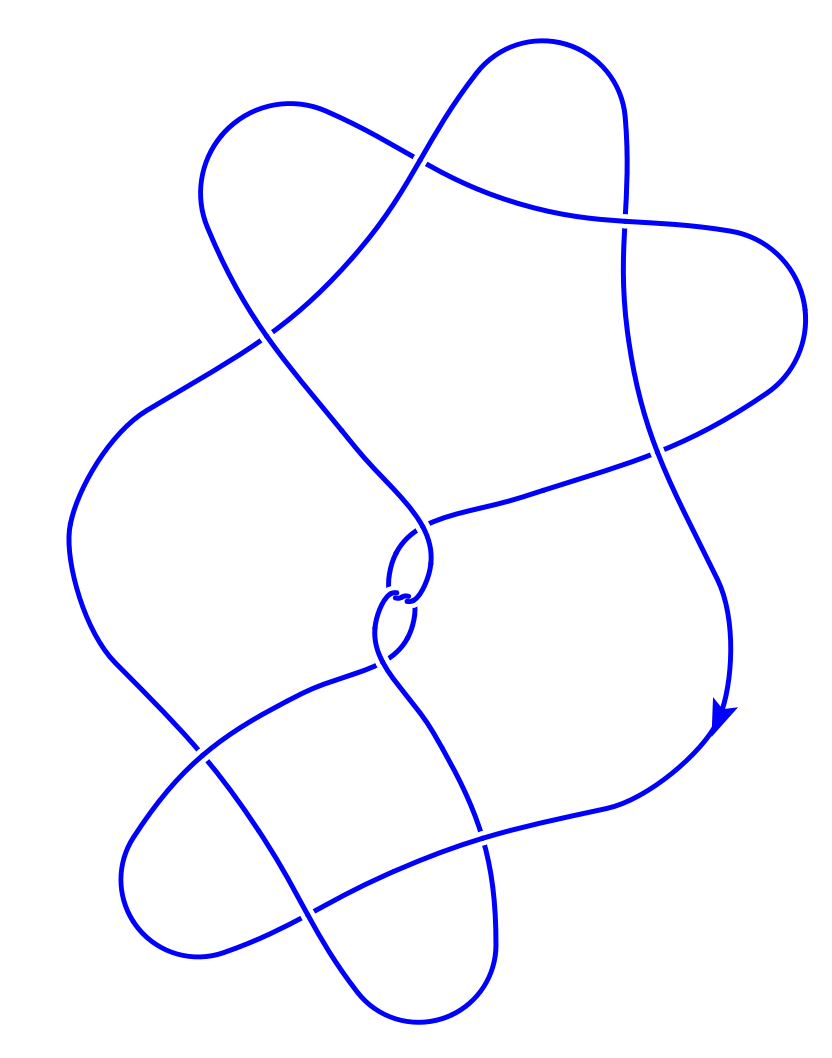 10_113
10_113
 10_143
10_143
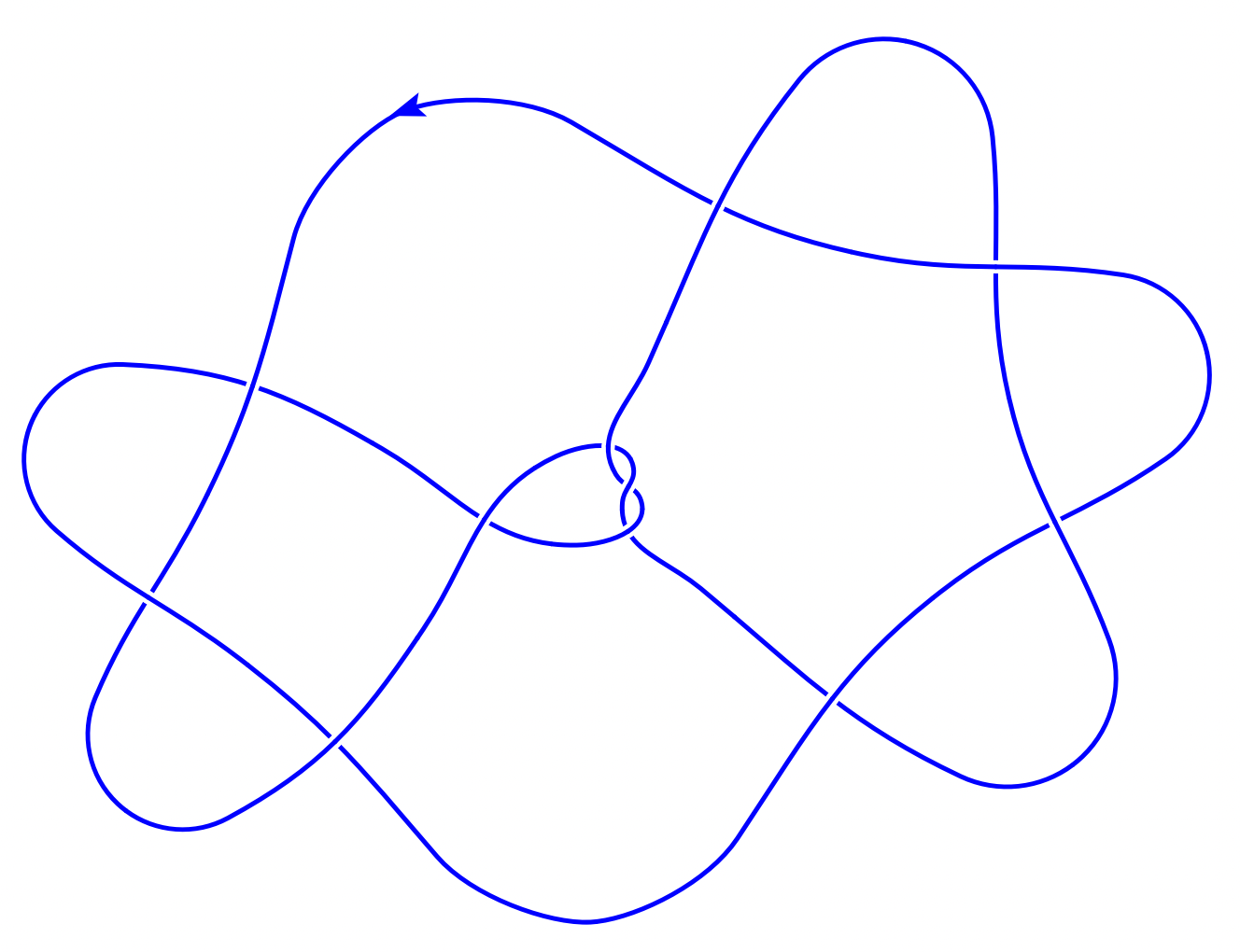 10_152
10_152
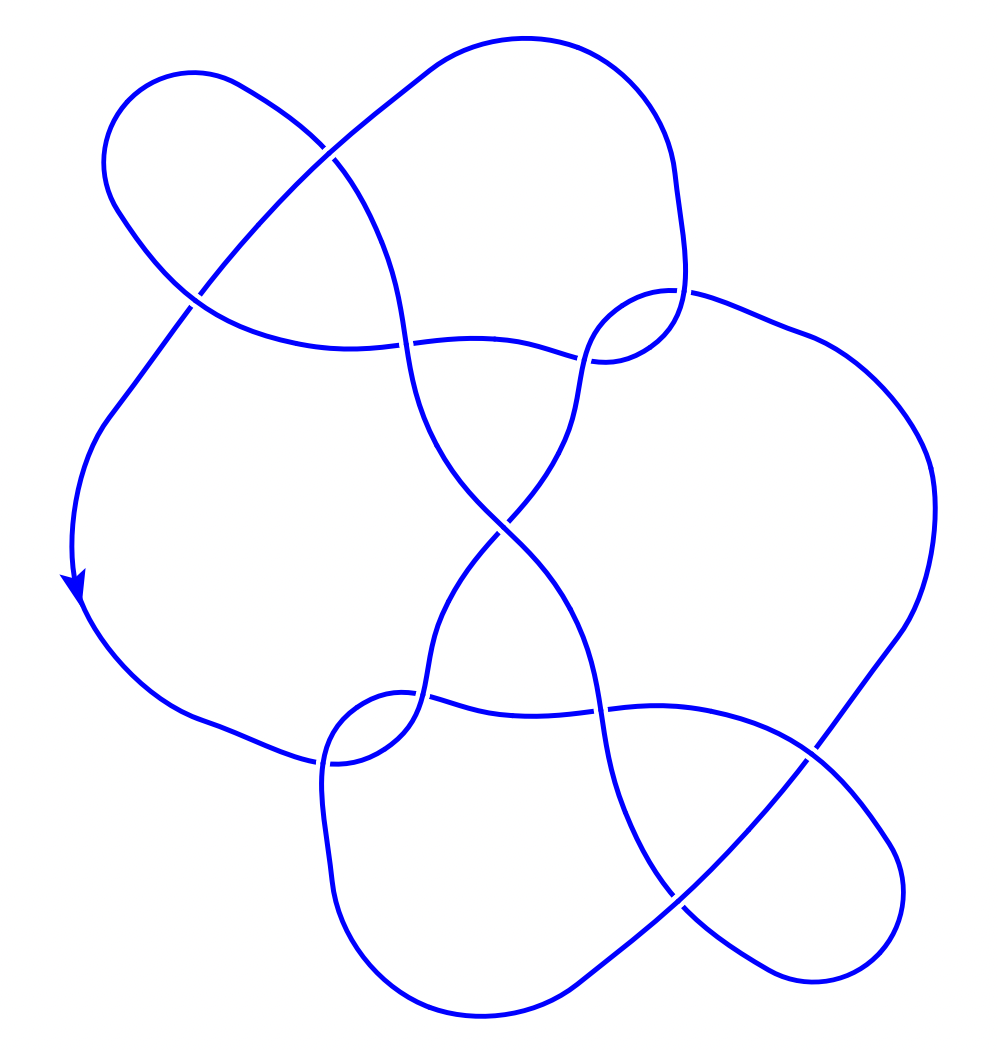 10_154
10_154
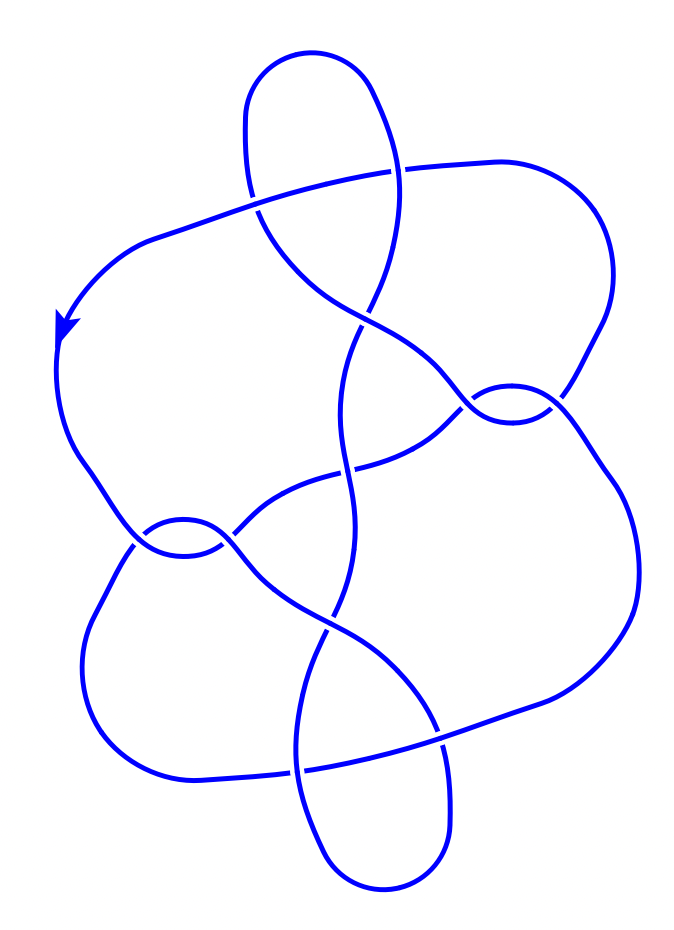
(For the last two the symmetry axis is orthogonal to the projection plane.)