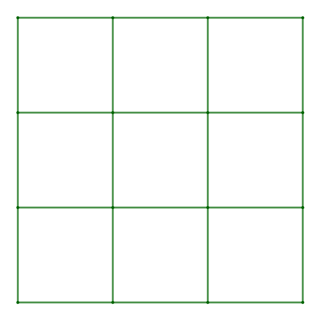
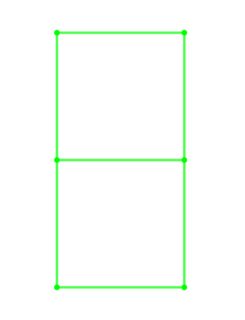
Weeds have taken over the paths. If mowed, they don't grow back, but unmowed weeds spread at speed $1$ along the road. What's the minimum speed of the mower to get rid of all the weeds? Roads are connected at each intersection and the mower must move on roads.

Source: Here's a more cinematic, but mathematically equivalent formulation of the same puzzle.

Weeds have taken over the paths (two squares). If mowed, they don't grow back, but unmowed weeds spread at speed $1$ along the road. What's the minimum speed of the mower to get rid of all the weeds? Roads are connected at each intersection and the mower must move on roads.

I've edited the problem to reduce the number of squares from 9 to 2. But the dynamics of the optimal solution still seems eluding. The puzzle was initially asked here.