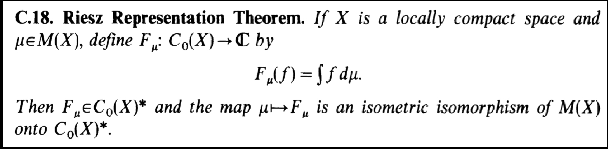I need to use the version of this theormtheorem about the dual space of $C_0(X)$, which is the set of continuous functions on X which vanish at infinity.
I found in wikiWikipedia that:
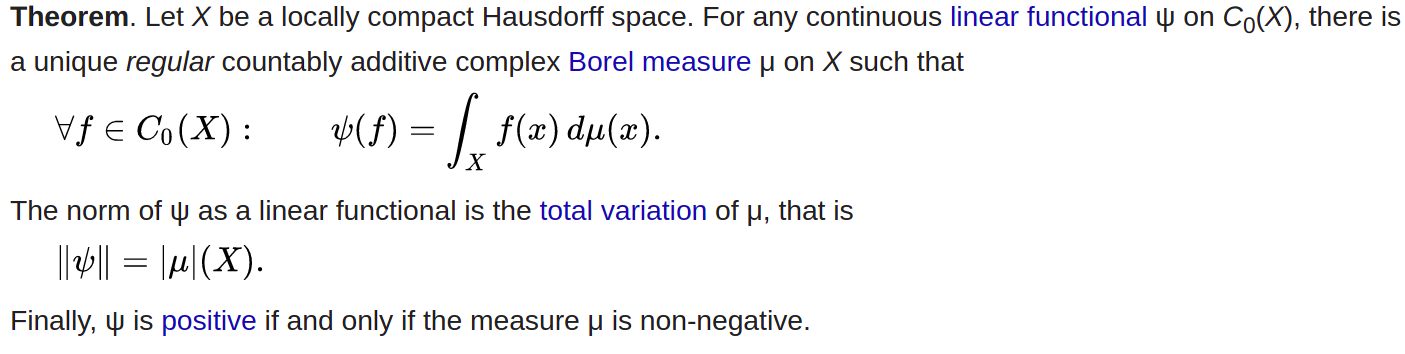
Here the space $X$ is needed to be LCH space. But I also found in Page 383 of Conway's book A course in functional analysis 2nd that:
Here $M(X)$ denotes the space of regular Borel measures and the space $X$ is not need to be Hausdorff. Now my puzzles are:
1)Does the Thm appliestheorem apply for a general locally compact space which is not necessarily Hausdorff?
2)Conway said "$M(X)$ is a normed space, the norm $\left\|\mu\right\|=\left|\mu\right|(X)$". But I think not every $\mu$ in $M(X)$ is finite, such as the Lebesgue measure of the real line. So should the denotiondefinition $M(X)$ be modified to "the space of finite regular Borel measures"? In this sencesens, $M(X)$ is a well-defined normed space and the isometrically isomorphimisomorphism gets its meaning.
Is there somebody who havehas read Conway's Book and Couldcould you help me? Thanks very much!