I was looking for ways to continue gap series, and it seemed to be that they could be continued outside of the boundary by simply turning $$f(x)= \sum_{n=0}^\infty x^{n^k}$$ into $$g(x) =- \sum_{n=1}^\infty x^{-n^k}$$ for odd values of k.
These two functions seem to agree at any angle that is a rational multiple of $\pi$. Plugging in $x = \cos\left(\frac{p}{q} \pi\right) + i\sin\left(\frac{p}{q} \pi\right)$ gives:
$$f(x) =\sum_{n=0}^\infty x^{n^k} = \sum_{n=0}^\infty \cos\left(\frac{p}{q} \pi n^k\right) + i\sin\left(\frac{p}{q} \pi n^k\right)$$
$$g(x) =-\sum_{n=1}^\infty x^{-n^k} = \sum_{n=1}^\infty -\cos\left(\frac{p}{q} \pi n^k\right) + i\sin\left(\frac{p}{q} \pi n^k\right)$$
The imaginary parts are equal since $\sin(0)=0$, so both series are exactly the same. The imaginary part also appears to converges when we are at a rational multiple of $\pi$, and seems to agree with the the method of using Ceasero summation. For instance, at the angle $\frac{2}{7}\pi$, the function and its continuation looks like this:
 Zooming in on the point $x = 1$:
Zooming in on the point $x = 1$: 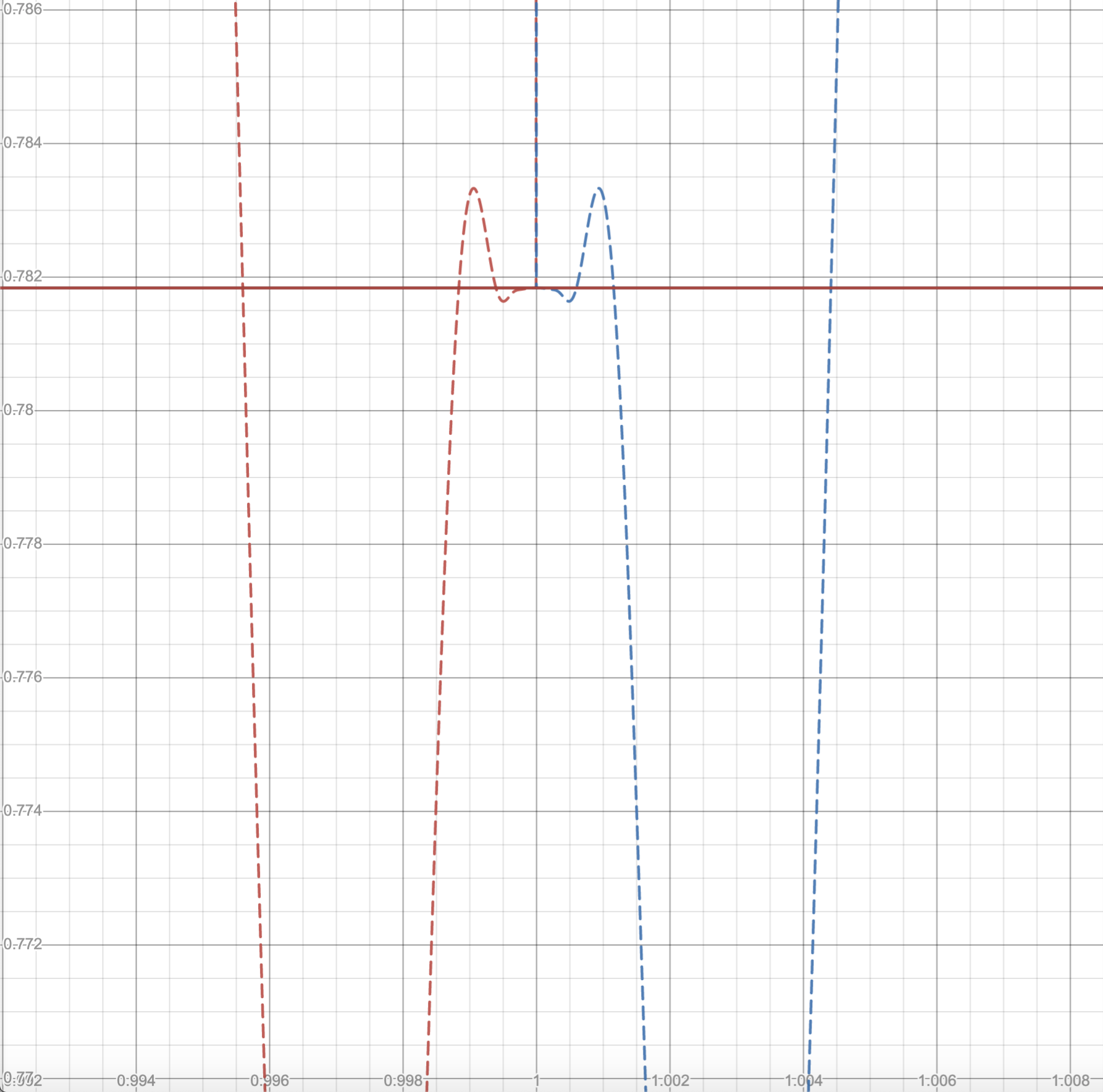
The red line is the value assigned by CaeseroCesàro summation for $x=1$. (Here is the link to the desmos graph if you would like to test out different angles: https://www.desmos.com/calculator/fkjjctmuqf )
Similar arguments give that the real part is equal to $\frac{1}{2}$ when it converges. Numerical testing also seems to suggest that all orders of the derivatives are also equal for $f(x)$ and $g(x)$ at rational multiples of $\pi$.
In general, its seems to be true that the analytical continuation of $\sum_{n=0}^\infty f(n)x^{g(n)}$ is $-\sum_{n=1}^\infty f(-n)x^{g(-n)}$, where I think $f(n)$ must be analytic for all n, and for $g(n)$, the highest power of $n^k$ must be odd (I'm less sure if this restriction is right).
Is the formula valid, for instance, for $\sum_{n=0}^\infty x^{n^3}$, and in general? I'm unsure if I can apply the Identity theorem here since the two functions aren't defined on the boundary unless I regularize the sums. Any help or insight on this problem would be appreciated!
