I prove that they lie on a conic. Proving that is ellipse require checking some inequalities. I am not entirely sure that it is always so.
We start with
Lemma. Assume that on the picture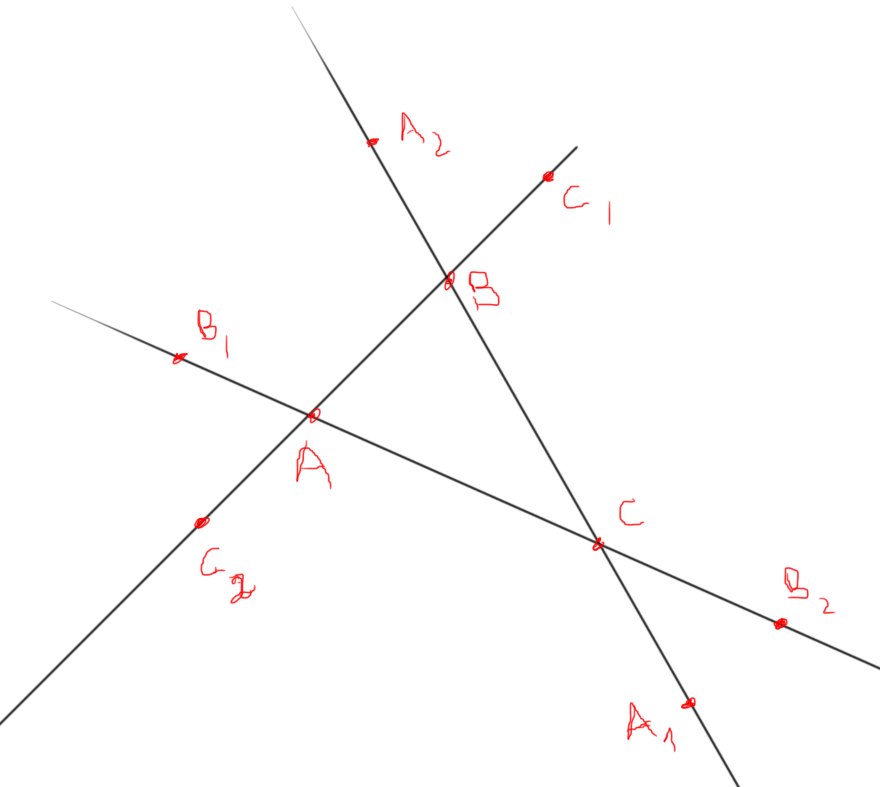 
$$\frac{AB_1\cdot AB_2}{AC_1\cdot AC_2}\cdot \frac{CA_1\cdot CA_2}{CB_1\cdot CB_2}\cdot
\frac{BC_1\cdot BC_2}{BA_1\cdot BA_2}=1.\quad\quad(\heartsuit)$$ Then $A_1,A_2,B_1,B_2,C_1,C_2$ lie on a conic.
Proof. Apply inverse Pascal theorem to the hexagon $C_2C_1A_2A_1B_2B_1$. We should check the points $A_0=A_1A_2\cap B_1C_2$,$B_0=B_1B_2\cap C_1A_2$, $C_0=C_1C_2\cap A_1B_2$ are collinear. By Menelaus we have $A_0B:A_0C=(C_2B:C_2A)\cdot(B_1A:B_1C)$. Applying three such relations and (inverse) Menelaus we see that $A_0,B_0,C_0$ are collinear if and only if $(\heartsuit)$ holds.
On your picture six segments cancel out as $NE=NM$ etc. For segments like $ND$ we denote by $S$ the double area of triangle $AFD$ (or $CFE$, or $BED$ --- three areas are equal due to your assumptions $\frac{AB}{DA}=\frac{BC}{EB}=\frac{AC}{FC}$) write $ND=AH+ED=AH+S/FD=(AH\cdot FD+S)/ED$. And remaining terms in $(\heartsuit)$ also cancel out.