This question is somewhat dual to my previously stated question about Maximum area of the intersection of a parallelogram and a triangle, where the triangle and parallelogram each is assumed to be of unit area.
Now I ask:
Question. Suppose a parallelogram is of area 1 (say, the unit square if you prefer) and a triangle is of area $1$ as well, then what is the minimum area of the convex hull of the union of the parallelogram and the triangle?
It is obvious that the minimum exists and is greater than $1$. The best I can do is $\sqrt{2}$ $-$ is this the minimum?
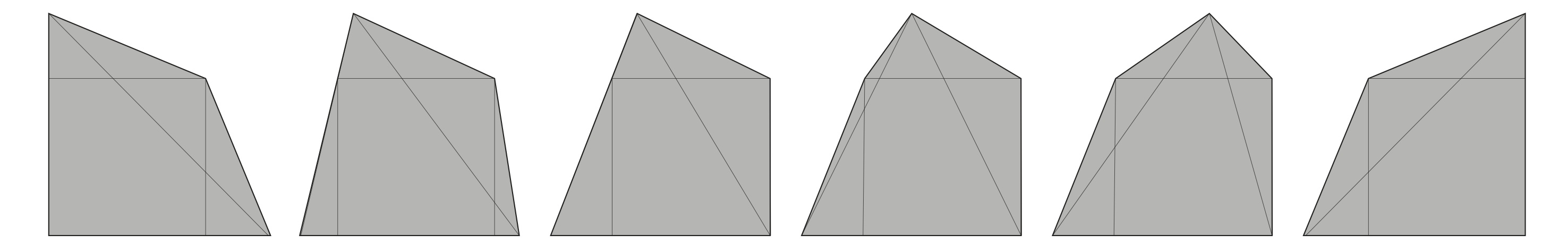
My example is: the unit square and the right triangle with legs of length $\sqrt2$ each, the triangle's right angle coinciding with the square's corner. (Added by Joe's request.)
In fact, there is a continuous cyclic family of examples, each producing the same area of the convex hull:
An analogous question can be asked in three dimensions, replacing parallelogram with parallelepiped (or cube, if you prefer) and triangle with simplex or affine pyramidpyramid with a parallelogram base, each of unit volume.